11类RD Sharma解决方案–第3章功能–练习3.2

问题1.如果f(x)= x ² -3x + 4，则找到满足方程f(x)= f(2x + 1)的x值。

解决方案：

We have, 

f(x)=x²-3x+4 

Now,

f(2x+1)=(2x+1)²-3(2x+1)+4

f(2x+1)=4x²+1+4x-6x-3+4

f(2x+1)=4x²-2x+2

It is given that 

f(x)=f(2x+1)

x²-3x+4=4x²-2x+2

0=4x²-x²-2x+3x+2-4

3x²+x-2=0

3x²+3x-2x-2=0

3x(x+1)-2(x+1)=0

(x+1)(3x-2)=0

x+1=0 or 3x-2=0

x=-1 or x=2/3

Therefore, the value of x are -1 and 2/3.

问题2.如果f(x)=(xa) ² (xb) ² ，则找到f(a + b)。

解决方案：

We have, 

f(x)=(x-a)²(x-b)²

Now, let us find f(a+b)

f(a+b)=(a+b-a)²(a+b-b)²

f(a+b)=b²a²

Therefore, f(a+b)=(ba)²

问题3.如果y = f(x)=(ax-b)/(bx-a)，请证明x = f(y)。

解决方案：

Given,

y=f(x)=(ax-b)/(bx-a)

As we know, f(y)=(ay-b)/(by-a)

Let us prove that x=f(y).

We have,

y=(ax-b)/(bx-a)

By cross multiplying,

y(bx-a)=ax-b

bxy-ay=ax-b

bxy-ax=ay-b

x(by-a)=ay-b

x=(ay-b)/(by-a)

Therefore, x=f(y)

Hence proved.

问题4.如果f(x)= 1 /(1 – x)，则表明f [f {f(x)}] = x。

解决方案：

Given:

f (x) = 1 / (1 – x)

Let us prove that f [f {f (x)}] = x.

Firstly, let us solve for f {f (x)}.

f {f (x)} = f {1/(1 – x)}

= 1 / 1 – (1/(1 – x))

= 1 / [(1 – x – 1)/(1 – x)]

= 1 / (-x/(1 – x))

= (1 – x) / -x

= (x – 1) / x

∴ f {f (x)} = (x – 1) / x

Now, we shall solve for f [f {f (x)}]

f [f {f (x)}] = f [(x-1)/x]

= 1 / [1 – (x-1)/x]

= 1 / [(x – (x-1))/x]

= 1 / [(x – x + 1)/x]

= 1 / (1/x)

∴ f [f {f (x)}] = x

Hence proved.

问题5.如果f(x)=(x + 1)/(x – 1)，请证明f [f(x)] = x。

解决方案：

Given:

f (x) = (x + 1) / (x – 1)

We have to prove that f [f (x)] = x.

f [f (x)] = f [(x+1)/(x-1)]

= [(x+1)/(x-1) + 1] / [(x+1)/(x-1) – 1]

= [[(x+1) + (x-1)]/(x-1)] / [[(x+1) – (x-1)]/(x-1)]

= [(x+1) + (x-1)] / [(x+1) – (x-1)]

= (x+1+x-1)/(x+1-x+1)

= 2x/2

= x

∴ f [f (x)] = x

Hence proved.

问题6。

$f(x) = \begin{cases} x^2,\text{when } x<0\\ x, \text{when }0\leq x<1\\ \frac{1}{x},\text{when }x\geq1 \end{cases}$

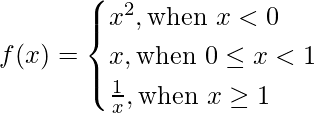
找：

(i)f(1/2)

(ii)f(-2)

(iii)f(1)

(iv)f(√3)

(v)f(√-3)

解决方案：

(i) f (1/2)

When, 0 ≤ x ≤ 1, f(x) = x

∴ f (1/2) = 1/2

(ii) f (-2)

When, x < 0, f(x) = x²

f (–2) = (–2)²

= 4

∴ f (–2) = 4

(iii) f (1)

When, x ≥ 1, f (x) = 1/x  

f (1) = 1/1

∴ f(1) = 1

(iv) f (√3)

We have √3 = 1.732 > 1  

When, x ≥ 1, f (x) = 1/x  

∴ f (√3) = 1/√3

(v) f (√-3)

We know √-3 is not a real number and the function f(x) is defined only when x ∈ R.

∴ f (√-3) does not exist.

问题7.如果f(x)= x ^3- (1 / x ³ )，则表明f(x)+ f(1 / x)= 0。

解决方案：

We have,

f(x)=x³-(1/x)³              —(i)

Now,

f(1/x)=(1/x)³-(1/(1/x)³)

f(1/x)=(1/x)³-x³        —(ii)

Adding equation (i) and (ii), we get 

f(x)+f(1/x) = (x³-1/x³)+(1/x³-x³) 

f(x)+f(1/x)=x³-x³+1/x³-1/x³

f(x)+f(1/x)=0    

Hence, proved.

问题8.如果f(x)= 2x /(1 + x ² )，则表明f(tanθ )= sin2θ 。

解决方案。

We have,

f(x)=2x/(1+x²)

Now,

f(tan θ)=2(tan θ)/(1+tan² θ)

f(tan θ)=sin 2θ    (Because, sin 2θ = 2(tan θ)/(1+tan²θ))

Hence, proved.

问题9.如果f(x)=(x-1)/(x + 1)，则表明

i)f(1 / x)=-f(x)

ii)f(1 /(-x))=-1 / f(x)

解决方案。

i) We have, 

f(x)=(x-1)/(x+1)

Now, 

f(1/x)=((1/x)-1)/((1/x)+1)

f(1/x)=((1-x)/x)/((1+x)/x)

f(1/x)=(1-x)/(1+x)=f(-x)

Hence, proved.

ii) We have, 

f(x)=(x-1)/(x+1)

Now, 

f(1/(-x))= ((1/(-x))-1)/((1/(-x))+1)

f(1/(-x))=((1+x)/(-x))/((1-x)/(-x))

f(1/(-x))=(1+x)/(1-x)

f(1/(-x))=(-1)/((x-1)/(x+1))

f(1/(-x))=-1/f(x)

Hence, proved.

问题10.如果f(x)=(ax ⁿ ) ^{1 / n} ，a> 0且n∈N，则证明对所有x都f(f(x))= x。

解决方案。

We have, 

$f(x)=(a-x^n)^\frac{1}{n}, a>0\\ \newline \\ Now, \\ f(f(x))=f(a-x^n)^\frac{1}{n}\\ f(f(x))=[a-\{(a-x^n)^\frac{1}{n}\}^n]^\frac{1}{n} \\ f(f(x))=[a-(a-x^n)]^\frac{1}{n}\\ f(f(x))=[a-a+x^n]^\frac{1}{n}\\ f(f(x))=(x^n)^\frac{1}{n}\\ f(f(x))=x\\ Hence, proved$

问题11。如果对于非零x，af(x)+ bf(1 / x)= 1 / x – 5，其中a ≠ b，则找到f(x)。

解决方案。

We have,

$af(x)+bf(\frac{1}{x})=\frac{1}{x}-5$      —(i)

$af(\frac{1}{x})+bf(x)=\frac{1}{\frac{1}{x}}-5\\ af(\frac{1}{x})+bf(x)=x-5$

$af(\frac{1}{x})+bf(x)=x-5$                —(ii)                         

Adding equation (i) and (ii), we get 

$af(x)+bf(x)+bf(\frac{1}{x})+af(\frac{1}{x})=\frac{1}{x}-5+x-5\\ (a+b)f(x)+f(\frac{1}{x})(a+b)=\frac{1}{x}+x-10$        

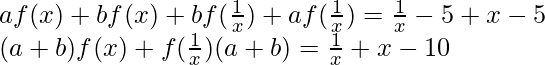
$f(x)+f(\frac{1}{x})=\frac{1}{a+b}[\frac{1}{x}+x-10]$                   —(iii)

Subtracting equation (ii) from equation (i)

$af(x)-bf(x )+bf(\frac{1}{x})-af(\frac{1}{x})=\frac{1}{x}-5-x+5 \\ (a-b)f(x)-f(\frac{1}{x})(a-b)=\frac{1}{x}-x \\ f(x)-f(\frac{1}{x})=\frac{1}{a-b}[\frac{1}{x}-x]$  —(iv)

Adding equations(iii) and (iv), we get 

$2f(x)=\frac{1}{a+b}[\frac{1}{x}+x-10]+\frac{1}{a-b}[\frac{1}{x}-x]\\ 2f(x)=\frac{(a-b)[\frac{1}{x}+x-10]+(a+b)[\frac{1}{x}-x]}{(a+b)(a-b)} \\ 2f(x)=\frac{\frac{a}{x}+ax-10a-\frac{b}{x}-bx+10b+\frac{a}{x}-ax+\frac{b}{x}-bx}{a^2-b^2} \\ 2f(x)=\frac{\frac{2a}{x}-10a+10b-2bx}{a^2-b^2} \\ f(x)=\frac{1}{a^2-b^2}\times\frac{1}{2}[\frac{2a}{x}-10a+10b-2bx]\\ f(x)=\frac{1}{a^2-b^2}[\frac{a}{x}-5a+5b-bx]$

Therefore, 

$f(x)=\frac{1}{a^2-b^2}[\frac{a}{x}-5a+5b-bx]$