问题14.如果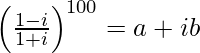 ,找到(a,b)。
,找到(a,b)。
解决方案:
We have,
=>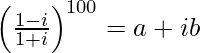
=>![Rendered by QuickLaTeX.com \left[\frac{(1-i)^2}{(1+i)(1-i)}\right]^{100}=a+ib](https://mangdo-1254073825.cos.ap-chengdu.myqcloud.com//front_eng_imgs/geeksforgeeks2021/Class%2011%20RD%20Sharma%20Solutions%20%E2%80%93%20Chapter%2013%20Complex%20Numbers%20%E2%80%93%20Exercise%2013.2%20%7C%20Set%202_2.jpg)
=>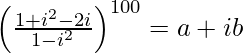
=>![]()
=> (−i)100 = a + ib
=> a + ib = 1
On comparing real and imaginary parts on both sides, we get,
=> (a, b) = (1, 0)
问题15。如果a = cosθ+ i sinθ,则求出值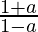 。
。
解决方案:
Given a = cos θ + i sin θ, we get,
![]() =
=![]()
=![]()
=![]()
=![]()
=![]()
=![]()
=![]()
=![]()
=![]()
=![]()
Therefore, the value of![]() is
is![]() .
.
问题16:评估以下内容:
(i)当x =(3-5i)/ 2时2x 3 + 2x 2 − 7x + 72
解决方案:
We have, x = (3−5i)/2
=> 2x = 3 − 5i
=> 2x − 3 = −5i
=> (2x − 3)2 = 25i2
=> 4x2 + 9 − 12x = −25
=> 4x2 − 12x + 34 = 0
=> 2x2 − 6x + 17 = 0
Now, 2x3 + 2x2 − 7x + 72 = x (2x2 − 6x + 17) + 6x2 − 17x + 2x2 − 7x + 72
= x (0) + 8x2 − 24x + 72
= 4 (2x2 − 6x + 17) + 4
= 4 (0) + 4
= 4
Therefore, the value of 2x3 + 2x2 − 7x + 72 is 4.
(ii)当x = 3 + 2i时, x 4 − 4x 3 + 4x 2 + 8x +44
解决方案:
We have, x = 3 + 2i
=> x − 3 = 2i
=> (x − 3)2 = (2i)2
=> x2 + 9 − 6x = 4i2
=> x2 − 6x + 9 + 4 = 0
=> x2 − 6x + 13 = 0
Now, x4 − 4x3 + 4x2 + 8x + 44 = x2 (x2 − 6x + 13) + 6x3 − 13x2 − 4x3 + 4x2 + 8x + 44
= 2x3 − 9x2 + 8x + 44
= 2x (x2 − 6x + 13) + 12x2 − 26x − 9x2 + 8x + 44
= 3x2 − 18x + 44
= 3 (x2 − 6x + 13) + 5
= 5
Therefore, the value of x4 − 4x3 + 4x2 + 8x + 44 is 5.
(iii)x 4 + 4x 3 + 6x 2 + 4x + 9(当x = −1 +i√2时)
解决方案:
We have, x = −1 + i√2
=> x + 1 = i√2
=> (x + 1)2 = 2i2
=> x2 + 1 + 2x = −2
=> x2 + 2x + 3 = 0
Now, x4 + 4x3 + 6x2 + 4x + 9 = x2 (x2 + 2x + 3) − 2x3 − 3x2 + 4x3 + 6x2 + 4x + 9
= 2x3 + 3x2 + 4x + 9
= 2x (x2 + 2x + 3) − 4x2 − 6x + 3x2 + 4x + 9
= − x2 − 2x + 9
= − (x2 + 2x + 3) + 3 + 9
= 3 + 9
= 12
Therefore, the value of x4 + 4x3 + 6x2 + 4x + 9 is 12.
(iv)x 6 + x 4 + x 2 +1,当x =(1 + i)/√2时
解决方案:
We have, x = (1+i)/√2
=> √2x = 1 + i
=> 2x2 = 1 + i2 + 2i
=> 2x2 = 2i
=> 4x4 = 4i2
=> x4 = −1
=> x4 + 1 = 0
Now, x6 + x4 + x2 + 1 = (x6 + x2) + (x4 +1)
= x6 + x2
= x2 (x4 + 1)
= 0
Therefore, the value of x6 + x4 + x2 + 1 is 0.
(v)当x = −2 −√3i时为2x 4 + 5x 3 + 7x 2 − x + 41
解决方案:
We have, x = −2 − √3i
x2 = (−2 − √3i)2 = 4 + 4√3i + 3i2 = 1 + 4√3i
x3 = (1 + 4√3i) (−2 − √3i) = −2 − √3i − 8√3i −12i2 = 10 − 9√3i
x4 = (1 + 4√3i)2 = 1 + 8√3i + 48i2 = −47 + 8√3i
Now, 2x4 + 5x3 + 7x2 − x + 41 becomes,
= 2(−47 + 8√3i) + 5(10 − 9√3i) + 7(1 + 4√3i) − (−2 − √3i) + 41
= −94 + 16√3i + 50 − 45√3i + 7 + 28√3i + 2 + √3i + 41
= 6
Therefore, the value of 2x4 + 5x3 + 7x2 − x + 41 is 6.
问题17.对于正整数n,找到(1-i) n (1-1 / i) n的值。
解决方案:
We have,
(1−i)n (1−1/i)n = (1−i)n![]()
=![]()
=![]()
=![]()
= 2n
Therefore, the value of (1−i)n (1−1/i)n is 2n.
问题18.如果(1 + i)z =(1-i) 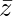 ,则表明z = −i
,则表明z = −i 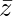 。
。
解决方案:
We have,
=> (1+i)z = (1−i)![]()
=> z =![]()
=> z =![]()
=> z =![]()
=> z =![]()
=> z = −i![]()
Hence proved.
问题19:解方程组:Re(z 2 )= 0,| z | = 2。
解决方案:
Let z = x + iy.
Now z2 = (x + iy)2
= x2 + i2y2 + 2xyi
= x2 − y2 + 2xyi
We have, Re(z2) = 0
=> x2 − y2 = 0 . . . . (1)
Also, it is given, |z| = 2.
=>![]() = 2
= 2
=> x2 + y2 = 4 . . . . (2)
Solving (1) and (2), we get, x = ±√2 and y = ±√2.
Therefore, x + iy = ±√2 ± √2i .
问题20.如果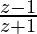 是纯虚数(z≠−1),请找到| z |的值。
是纯虚数(z≠−1),请找到| z |的值。
解决方案:
Let z = x + iy
We have,![]()
=![]()
=![]()
=![]()
=![]()
=![]()
As the complex number is purely imaginary, therefore,
=> Re(z) = 0
=>![]() = 0
= 0
=> x2 + y2 = 1
=>![]() = 1
= 1
=> |z| = 1
Therefore, the value of |z| is 1.
问题21.如果z 1是非-1的复数,则| z 1 | = 1和z 2 = 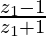 ,则表明z 2的实部为零。
,则表明z 2的实部为零。
解决方案:
Given |z| = 1
=> |z|2 = 1
=> x2 + y2 = 1 . . . . (1)
Let z1 = x + iy and z2 = a + ib.
According to the question, we have,
=> z2 =![]()
=> a + ib =![]()
=> a + ib =![]()
=> a + ib =![]()
=> a + ib =![]()
Using (1) we get,
=> a + ib =![]()
=> a + ib =![]()
On comparing the real and imaginary parts on both sides, we get a = 0.
Therefore, the real parts of z2 is 0. Hence proved.
问题22:如果| z + 1 | = z + 2(1 + i),找到z。
解决方案:
Let z = x + iy. According to the question, we have,
=> |x + iy + 1| = x + iy + 2(1 + i)
=>![]() = (x + 2) + i(y + 2)
= (x + 2) + i(y + 2)
On comparing the real and imaginary parts, we get
=> y + 2 = 0
=> y = −2
And also,
=> x + 2 =![]()
=> (x + 2)2 = (x+1)2 + y2
=> x2 + 4 + 4x = x2 + 2x + 1+ y2
=> 2x = y2 − 3
=> 2x = 4 − 3
=> 2x = 1
=> x = 1/2
Therefore, z = x + iy = 1/2 −2i.
问题23.解方程:| z | = z + 1 + 2i。
解决方案:
Let z = x + iy. According to the question, we have,
=> |z| = z + 1 + 2i
=> |x + iy| = x + iy + 1 + 2i
=>![]() = (x + 1) + (y + 2)i
= (x + 1) + (y + 2)i
=> x2 + y2 = (x+1)2 + (y+2)2i2 + 2 (x+1) (y+2)i
=> x2 + y2 = x2+1 + 2x − y2 − 1 + 2y + 2 (x+1) (y+2)i
=> 2y2 − 2x + 4y + 4 = 2i (x+1) (y+2)
=> y2 − x + 2y + 2 = i (x+1) (y+2)
On comparing both sides, we get,
=> (x+1) (y+2) = 0
=> x = −1 and y = −2
Also, y2 − x + 2y + 2 = 0
Taking x = −1, we get y2 − (−1) + 2y + 2 = 0
=> y2 + 2y + 3 = 0, which doesn’t have a solution as the roots are imaginary.
Taking y = −2, (4 − x −4 + 2) = 0
=> x = 2
Therefore, z = x + iy = 2 − 2i.
问题24.(1 + i) 2n =(1-i) 2n的最小正整数n是多少?
解决方案:
We are given,
=> (1+i)2n = (1−i)2n
=>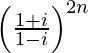 = 1
= 1
=>![Rendered by QuickLaTeX.com \left[\frac{(1+i)^2}{(1-i)(1+i)}\right]^{2n}](https://mangdo-1254073825.cos.ap-chengdu.myqcloud.com//front_eng_imgs/geeksforgeeks2021/Class%2011%20RD%20Sharma%20Solutions%20%E2%80%93%20Chapter%2013%20Complex%20Numbers%20%E2%80%93%20Exercise%2013.2%20%7C%20Set%202_53.jpg) = 1
= 1
=>![Rendered by QuickLaTeX.com \left[\frac{1+i^2+2i}{1-i^2}\right]^{2n}](https://mangdo-1254073825.cos.ap-chengdu.myqcloud.com//front_eng_imgs/geeksforgeeks2021/Class%2011%20RD%20Sharma%20Solutions%20%E2%80%93%20Chapter%2013%20Complex%20Numbers%20%E2%80%93%20Exercise%2013.2%20%7C%20Set%202_54.jpg) = 1
= 1
=>![]() = 1
= 1
=> i2n = 1
=> i2n = i4
=> 2n = 4
=> n = 2
Therefore, the smallest positive integer n for which (1+i)2n = (1−i)2n is 2.
问题25.如果z 1 ,z 2 ,z 3是复数,使得| z 1 | = | z 2 | = | z 3 | = 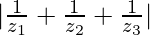 = 1,然后找到| z 1 + z 2 + z 3 |的值。
= 1,然后找到| z 1 + z 2 + z 3 |的值。
解决方案:
We are given,
|z1| = |z2| = |z3| =![]() = 1
= 1
Now, |z1 + z2 + z3| =![]()
=![]()
=![]()
= 1
Therefore, the value of |z1 + z2 + z3| is 1.
问题26.找出z 2 + | z |的解数2 = 0。
解决方案:
Let z = x + iy. We have,
=> z2 + |z|2 = 0
=> (x + iy)2 + |x + iy|2 = 0
=> x2 + i2y2 + 2xyi + x2 + y2 = 0
=> x2 − y2 + 2xyi + x2 + y2 = 0
=> 2x2 + 2xyi = 0
On comparing the real and imaginary parts on both sides, we get
=> 2x2 = 0 and 2xy = 0
=> x = 0 and y ∈ R
Therefore, z = 0 + iy, where y ∈ R.