在求解和找到三角形的面积时,应事先提供某些参数,例如,三角形的高度和底边必须可用,或者在等边三角形的情况下,应给出边的长度。
三角形的面积通常由下式给出:
![]()
底部和高度是从给定的三角形开始测量的。但是有时可能会发生我们没有该信息的情况。假设我们只有边的长度信息。高度未知,那么面积将如何计算呢?
这个问题的答案是苍鹭的公式。
Heron was born in Egypt in about 10AD. He was a great mathematician, he worked in applied mathematics. His great deal of work revolved around squares, rectangles. This formula is also called “Hero’s Formula”.
苍鹭的配方
苍鹭的配方也被称为英雄的配方,以埃及的一位非常著名的工程师的名字命名。他以著名的“亚历山大英雄”而闻名,他的著名作品包括苍鹭的配方,自动贩卖机等。公式是在求解任何三角形的面积之前不需要任何角度或距离。
假设我们有一个三角形ABC,其边长为“ a”,“ b”和“ c”。
然后是苍鹭给定的那个三角形的面积,
三角形ABC的面积![]()
其中a,b和c是边的长度,而s =半周长(周长的一半)
![]()
该公式使我们能够计算未给出高度长度的三角形的面积。
苍鹭公式在三角形寻找区域中的应用
如果给出了三角形的边长,则可以使用“苍鹭公式”来查找不同类型的三角形的区域,
问题:如果三角形的边为3、5、4,则找出三角形的面积。
解决方案:
Area of this triangle can be calculated by Heron’s formula studied above,
Let’s calculate semi-perimeter first,

Plugging the values of s, a, b and c in the formula. Let’s calculate the area.
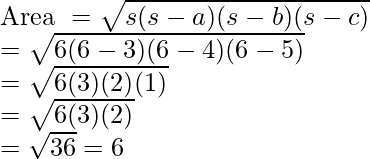
Thus, the area of the triangle is 6 square units.
苍鹭公式在四边形求面积中的应用
我们知道如何计算矩形,正方形和梯形等标准四边形的面积。但是有时候我们的四边形可能没有任何一种形式。

上面给出的四边形不属于这些类别,因此无法使用我们的标准公式。苍鹭的公式在这种情况下对我们有帮助。我们可以将上图中的任意两个相反的顶点连接起来,并可以形成两个三角形。然后可以计算出面积。让我们来看一些有关此的示例。
问题:使用苍鹭公式找出给定菱形的区域。
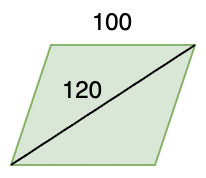
解决方案:
There are two triangles here, both of them are similar.
The length of sides of both triangles are 100, 100 and 120.
Thus, semi perimeter for both the triangles is,
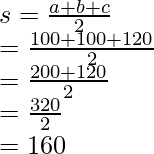
Now, calculating the area of the triangle

Thus, area of a single triangle is 4800. Since, there are two equal triangles. So, the total area is 9600 sq units.
让我们看一些关于这些概念的样本问题,
基于苍鹭公式的示例问题
问题1:有一个三角形的混凝土空间需要平铺。平铺的成本为每平方单位20卢比。查找平铺该区域的总成本。

回答:
The length of given sides are 10, 10 and 10.
The semi-perimeter “s” = 15
We know the area of the triangle using Heron’s formula.
![]()
Here, s = 15, a = b = c = 10.

The area of the triangle is 25√3
So, the cost of tiling the area = 25√3 x 20
= 500√3
= 500 (1.73)
= 866 (approx)
Thus, cost of tiling the area is Rs. 866.
问题2:三角形的边长比例为3:5:4,周长为96m。找到三角形的面积。
解决方案:
The sides are in ratio of 3:5:4. Suppose the length of the sides is 3x,5x and 4x.
3x + 5x + 4x = 96
= 12x = 96
= x = 8.
Thus, the sides are 24, 40, 32. Now let’s calculate “s” and plug in the values in Heron’s formula.
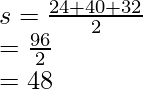

问题3:假设三角形的边分别为3y,4y和5y。找出三角形面积的表达式。
回答:
Let’s find out the perimeter and semi perimeter so that we can plug in the values in Heron’s formula.
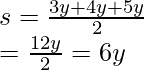
Plugging in the values in Heron’s Formula.
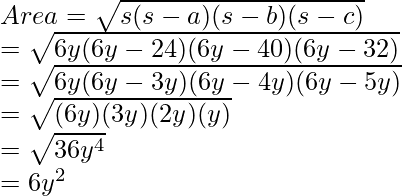
问题4:找出以下四边形的面积:

解决方案:
The quadrilateral ABCD can be divided into two triangles whose area we can compute if we join A and C.
Now we have triangles ACD and ABC. Out of which ACD is a right-angled triangle.
AC2 = AD2 + DC2
⇒ AC2 = 92 + 402
⇒ AC2 = 81 + 1600
⇒ AC2 = 1681
⇒ AC = 41
Area of triangle ADC = 
Area of triangle ABC will be calculated using Heron’s Formula. The sides of the triangle are 28,15 and 41.
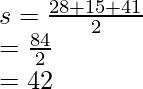
Area of triangle ABC = 
Area of quadrilateral = Area of triangle ADC + Area of triangle ABC
= 180 + 126
= 306 sq units.
问题5:假设三角形的边分别为2y,2y + 2和4y – 2,并且其面积由y√10给出。找出y的值。
解决方案:
Let’s calculate “s”
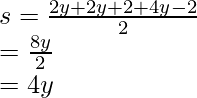
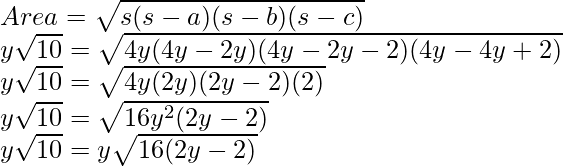
Comparing both sides of the equation,
