9类RD Sharma解决方案–第12章苍鹭的公式-练习12.1

问题1.找到一个三角形的面积，该三角形的边分别为150厘米，120厘米和200厘米。

解决方案：

By Heron’s formula, we have,

Area of triangle = $\sqrt[]{s(s-a)(s-b)(s-c)}$

Semi-perimeter, s = (a + b + c)/2

where a, b and c are sides of triangle

We have,

a = 150 cm

b = 120 cm

c = 200 cm

Step 1: Computing s

s = (a+b+c)/2

s = (150+200+120)/2

s = 235 cm

Step 2: Computing area of a triangle

 $Area= \sqrt[]{235(235-120)(235-150)(235-200) } \\ =\sqrt[]{235(35)(115)(85) } \\=\sqrt[]{8093375}$

= 8966.56

Area of triangle is 8966.56 sq. cm.

问题2。找到三角形的边长分别为9 cm，12 cm和15 cm的区域。

解决方案：

By Heron’s formula, we have,

Area of triangle = \sqrt[]{s(s-a)(s-b)(s-c)}

Semi-perimeter, s = (a + b + c)/2

where a, b and c are sides of triangle

We have, 

a = 9 cm

b = 12 cm

c = 15 cm

Step 1: Computing s

s = (a+b+c)/2

s = (9 + 12 + 15)/2

s = 18 cm

Step 2: Computing area of a triangle

$Area= \sqrt[]{18(18-9)(18-12)(18-15) } \\ =\sqrt[]{18 * 9 * 6 * 3} \\=\sqrt[]{2916}$

= 54

Area of triangle is 54 sq. cm.

问题3.找到三角形的面积，该三角形的两个边分别为18 cm和10 cm，周长为42 cm。

解决方案：

We have,

a = 18 cm, b = 10 cm, and perimeter = 42 cm

Let us assume c to be the third side of the triangle.

Step 1: Computing third side of the triangle, that is c,

We know, perimeter = 2s,

2s = 42

s = 21

Also,

s = (a+b+c)/2

Substituting s, we get

21 = (18+10+c)/2

42 = 28 + c

c = 14 cm

Step 2: Computing area of triangle, 

$Area= \sqrt[]{21(21-18)(21-10)(21-14) } \\ =\sqrt[]{21 * 3 * 11 * 7} \\=21\sqrt[]{11}$

问题4.在一个三角形的ABC中，AB = 15厘米，BC = 13厘米，AC = 14厘米。求出三角形ABC的面积，并求出其在AC上的高度。

解决方案：

By Heron’s formula, we have,

Area of triangle =\sqrt[]{s(s-a)(s-b)(s-c)}

Semi-perimeter, s = (a + b + c)/2

where a, b and c are sides of triangle

We have,

a = 150 cm

b = 120 cm

c = 200 cm

Step 1: Computing s

s = (a+b+c)/2

s = (15+13+14)/2

s = 21 cm

Step 2: Computing area of a triangle

$Area= \sqrt[]{21(21-13)(21-14)(21-15) } \\ =\sqrt[]{21 * 8 * 7 * 6} \\=\sqrt[]{7056}$

= 84

Area = 84 cm²

Let us assume, BE is a perpendicular on AC

We know, area of triangle = ½ x Base x Height

=> ½ × BE × AC = 84

BE = 12cm

Therefore, the length of altitude is 12 cm.

问题5：一个三角形场的周长为540 m，其边长比为25:17:12。找到三角形的面积。

解决方案：

Let the sides of a given triangle be a = 25x, b = 17x, c = 12x respectively,

We have, Perimeter of triangle = 540 cm

Also, Perimeter = 2s

2s = a + b + c

=> a + b + c = 540 cm

=> 25x + 17x + 12x = 540 cm

=> 54x = 540 cm

=> x = 10 cm

So, the sides of a triangle are

a = 250 cm

b = 170 cm

c = 120 cm

Also, semi perimeter, s = (a+b+c)/2

= 540/2

= 270

s = 270 cm

And, 

$Area= \sqrt[]{270(270-250)(270-170)(270-120) } \\ =\sqrt[]{270 * 20 * 100 * 150} \\=\sqrt[]{81000000}$

= 9000

Hence, the area of the triangle is 9000 sq. cm.

问题6.三角形场的周长为300 m，边长为3：5：7。找到三角形的面积。

解决方案：

Let the sides of a given triangle be a = 3x, b = 5x, c = 7x respectively,

We have, Perimeter of triangle = 300 m

Also, Perimeter = 2s

2s = a + b + c

=> a + b + c = 300 m

=> 3x + 5x + 7x = 300 m

=> 15x = 300 m

=> x = 20 cm

So, the sides of a triangle are

a = 60 m

b = 100 m

c = 140 m

Also, semi perimeter, s = (a+b+c)/2

= 300/2

= 150

s = 150 m

And,

$Area= \sqrt[]{150(150-60)(150-100)(150-140) } \\ =\sqrt[]{150 * 90 * 50 * 10} \\=1500\sqrt[]{3}$

Hence, the area of the triangle is 1500\sqrt[]{3} sq. cm.

问题7.三角形场的周长为240dm。如果它的两个侧面分别为78dm和50 dm，请从相反的顶点找到侧面50dm的垂直线的长度。

解决方案：

Semi-perimeter, s = (a + b + c)/2

where a, b and c are sides of triangle

Perimeter = 2s

2s = 240dm

s = 120dm

Let the third side be x. 

Now, 

120 = (78 + 50 + x)/2

x = 112 dm

Computing area of triangle, we have, 

$Area= \sqrt[]{120(120-112)(120-50)(120-78) } \\ =\sqrt[]{(3 * 8 * 7 * 10)^2} \\=\sqrt[]{3 * 8 * 7 * 10} \\={3 * 8 * 7 * 10} sq. dm. \\={1680} sq. dm.$

Let us assume the length of altitude of length 50dm be a.

We know, area of triangle = ½ x Base x Height

=> ½ × a × 50 = 1680

BE = 67.2dm

Therefore, the length of altitude is 67.2 dm.

问题8.三角形的边长分别为35cm，54cm和61cm。找到它的区域。找到最小的高度。

解决方案：

Semi-perimeter, s = (a + b + c)/2

where a, b and c are sides of triangle

s = (34+54+61)/2

=75 cm

Computing area of triangle, we have, 

$Area= \sqrt[]{75(75-35)(75-54)(75-61) } \\ =\sqrt[]{(75 * 40 * 21 * 14)^2} \\=\sqrt[]{(5 * 3 * 7 * 2)^2 * 2 * 5} \\={5 * 3 * 7 * 2}\sqrt{10} sq. cm. \\={420}\sqrt{5} sq. cm.$

Let us assume the length of altitude of length 50dm be a.

We know, area of triangle = ½ x Base x Height

For different measurements, the area remains constant. 

The smallest altitude always lies on the longest side.

$½ × a × 61 = 420 \sqrt{5}$

=> a = 30.79 cm

Therefore, the length of altitude  = 30.79 cm

问题9：一个三角形场的周长为144厘米，其边的比例为3：4：5。找到与最长边相对应的三角形的面积和高度。

解决方案：

Let the sides of a given triangle be a = 3x, b = 4x, c = 5x respectively,

We have, Perimeter of triangle = 144 cm

Also, Perimeter = 2s

2s = a + b + c

=> a + b + c = 144 cm

=> 3x + 4x + 5x = 144 cm

=> 12x = 144cm

=> x = 12 cm

So, the sides of a triangle are

a = 36 cm

b = 48 m

c = 60 m

Also, semi perimeter, s = (a+b+c)/2

= 144/2

= 72

s = 72 cm

And,

$Area= \sqrt[]{72(72-60)(72-48)(72-36) } \\ =\sqrt[]{72 * 12 * 24 * 36}$\\=1500\sqrt[]{3}

a = 9.6 cm

问题10.等腰三角形的周长是42厘米，其底边(3/2)乘以其等边的倍数。找出每边的长度。

解决方案：

Let the equal sides be a. 

So, base = 3/2a

Perimeter of triangle = 3/2a + a + a

7a/2 = 42 cm

a = 12 cm

Therefore, sides of the triangle are, 12 cm is each of the equal sides

and 3/2a = 18 cm = base

Semi perimeter = 42/2 cm = 21 cm

By heron’s formula, 

$Area= \sqrt[]{21(21-12)(21-18)(21-12) } \\ =\sqrt[]{21 * 3 * 9 * 9} \\=\sqrt[]{7 * 3 * 3 * 9 * 9} \\={27}\sqrt{7} sq. cm. \\=71.43 sq. cm.$

We know, area of triangle = ½ x Base x Height

Substituting th values we get,

1/2 x h x 18 = 71.43 cm

h = 7.92 cm

问题11：找到阴影区域

解决方案：

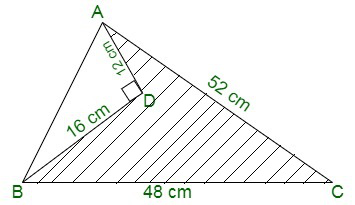
By Pythagoras theorem, 

AB² = AD² + BD²

$AB = \sqrt[]{AB^2 + BD^2} \\AB = \sqrt[]{12^2 + 16^2} \\AB = \sqrt[]{144 + 256} \\AB = \sqrt[]{400} AB = 20cm$

In triangle ABC,

Perimeter = AB + BC + AC = 20cm + 52cm + 48cm 

=120 cm

Now,

s = 120/2 = 60 cm

Area of the triangle by heron’s formula, 

$Area= \sqrt[]{60(60-20)(60-48)(60-52) } \\ =\sqrt[]{60 * 40 * 12 * 8} \\=\sqrt[]{(8 * 8 * 6 * 6 * 10 * 10)} \\=\sqrt[]{(8 * 6 * 10)^2} \\=480 sq. cm.$

Area of triangle ABD = 1/2(AD)(BD)

1/2 (12)(16) sq. cm.  = 96 sq. cm.

Shaded area = area of triangle ABC – area of triangle ABD

= 480 – 96 sq. cm.

384 sq. cm.