9类NCERT解决方案-第12章“苍鹭的公式” –练习12.2

问题1.一个呈四边形ABCD形状的公园，其C =90º，AB = 9 m，BC = 12 m，CD = 5 m，AD = 8 m。它占用多少面积?

解决方案：

Given, a quadrilateral ABCD where ∠C = 90º.

Construction: Join diagonal BD.

As we can see that, △DCB is right-angled at C

Hence, BC is the base and CD is height of △DCB, so

ar(△DCB) = $\mathbf{\frac{1}{2}}$ × Base × Height

= $\frac{1}{2}$ × 12 × 5

= 30 m²……………………………………(1)

As △DCB is right angle triangle we can calculate third side by Pythagoras theorem

BD² = CB² + CD²

BD² = 12² + 5²

BD = √(144+25)

BD = √169

BD =13 m

Now, Area of △DAB can be calculated by Heron’s Formula, where 

AB = a = 9 m

AD = b = 8 m

BD = c = 13 m 

Semi Perimeter (s) = $\mathbf{\frac{a+b+c}{2}}$

s = $\frac{9+8+13}{2}$

s = 15 m

ar(△DAB) = √s(s-a)(s-b)(s-c)

= √15(15-9)(15-8)(15-13)

= √15×(6)×(7)×(2)

= 6√35 m²……………………………………..(2)

From (1) and (2), we can conclude that,

ar(ABCD) = ar(△DCB)+ar(△DAB)

= (30 + 6√35)

= (30 + 35.5)

≈ 65.5 m² (approx.)

问题2。找到四边形ABCD的面积，其中AB = 3 cm，BC = 4 cm，CD = 4 cm，DA = 5 cm，AC = 5 cm。

解决方案：

Here, we can notice that in △ABC

AC² = AB² + BC² 

5² = 3² + 4²  

25 = 25

LHS = RHS

As this triangle is satisfying the Pythagoras theorem, Therefore, △ABC is a right angle triangle, 90° at B.

Hence, BC is the base and AB is height of △ABC. so

So, ar(△ABC) = $\mathbf{\frac{1}{2}}$ × Base × Height

= $\frac{1}{2}$ × 4 × 3

= 6 cm²……………………………….(1)

Now, Area of △DAC can be calculated by Heron’s Formula, where

AD = a = 5 cm

DC = b = 4 cm

AC = c = 5 cm

Semi Perimeter (s) = $\mathbf{\frac{a+b+c}{2}}$

s = $\frac{(5+4+5)}{2}$

s = 7 cm

ar(△DAC) = √s(s-a)(s-b)(s-c)

= √7(7-5)(7-4)(7-5)

= √7×(2)×(3)×(2)

= 2√21 cm²……………………………………..(2)

From (1) and (2), we can conclude that,

ar(ABCD) = ar(△ABC)+ar(△DAC)

= (6 + 2√21)

= (6 + 9.2(approx.))

≈ 15.2 cm² (approx.)

问题3. Radha用彩色纸对飞机进行了拍摄，如图(b)所示。查找所用纸张的总面积。

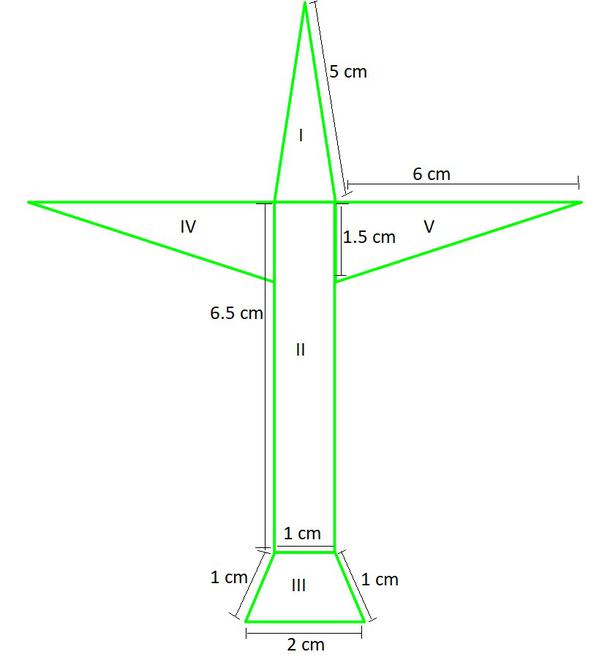
解决方案：

Total area of the paper used = (Area I + Area II + Area III + Area IV + Area V)

Area I: Triangle

Now, Area of triangle can be calculated by Heron’s Formula, where

a = 5 cm

b = 5 cm

c = 1 cm

Semi Perimeter (s) = $\mathbf{\frac{a+b+c}{2}}$

s = $\frac{(5+5+1)}{2}$

s = 5.5 cm

ar(△) = √s(s-a)(s-b)(s-c)

= √5.5(5.5-5)(5.5-5)(5.5-1)

= √5.5×(0.5)×(0.5)×(4.5)

= 0.5×0.5×3√11

= 0.75√11

 ≈ 2.5 cm² ……………………………………..(1)

Area II: Rectangle

Area of Rectangle = length×Breadth

= 1 × 6.5 = 6.5 cm²……………………………….(2)

Area III: Trapezium = Area of parallelogram EFAO + △ AFD

OD = 2cm

AD = OD-OA = 2-1 = 1 cm

Hence, △ AFD is equilateral.

PD = $\frac{1}{2}$ AD = $\frac{1}{2}$ cm

△ PFD is right angled at P, Pythagoras theorem is applicable 

1²=h² +($\frac{1}{2}$)² 

h = √(1-$\frac{1}{4}$)

h = √$\frac{3}{2}$ cm

Area III: 

= (Base × Height) + ($\frac{1}{2}$ × Base × Height)

= (1 × $\frac{√3}{2}$) + ($\frac{1}{2}$ × 1 × $\frac{√3}{2}$)

= $\frac{√3}{2}×\frac{3}{2}$

= $\mathbf{\frac{3√3}{4}}$= 1.29 cm²…………………………………(3)

Area IV and V: 2 times Triangle

ar(△) = $\mathbf{\frac{1}{2}}$ × Base × Height

= $\frac{1}{2}$ × 6 × 1.5

= 4.5 cm²

Area IV + Area V = 2×ar(△)

= 2×4.5

= 9 cm² …………………………………(4)

Hence, Total area of the paper used = (Area I + Area II + Area III + Area IV + Area V)

= (1) + (2) + (3) + (4)

= 2.5 + 6.5 + 1.29 +9

= 19.29 cm²

问题4.三角形和平行四边形具有相同的底面和相同的面积。如果三角形的边分别为26 cm，28 cm和30 cm，并且平行四边形位于底部28 cm上，请找到平行四边形的高度。

解决方案：

Now, Area of △AEB can be calculated by Heron’s Formula, where

AE = a = 28 cm

EB = b = 30 cm

AB = c = 26 cm

Semi Perimeter (s) = $\mathbf{\frac{a+b+c}{2}}$

s = (28+30+26)/2

s = 42 cm

ar(△AEB) = √s(s-a)(s-b)(s-c)

= √42(42-28)(42-30)(42-26)

= √42×(14)×(12)×(16)

= 336 cm²

As it is given, ar(△AEB) = ar(parallelogram ABCD)

336 = Base × Height

336 = 28 × h

h = $\frac{336}{28}$

h = 12 cm

Hence, the height of the parallelogram = 12 cm

问题5：菱形的田野上长满绿草，可放牧18头奶牛。如果菱形的每一侧为30 m，较长的对角线为48 m，那么每头母牛将获得多少草地面积?

解决方案：

ABCD is a rhombus having diagonal AC = 48 cm

side AB=BC=CD=AD=30 cm

Diagonal of Rhombus divides the area into two equal parts.

Now, ar(△ABC) can be calculated by Heron’s Formula, where

AB = a = 30 m

BC = b = 30 m

AC = c = 48 m

Semi Perimeter (s) = $\mathbf{\frac{a+b+c}{2}}$

s = $\frac{(30+30+48)}{2}$

s = 54 m

ar(△ABC) = √s(s-a)(s-b)(s-c)

= √54(54-30)(54-30)(54-48)

= √54×(24)×(24)×(6)

= 432 m²

Hence, Area of rhombus = 2 × (ar(△))

= 2 × 432 = 864 m²

Area for 18 cows = 864 m²

Area for each cow = 864 / 18 = 48 m²

问题6.伞是由10块三角形的两块不同颜色的布缝在一起制成的(见图12.16)，每块布分别为20厘米，50厘米和50厘米。每种雨伞需要多少颜色的布?

解决方案：

Let’s consider for each triangle.

Now, for each ar(△)can be calculated by Heron’s Formula, where

a = 50 cm

b = 50 cm

c = 20 cm

Semi Perimeter (s) = $\mathbf{\frac{a+b+c}{2}}$

s = $\frac{(50+50+20)}{2}$

s = 60 cm

ar(△) = √s(s-a)(s-b)(s-c)

= √60(60-50)(60-50)(60-20)

= √60×(10)×(10)×(40)

= 200√6 cm²

Hence, the Total Area = 5×200√6

= 1000√6 cm²

问题7.如图所示，方形风筝的对角线长32厘米，底边的等腰三角形长8厘米，边长6厘米，应由三种不同的阴影制成。用在里面吗?

解决方案：

As the area of kite is in the square, it area will be

Area of kite = $\mathbf{\frac{1}{2}}$×(diagonal)²

= $\frac{1}{2}$×32×32

= 512 cm²

Diagonal divides area into equal areas.

Area of kite = Area I + Area II

512 = 2 × Area I

Area I =Area II = 256 cm²……………………………..(1)

Area III: Area of triangle

Now, for each ar(△)can be calculated by Heron’s Formula, where

a = 6 cm

b = 6 cm

c = 8 cm

Semi Perimeter (s) = $\mathbf{\frac{a+b+c}{2}}$

s = $\frac{(6+6+8)}{2}$

s = 10 cm

ar(△) = √s(s-a)(s-b)(s-c)

= √10(10-6)(10-6)(10-8)

= √10×(4)×(4)×(2)

= 8√5 cm²………………………………(2)

问题8.地板上的花卉设计由16个三角形的瓷砖组成，三角形的边分别为9厘米，28厘米和35厘米(见图)。找到的50P速率每^平方厘米抛光瓷砖的成本。

解决方案：

Area for each triangle will be:

Now, for each ar(△)can be calculated by Heron’s Formula, where

a = 9 cm

b = 28 cm

c = 35 cm

Semi Perimeter (s) = $\mathbf{\frac{a+b+c}{2}}$

s = $\frac{(9+28+35)}{2}$

s = 36 cm

ar(△) = √s(s-a)(s-b)(s-c)

= √36(36-9)(36-28)(36-35)

= √36×(27)×(8)×(1)

= 36√6 cm²

As there are 16 tiles, so total area = 16 × 36√6

= 1410.906 cm²

As 1 cm² = 50 p = ₹ 0.5

1410.906 cm² = ₹ 0.5 ×1410.906

= ₹ 705.45

问题9.场为梯形，其平行边分别为25 m和10 m。非平行边为14 m和13 m。查找字段的区域。

解决方案：

AB = 25 m

EB = AB-AE = 25-10 = 15 m

Now, for ar(△ECB) can be calculated by Heron’s Formula, where

a = 13 m

b = 14 m

c = 15 m

Semi Perimeter (s) = $\mathbf{\frac{a+b+c}{2}}$

s = $\frac{(13+14+15)}{2}$

s = 21 m

ar(△ECB) = √s(s-a)(s-b)(s-c)

= √21(21-3)(21-14)(21-15)

= √21×(18)×(7)×(6)

= 84 m² ………………………….(1)

ar(△ECB) = $\mathbf{\frac{1}{2}}$ × Base × Height

84 m² = $\frac{1}{2}$ × 15 × h

h = $\frac{(84 × 2)}{15}$ m

h = 11.2 m

Total Area = Area of parallelogram AECD + ar(△ECB)

= (Base × Height) + 84m² 

= 10 × 11.2 + 84

Total Area = 196 m²