9类NCERT解决方案-第12章“苍鹭的公式” –练习12.1

问题1.一个交通信号板，指示“ SCHOOL AHEAD”，是带有边“ a”的等边三角形。使用Heron公式查找信号板的面积。如果其周长为180厘米，则信号板的面积为多少?

解决方案：

As it is mentioned here that, ΔABC is an equilateral triangle having side length = a.

So, here

AB =BC = AC = a

Perimeter of Equilateral triangle = 3× (Length of a side)

 = 3×a = 3a

and perimeter = 180 cm (given)

So, 3a = 180

a = 60 cm.

Hence, length of each side is 60 cm.

Now, Area of △ABC can be calculated by Heron’s Formula, where

AB = a = 60 cm

BC = b = 60 cm

AC = c = 60 cm

Semi Perimeter (s) = (a+b+c)/2

s = 180/2

s = 90 cm

ar(△ABC) = √s(s-a)(s-b)(s-c)

= √90(90-60)(90-60)(90-60)

= √90×(30)×(30)×(30)

= 900√3 cm²

Hence, the area of the signal board = 900√3 cm²

问题2.天桥的三角形侧壁已用于广告。墙的侧面分别为122 m，22 m和120 m(见图)。这些广告每年可产生₹5000每m ^2的收入。一家公司雇用了其中一堵墙，为期三个月。它支付了多少租金?

解决方案：

Here, Area of △ABC can be calculated by Heron’s Formula, where

AB = a = 120 m

BC = b = 22 m

AC = c = 122 m

Semi Perimeter (s) = (a+b+c)/2

s = (120+22+122)/2

s = 132 m

ar(△ABC) = √s(s-a)(s-b)(s-c)

= √132(132-120)(132-22)(132-122)

= √132×(12)×(110)×(10)

= 1320 m²

As it is given that,

For 1 year we cost

1 m² = ₹ 5000 

So, for 3 months,

1 m² = ₹ 5000 × (1/4)

For area of walls 1320 m² = 5000×(1/4)×(1320)

= ₹ 16,50,000

Hence, ₹ 16,50,000 much rent company will be pay for 3 months.

问题3.公园里有一张幻灯片。它的侧壁之一已涂上某种颜色，并带有“保持绿色和清洁”的信息(见图)。如果墙的边长分别为15 m，11 m和6 m，请查找涂有颜色的区域。

解决方案：

Here, Area of △ABC can be calculated by Heron’s Formula, where

AB = a = 11 m

BC = b = 6 m

AC = c = 15 m

Semi Perimeter (s) = (a+b+c)/2

s = (11+6+15)/2

s = 16 m

ar(△ABC) = √s(s-a)(s-b)(s-c)

= √16(16-11)(16-6)(16-15)

= √16×(5)×(10)×(1)

= 20√2 m²

Hence, the area painted in colour is 20√2 m²

问题4.找到三角形的面积，该三角形的两个边分别为18 cm和10 cm，周长为42 cm。

解决方案：

Here, length of two sides are given as 18 cm and 10 cm respectively.

and, perimeter = 42 cm.

Hence, length of third side = (Perimeter)-(length of two side)

= 42-(18+10)

AC = 14 cm

Here, Area of △ABC can be calculated by Heron’s Formula, where

AB = a = 18 cm

BC = b = 10 cm

AC = c = 14 cm

Semi Perimeter (s) = (a+b+c)/2

s = (18+10+14)/2

s = 21 cm

ar(△ABC) = √s(s-a)(s-b)(s-c)

= √21(21-18)(21-10)(21-14)

= √21×(3)×(11)×(7)

= 21√11 cm²

Hence, the area of triangle is 21√11 cm²

问题5.三角形的边之比为12:17:25，其周长为540厘米。查找其区域。

解决方案：

The ratio of the sides of the triangle are given as 12 : 17 : 25

Lets consider the common ratio between the sides of the triangle be p

Then, the sides are 12p, 17p and 25p

The perimeter of the triangle = 540 cm  (Given)

12p+17p+25p = 540 cm

54p = 540cm

So, p = 10

Hence, the sides of triangle are 120 cm, 170 cm, 250 cm.

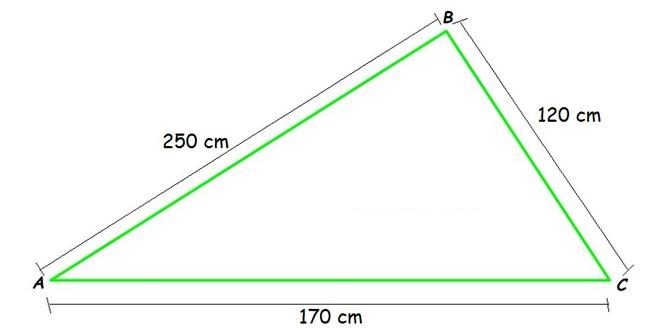
Here, Area of △ABC can be calculated by Heron’s Formula, where

AB = a = 250 cm

BC = b = 120 cm

AC = c = 170 cm

Semi Perimeter (s) = (a+b+c)/2

s = (250+120+170)/2

s = 270 cm

ar(△ABC) = √s(s-a)(s-b)(s-c)

= √270(270-250)(270-120)(270-170)

= √270×(20)×(150)×(100)

= 9000 cm²

Hence, the area of triangle is 9000 cm² .

问题6.等腰三角形的周长为30厘米，等边各为12厘米。找到三角形的面积。

解决方案：

Here, length of two equal sides of isosceles triangle are given as 12 cm.

and, perimeter = 30 cm.

Hence, length of third side = (Perimeter)-(length of two side)

= 30-(12+12)

AC = 6 cm

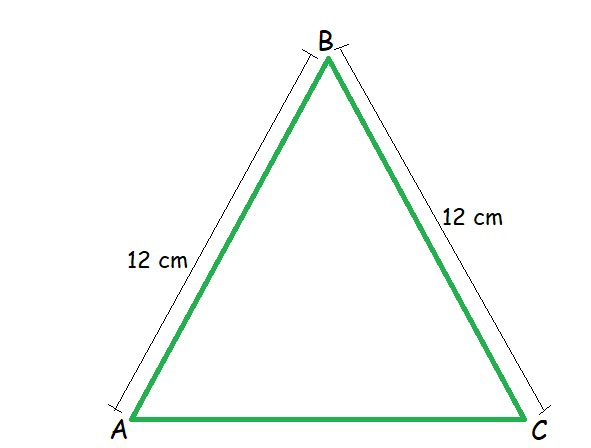
Here, Area of △ABC can be calculated by Heron’s Formula, where

AB = a = 12 cm

BC = b = 12 cm

AC = c = 6 cm

Semi Perimeter (s) = (a+b+c)/2

s = (12+12+6)/2

s = 15 cm

ar(△ABC) = √s(s-a)(s-b)(s-c)

= √15(15-12)(15-12)(15-6)

= √15×(3)×(3)×(9)

= 9√15 cm²

Hence, the area of triangle is 9√15 cm²