第9类RD Sharma解决方案–第16章圈子–练习16.3

问题1.三个女孩Ishita，Isha和Nisha站在公园画的半径20m的圆圈上玩游戏。石田向伊莎扔球，伊莎向尼莎扔球，尼莎向石田扔球。如果Ishita与Isha之间以及Isha与Nisha之间的距离均为24m，那么Ishita与Nisha之间的距离是多少?

解决方案：

Let A, B and C be the position of Ishita, Isha and Nisha respectively.
⇒ XA = AB = 24/2 = 12cm

⇒ OA = OB = OC {As they are radius of the circle.}
In triangle OXA,
⇒ OX² + XA² = OA²
⇒ OX² + 12² = 20²
⇒ OX² = 400 − 144
⇒ OX² = 256m²
⇒ OX = 16m

Now, let’s take an isosceles triangle ABC as shown above in figure 1.
The isosceles triangle’s altitude divides the base in equal parts.
So in triangle ABC,
⇒ ∠AZB = 90° and AZ = ZC
⇒ Area of triangle OAB = 1/2 × OX × AB { 1/2 × base × height}
⇒ 1/2 × AZ × OB = 1/2 × 16 × 24

⇒ AZ × 20 = 16 × 24
⇒ AZ = 19.2
⇒ AC = 2 × 19.2
⇒ AC = 38.4m
Hence, the distance between Ishita and Nisha is 38.4m.

问题2：一个殖民地内有一个半径为40m的圆形公园。三个男孩Ankur，Amit和Anand在边界处等距坐着，每个手里都拿着玩具电话互相交谈。查找每个电话的字符串长度。

解决方案：

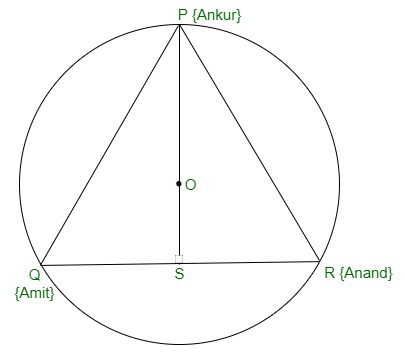
Given: PQ = QR = RP
Hence, PQR is an equilateral triangle.
⇒ OA = 40m {radius}
Median of an equilateral triangle passes through the circumcentre(O) of the equilateral triangle PQR.
The median of an equilateral triangle intersects each other at the ratio of 2:1.
As PS is the median of equilateral triangle PQR. So,
⇒  OP  =  2 
     OS       1
⇒  40m  =  2 
      OS        1
⇒ OS = 20m
Therefore, PS = OP + OS = 40m + 20m = 60m
In triangle PSR
⇒ PR² = PS² + SR² {By Pythagoras theorem}
⇒ PR² = 60² + ( QR )²
                            2
⇒ PR² = 3600 + ( PR )² {PR = QR} 
                              4
⇒ 3 PR² = 3600
    4
⇒ PR² = 4800
⇒ PR² = 40√3 m