用微积分基本定理求导

积分是微分的逆过程。它们也被称为反导数，用于找出我们没有可用公式的任意形状的面积和体积。不定积分只是计算函数的反导数，而定积分有极限，通常表示曲线下的面积。微积分的基本定理将积分规则与导数和链式规则联系起来。它用于解决集成中的难题。让我们看看这个定理。

应用微积分基本定理

将函数f(x) 视为在给定区间 [a, b] 内连续且可微的函数。这些限制之间的定积分表示为$\int^{b}_{a}f(x)dx$ .这被定义为由函数f(x) 和 x 轴在限制 x = a 和 x = b 之间包围的区域。这个定积分可以通过改变极限的上限转换成一个函数。这个函数可以重写为，

让$F(x) = \int^{x}_{a}f(t)dt$ .现在从几何上讲，这个函数给出了同一曲线下的面积，但从 x = a 到 x，其中 x 位于极限边界之间。下图显示了这个函数：

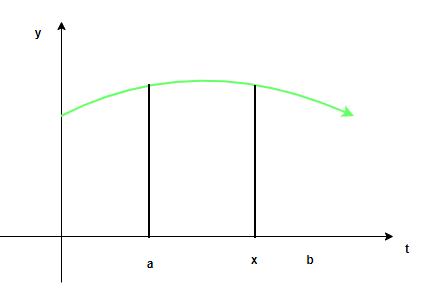
现在在 x = a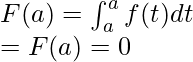， $F(a) = \int^{a}_{a}f(t)dt \\ = F(a) = 0$

基本定理使我们能够计算给定函数的导数。

Fundamental Theorem of Calculus – Part I

For a function f which is continuous and differentiable on the interval [a, b], suppose $F(x) = \int^{x}_{a}f(t)dt$. Then, F is a differentiable function on (a, b), and 

F'(x) = f(x)

这个定理看似微不足道，却有着非常深远的影响。有一个函数f(x) = x ² + sin(x)，

给定，F(x) = $\int^{x}_{-5}t^2 + sin(t)dt$

根据上述基本定理，

$\frac{d}{dx}F(x) = \frac{d}{dx}\int^{x}_{-5}(t^2 + sin(t))dt \\ F'(x) = x^2 + sin(x)$

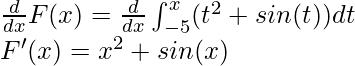
这个定理可以用来推导出一个流行的结果，

假设有一个定积分$\int^{b}_{a}f(t)dt$ .另外，假设 F(x) = $\int^{x}_{c}f(t)dt$ .

$\int^{b}_{a}f(t)dt \\ = \int^{c}_{a}f(t)dt + \int^{b}_{c}f(t)dt \\ = -\int^{a}_{c}f(t)dx + \int^{b}_{c}f(t)dt \\ = -F(a) + F(b) \\ = F(b) - F(a)$

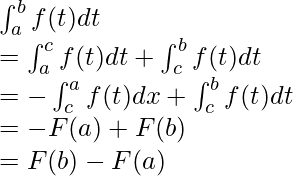
这是微积分基本定理的第二部分。

Fundamental Theorem of Calculus – Part II

For a function f which is continuous and differentiable on the interval [a, b], let F be any anti-derivative of the given function. Then, 

$\int^{b}_{a}f(x)dx = F(b)- F(a)$

使用链式法则应用基本定理

定积分的难题可以通过链式法则和微积分基本定理相结合来解决。例如，

$\frac{d}{dx}(\int^{x^2}_{1}cos(t)dt)$

为了解决这些问题，我们需要一个更广义的基本定理。

For a function f which is continuous and two other functions g and h which are differentiable, 

$\frac{d}{dx}\int^{h(x)}_{g(x)}f(s)ds = \frac{d}{dx}[F(h(x)) - F(g(x))] \\ = F'(h(x))h'(x) - F'(g(x))g'(x) \\ = f((h(x))h'(x) - f(g(x))g'(x)$

让我们看看与这些概念相关的一些问题。

示例问题

问题 1：给定以下函数F(x)，计算其导数。

F(x) = $\int_{0}^{x}cos(t)dt$

解决方案：

Given: F(x) = $\int_{0}^{x}cos(t)dt$

$\frac{d}{dx}(F(x)) = \frac{d}{dx}(\int_{0}^{x}cos(t)dt)$

This can be solved using the fundamental theorem of calculus part – I

$\frac{d}{dx}(F(x)) = \frac{d}{dx}(\int_{0}^{x}cos(t)dt) \\ = F'(x) = cos(x)$

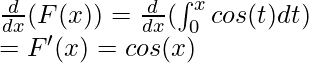
问题 2：给定以下函数F(x)，计算其导数。

F(x) = $\int_{0}^{x}(e^t + e^{-t})dt$

解决方案：

Given: F(x) = $\int_{0}^{x}(e^x + e^{-x})dt$

$\frac{d}{dx}(F(x)) = \frac{d}{dx}(\int_{0}^{x}(e^t + e^{-t})dt)$

This can be solved using the fundamental theorem of calculus part – I

$\frac{d}{dx}(F(x)) = \frac{d}{dx}(\int_{0}^{x}(e^t + e^{-t})dt) \\ F(x) = e^x + e^{-x}$

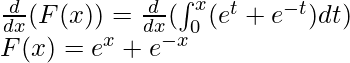
问题 3：给定以下函数F(x)，计算其导数。

F(x) = $\int_{0}^{x^2}e^tdt$

解决方案：

Given: F(x) = $\int_{0}^{x^2}e^tdt$

$\frac{d}{dx}(F(x)) = \frac{d}{dx}(\int_{0}^{x^2}e^tdt)$

This can be solved using the generalized form of the fundamental theorem of calculus part – I. It states that, 

$\frac{d}{dx}\int^{h(x)}_{g(x)}f(s)ds = \frac{d}{dx}[F(h(x)) - F(g(x))] \\ = F'(h(x))h'(x) - F'(g(x))g'(x) \\ = f((h(x))h'(x) - f(g(x))g'(x)$

Here, f(t) = e^t, h(x) = x² and g(x) = 0

$\frac{d}{dx}(\int^{x^2}_0e^tdt= e^{(h(x)}h'(x) - e^{g(x)}g'(x) \\ = \frac{d}{dx}(\int^{x^2}_0e^tdt = e^{x^2}(2x) - e^{0}(0) \\ = \frac{d}{dx}(\int^{x^2}_0e^tdt) = 2xe^{x^2}$

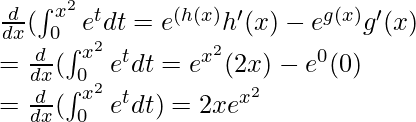
问题 4：给定以下函数F(x)，计算其导数。

F(x) = $\int_{0}^{x^3}cos(t)dt$

解决方案：

Given: F(x) = $\int_{0}^{x^3}cos(t)dt$

$\frac{d}{dx}(F(x)) = \frac{d}{dx}(\int_{0}^{x^3}cos(t)dt)$

This can be solved using the generalized form of the fundamental theorem of calculus part – I. It states that, 

$\frac{d}{dx}\int^{h(x)}_{g(x)}f(s)ds = \frac{d}{dx}[F(h(x)) - F(g(x))] \\ = F'(h(x))h'(x) - F'(g(x))g'(x) \\ = f((h(x))h'(x) - f(g(x))g'(x)$

Here, f(t) = cos(t), h(x) = x³ and g(x) = 0

$\frac{d}{dx}(\int^{x^3}_0cos(t)dt= cos((h(x))h'(x) - cos(g(x))g'(x) \\ = \frac{d}{dx}(\int^{x^3}_0cos(t)dt = cos(x^3)(3x^2) - cos(0)(0) \\ = \frac{d}{dx}(\int^{x^3}_0cos(t)dt) = 3x^2cos(x^3)$

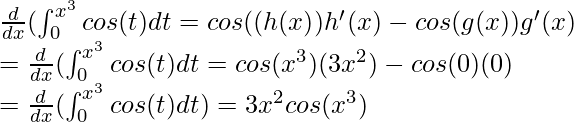
问题 5：给定以下函数F(x)，计算其导数。

F(x) = $\int_{0}^{sin(x)}(t + 2)dt$

解决方案：

Given: F(x) = $\int_{0}^{sin(x)}(t + 2)dt$

$\frac{d}{dx}(F(x)) = \frac{d}{dx}(\int_{0}^{sin(x)}(t + 2)dt)$

This can be solved using the generalized form of the fundamental theorem of calculus part – I. It states that, 

$\frac{d}{dx}\int^{h(x)}_{g(x)}f(s)ds = \frac{d}{dx}[F(h(x)) - F(g(x))] \\ = F'(h(x))h'(x) - F'(g(x))g'(x) \\ = f((h(x))h'(x) - f(g(x))g'(x)$

Here, f(t) = t + 2, g(x) = 0 and h(x) = sin(x)

$\frac{d}{dx}(\int^{sin(x)}_0(t + 2)dt)= (h(x) + 2)h'(x) - (g(x)+2)g'(x) \\ = \frac{d}{dx}(\int^{sin(x)}_0(t + 2)dt) = (sin(x) + 2)(cos(x)) - (0 + 2)(0) \\ = \frac{d}{dx}(\int^{sin(x)}_0(t + 2)dt) = cos(x)(sin(x) + 2)$

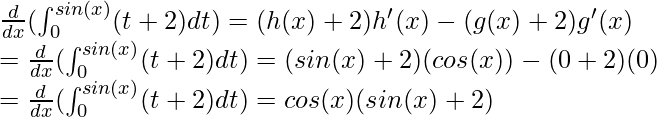
问题 6：给定以下函数F(x)，计算其导数。

F(x) = $\int_{0}^{cos(x)}(t)dt$

解决方案：

Given: F(x) = $\int_{0}^{cos(x)}(t)dt$

$\frac{d}{dx}(F(x)) = \frac{d}{dx}(\int_{0}^{cos(x)}tdt)$

This can be solved using the generalized form of the fundamental theorem of calculus part – I. It states that, 

$\frac{d}{dx}\int^{h(x)}_{g(x)}f(s)ds = \frac{d}{dx}[F(h(x)) - F(g(x))] \\ = F'(h(x))h'(x) - F'(g(x))g'(x) \\ = f((h(x))h'(x) - f(g(x))g'(x)$

Here, f(t) = t, g(x) = 0 and h(x) = cos(x)

$\frac{d}{dx}(\int^{cos(x)}_0tdt)= (h(x))h'(x) - (g(x))g'(x) \\ = \frac{d}{dx}(\int^{cos(x)}_0tdt) = (cos(x))(-sin(x)) - (0)(0) \\ = \frac{d}{dx}(\int^{cos(x)}_0tdt) = -cos(x)sin(x)$