差商公式

差商公式是函数导数定义的一部分。可以通过应用极限 h 趋向于零来获得函数的导数，即 h ⇢ 0 对差商函数。差商公式给出了割线的斜率。割线是通过曲线的两个点的线。

让我们考虑曲线 y = f(x) 和通过两点的割线是 (x, f(x)) 和 (x + h, f(x+h)) 那么差商公式由下式给出-

异商公式

$\frac{f(x+h)-f(x)}{h}$

Where,

f(x + h) is function by replacing x with x + h in f(x)

f(x) is given function.

差商公式证明

Let’s consider the curve y = f(x) and the secant line that passes through the two points are (x, f(x)) and (x + h, f(x + h)).

Given,

(x₁, y₁) = (x, f(x))

(x₂, y₂) = (x + h, f(x + h))

Find the slope of the secant line,

Slope = (y₂ – y₁)/(x₂ – x₁) 

= (f(x + h) – f(x))/(x + h – x)

= (f(x + h) – f(x))/h

So the different quotient formula is slope of the secant line that passes through the given points.

示例问题

以下是一些关于差商公式的示例问题，涵盖了主要类型的问题。

问题 1：函数f(x) = 7x + 9 的差商公式是什么？

解决方案：

Given,

f(x) = 7x + 9

Difference quotient formula = (f(x + h) – f(x))/h

= ((7(x + h) + 9) – (7x + 9))/h

= (7x + 7h + 9 – 7x – 9)/h

= 7h/h

= 7

Difference quotient formula for the given function is 7.

问题 2：函数f(x) = 7x ² – 1 的差商公式是什么？

解决方案：

Given,

f(x) = 7x² – 1

Difference quotient formula = (f(x + h) – f(x))/h

= ((7(x + h)² – 1) – (7x² – 1))/h

= ((7(x² + h² + 2xh) – 1) – (7x² – 1))/h

= (7x² + 7h² + 14xh – 1 – 7x² + 1)/h

= (7h² + 14xh)/h

= h(7h + 14x)/h

= 7h + 14x

Difference quotient formula for the given function is 7h + 14x.

问题 3：函数f(x) = 25x 的差商公式是什么

解决方案：

Given,

f(x) = 25x

Difference quotient formula = (f(x + h) – f(x))/h

= ((25(x + h)) – (25x))/h

= (25x + 25h – 25x))/h

= 25h/h

= 25

Difference quotient formula for the given function is 25.

问题 4：函数f(x) = √(x – 2) 的差商公式是什么

解决方案：

Given,

f(x) = √(x – 2)

Difference quotient formula = (f(x + h) – f(x))/h

= (√(x + h – 2) – √(x – 2))/h

$=\frac{\sqrt{x+h-2}-\sqrt{x-2}}{h}\times\frac{\sqrt{x+h-2}+\sqrt{x-2}}{\sqrt{x+h-2}+\sqrt{x-2}} =\frac{\sqrt{x+h-2}^2-\sqrt{x-2}^2}{h(\sqrt{x+h-2}+\sqrt{x-2})} =\frac{x+h-2-x+2}{h(\sqrt{x+h-2}+\sqrt{x-2})} =\frac{h}{h(\sqrt{x+h-2}+\sqrt{x-2})} =\frac{1}{\sqrt{x+h-2}+\sqrt{x-2}}$

Difference quotient formula for the given function is 1/(√(x + h – 2) + √(x – 2)).

问题 5：函数f(x) = 1/x 的差商公式是什么？

解决方案：

Given,

f(x) = 1/x

Difference quotient formula = (f(x + h) – f(x))/h

$=\frac{\frac{1}{x+h}-\frac{1}{x}}{h} =\frac{x-(x+h)}{h(x)(x+h))} =\frac{x-x-h}{h(x)(x+h)} =\frac{-h}{h(x)(x+h)} =\frac{-1}{(x)(x+h)}$

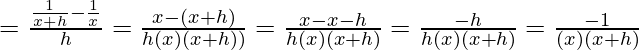
Difference quotient formula for the given function is -1/(x)(x + h)

问题 6：求函数f(x) = 2x – 1 的差商

解决方案：

Given f(x) = 2x – 1

Difference quotient = (f(x + h) – f(x))/h

= (2(x + h) – 1 – (2x – 1))/h

= (2x + 2h – 1 – 2x + 1)/h

= 2h/h

= 2

Hence Difference quotient for the function 2x – 1 is 2.

问题 7：函数f(x) = log(x) 的差商是多少

解决方案：

Given f(x) = log(x)

Difference Quotient = (f(x + h) – f(x))/h

= (log(x + h) – log(x))/h

From Quotient property of logarithms log(a) – log(b) = log(a/b)

= log((x + h)/x)/h

So the difference quotient for the given function is log((x + h)/x)/h