伯努利方程

当经常被问到飞机如何产生升力或真正酷的喷漆枪如何工作时，最常见的答案是伯努利原理——一个看似简单的两个词的答案，坦率地说是一个多层次的现象。流体的这个概念及其机械特性似乎是一个难以理解的话题，主要是由于冗长的推导方程和不吸引人的表示。但是，请记住，理解这个想法是关键。可视化这个概念可以为任何问题解决者创造奇迹。

在本文中，我们将帮助建立伯努利原理的定义和视角，以及相关的方程（就像物理学中曾经解释过的一切——使用方程）。

伯努利原理

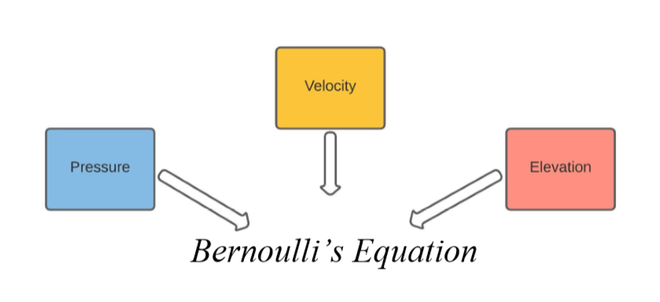
如上所示，伯努利方程只是一个数学表达式，有助于将流体的速度、压力和高度联系起来。建立这种关系时要牢记在流体的整个运动过程中能量是守恒的。因此，我们着眼于与流体相关的能量形式。

1. 流体压力产生的能量（静压）：这只是流体静止并向容器壁提供压力时系统的能量。它实际上是静压。
2. 流体运动引起的动能：与运动中的任何质量相关的明显能量——这里流体的运动导致这种能量形式的存在。另一种解释方式是与动态压力相关的能量。
3. 来自高程的重力势能：势能是一个颇具参考性的概念。在这里，流体的高度解释了这种势能的积累。换句话说，这是由于静水压力引起的。

因此，根据定义：

The total mechanical energy – which is defined as the sum of all these energies (kinetic, gravitational potential and fluid), must stay constant unless any work is done on the system. This is the Bernoulli’s Principle.

因此，可以写成下面的表达式：

$P + \frac{1}{2} \rho V^2 + \rho g h = constant$

使用该表达式可以得出的最明显的关系是流体压力与其速度之间的比例关系。在任何恒定的特定高度，重力势能也可以被视为一个常数。因此，我们留下了以下表达和理解。

P + (½)ρv² = k

∴ P = k – (½)ρv²

As P increases, v decreases.

显然，压力和速度成反比。这个想法将在后续章节中进一步应用。请注意，我们仍然围绕着这个原则。我们需要推导出方程，以便我们可以处理同一系统的不同实例。

伯努利方程

既然已经确定伯努利原理是能量守恒定律的体现，我们将继续考虑将流体沿流线从一点移动到另一点所做的功。我们用 ρ 表示流体的密度。

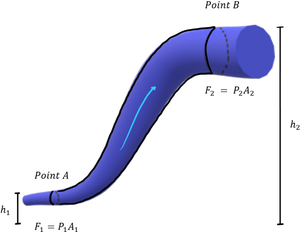
流体从 A 点流向 B 点

我们假设 A 点和 B 点的流体分别在高度 h ₁和 h ₂处升高。很明显，h ₂处的引力势能更大。另外，观察 A 点的横截面积为 A ₁ ，B 点的横截面积为 A ₂ 。现在，如果要做一些工作，流体必须移动一定距离，这也意味着相关的速度。

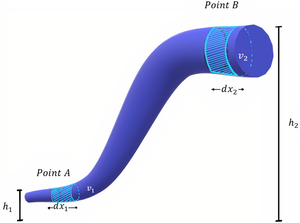
如果流体从 A 点移动 dx ₁ ，从 B 点移动 dx ₂ ，它应分别达到 v ₁和 v ₂的速度。我们将连同以下表达式一起计算出我们的推导：

dW = d(Kinetic Energy of Fluid) + d(Gravitational Potential of Fluid) 

or

dW = dK + dU

流体从 A 点移动到 B 点时所做的功为 dW = dW ₁ – dW ₂ 。由于流体被 dx ₁和 dx ₂取代，我们根据 A 点和 B 点所受的力来重写表达式。

dW = F ₁ .dx ₁ – F ₂ .dx ₂ = P ₁ .A ₁ .dx ₁ – P ₂ .A ₂ .dx ₂

F ₁和 F ₂分别是流体在 A 点和 B 点所受的力。现在，回想一下，力是每单位面积所承受的压力。然后可以修改 dW 的表达式。请注意，A ₁ dx ₁ = A ₂ dx ₂ = 微分体积（元素/小体积），因为任何面积乘以相邻长度都会得到体积。假设 dV 是微分体积。

dW = P ₁ .dV – P ₂ .dV = (P ₁ -P ₂ ).dV

同时，流体动能的表达式可以用流体在 A 和 B 处的质量和速度来表示。我们将使用与运动中的质量相关的动能的标准表达式，即 dK = 0.5m ₂ v ₂ ² – 0.5m ₁ v ₁ ²

请记住，我们已经获得了流体密度 (ρ) 和流体体积差 (dV)。由于我们希望使用更少的项，我们可以交替使用上面的来表示 A 点和 B 点处的流体质量。因此，我们有：

dK = ½ρdV (v ₂ ² – v ₁ ² )

相关的引力势能相当简单。再一次，我们可以通过替代地表达质量来进一步简化它，即质量是密度乘以体积。 GPE 用 dU 表示。

dU = m ₁ gh ₁ – m ₂ gh ₂ = ρdVg (h ₂ – h ₁ )。

通过将等式的两侧（dW = dK + dU）相等，我们得到以下表达式：

(P ₁ – P ₂ )dV = ½ρdV (v ₂ ² – v ₁ ² ) + ρdVg (h ₂ – h ₁ )

P ₁ – P ₂ = ½ρv ₂ ² – ½ρv ₁ ² + ρgh ₂ – ρgh ₁

$\mathbf{{\color{DarkGreen}P_{1}+\frac{1}{2}\rho v_{1}^{2}+\:\rho gh_{1} = P_{2}+\frac{1}{2}\rho v_{2}^{2}\;+\rho gh_{2}}}$

这实际上是伯努利方程。

在推导这个表达式时，我们确实做了一些假设，以便找出问题的动态性。一个非常重要的假设是流体的不可压缩性。该属性使我们能够使密度相对于管道不同点的压力保持不变。这如何影响我们的方程已在下面解释。

连续性方程

密度的变化——即使不是那么大，即使是很小的变化——在现实中也很普遍。因此，没有流体是完全不可压缩的。您可能会说液体比气体更不可压缩，但并非字面上不可压缩。话虽如此，这会影响能量计算所考虑的流体质量。

由于密度始终保持恒定，只要体积也保持相等，采样质量也保持相等 - 上述计算中使用的体积是微分/元素，即 dV，因此相等。结果，我们的群众是平等的。我们怎样才能更好地理解这一点？

不同的观点：质量守恒

考虑下图。

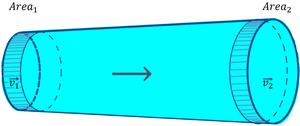
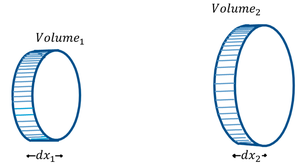
本文接下来的几行将紧贴质量守恒的思想——一定质量在开始时流入管道的速率必须与其离开管道的速率相同。这是至关重要的。

Rate at which mass enters = Rate at which the mass leaves

质量本质上是密度 (ρ) 乘以体积。因此，我们可以用这些术语来表达质量，并将流动的不同实例等同起来——在开始和结束时。由于压力不同，速度肯定会有所不同。到目前为止，我们有，

ρdV ₁ = ρdV ₂

其中 V ₁是开头的体积（管道的左侧）。 V ₂是最后的体积（最右边的阴影区域）。不要将它们与速度v ₁和v ₂混淆。体积可以可视化为圆柱体，就其横截面积和高度而言 - 圆柱体的体积表示为横截面积 x 其身体的高度。因此，如果圆柱体的高度为 dx ₁和 dx _2，

  ρA ₁ dx ₁ = ρA ₂ dx ₂

由于我们正在处理质量的“速率”，因此我们将以相等的时间间隔 Δt 测量流量。我们可以用流体的速度 v ₁和 v ₂来表示位移。

ρ.A₁.v₁.Δt = ρ.A₂.v₂.Δt

∴ A₁v₁ = A₂v₂

这就是连续性方程。在处理伯努利原理的几个应用时，它会派上用场。

伯努利原理的应用

(1) 撞船

过去一个可怕的故事，两艘船在彼此平行行驶时相撞，这是伯努利原理的一个经典例子。

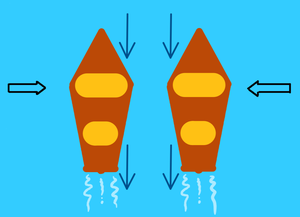
船外压力较高会导致碰撞

想象两艘船以相同的速度相互平行航行。由于船的中间部分最宽，因此它充当了两艘船之间的水的漏斗或挤压管。水通过的面积的减少和增加导致速度增加（伯努利原理）。然而，一旦速度增加，船之间的压力就会降低。结果，为了稳定这种较低的压力，外部的水向内推动，导致船只相互靠近。

(2) 翼型和升力

许多人将飞行背后的原因归因于伯努利原理。然而，在产生飞机起飞所需的升力时，另一个原理 - 康达效应 - 也在起作用。

入射空气必须在机翼上方和下方通过。这样做时，空气会在机翼顶部上方移动一段额外的距离。根据附壁效应，空气沿着曲面传播得更快。因此，空气的速度高于翼型。根据伯努利原理，较高的流体速度会导致相应的较低压力区域。同时，翼型下方的压力相对较高。这种压力差异导致向上的力——升力——这是飞行和飞机机翼结构背后的唯一原因。

(3) 旋转球偏离其轨迹

观察到任何正常投掷（没有任何旋转）的球都会受到重力的影响并勾勒出抛物线轨迹。然而，当球旋转时，它会偏离其轨迹并根据旋转方向和相对空气而上升或下降。

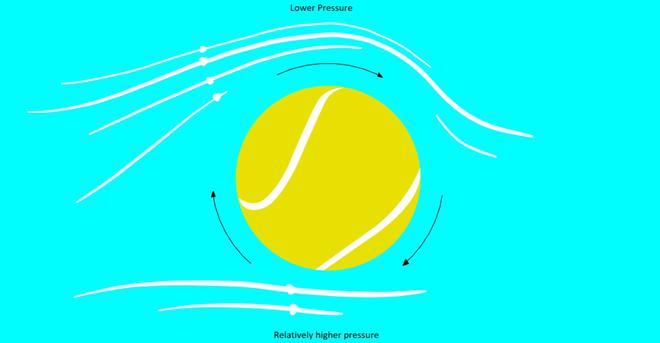
当小球旋转，空气向小球移动时，空气的流线也随着小球被拖拽，就是我们在上图上半部分看到的。同时，球下方的空气方向与旋转相反，这导致阻力最小（流线正常继续）。由于上方的空气必须在球上方并与球一起移动更远的距离，因此它必须以更大的速度移动。根据伯努利原理，较高的速度与较低的压力相关。这种速度差异以及随后产生的压力会导致动态升力，从而导致球上升。这种效应也被称为马格努斯效应，它是所有球类运动中的一个主要话题，因为旋转的动作会导致偏离运动（根据情况需要或不需要）。

示例问题

问题 1. 既然我们已经了解了如何使用伯努利原理来解释飞行，那么当飞机倒飞时，您将如何解释飞行？

解决方案：

Consider an airfoil, except inverted along the x-axis.

The confusing bit lies in how there is a lower pressure region underneath due to higher velocity along the streamlines. Also, the air is slower above which means the plane would logically crash as it is pushed downwards! Here’s the clever bit though. Airplanes such as stunt planes or fighter aircraft often resort to symmetrical wings which may tilt to provide lift. Since they are largely symmetrical, the velocities above and below are relatively equal. However, to generate the lift, tilting the wings upward by a minute angle helps keeping the plane in flight. This tilt is also sometimes known as the angle of attack.

问题 2：两个分别具有横截面积 A 和 2A 的圆柱形罐保持在水平地板上。第一个罐装满高度 H，而另一个是空的。如果它们通过在时间 t=0 时底部为“a”且横截面可忽略不计的管道连接，请计算两个水箱中的高度需要多长时间才能相等。

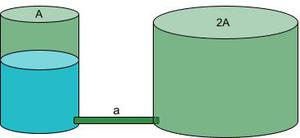
解决方案：

Take the cylinder on the left as point 1, the pipe as point 2, and the cylinder on the right as pipe 3. Many students make the mistake of directly applying Bernoulli’s equation between the two cylinders. This is incorrect since there is a loss of energy when liquid flows from the pipe to cylinder 3 due to inelastic collisions when the liquid collides with some mass of the liquid in cylinder 3 as it fills up (look below).

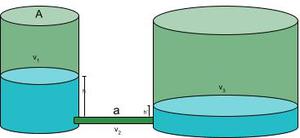
There is conservation of energy only between cylinder 1 and the pipe. However, there is conservation of mass throughout! This means we may use the equation of continuity. Therefore, we have the following:

A₁v₁ = av₂ = A₂v₃  (equation of continuity)

Now, take the difference in heights to be y i.e., h – h’ = y

We need this difference to equal 0 as the heights will be equal at some point. The rate or how quickly this happens can be written as a differential.

dy/dt = -(v₁ + v₃)  ……………v₁ is downwards and v₃ is upwards)

⇒ dy/dt = -v₁(1+A₁/A₂) ………by taking v₁ common and writing v₃/v₁ using the equation of continuity.

$\therefore v_{1} = \frac{-A_{2}}{A_{1}+A_{2}}\left(\frac{dy}{dt} \right)$

Now, let’s apply Bernoulli’s Equation between cylinder 1 and the pipe:

P₁ + (1/2)ρv₁² +ρgh = P2 + (1/2)ρv₂² + ρgh’

⇒ ρg(h – h’) = (1/2)ρv₁²((A₁²/a²) – 1) ……………by rearranging and using the equation of continuity for (v₂/v₁).

$\sqrt{2gy} = \frac{-A_{2}}{A_{1}+A_{2}}\left(\frac{dy}{dt}\right)\frac{A_{1}}{a}$

In the previous step we are approximating ((A₁²/a²) – 1) to A₁/a since a<1. In the next step we shall integrate the differential equation by bringing dy to one side and dt on the other.

$-\int_{H}^{0}\frac{dy}{\sqrt{y}} = \frac{A_{1}A_{2}}{A_{1}+A_{2}}\left( \frac{1}{a}\right)\int_{0}^{t}dt$

The limits for y tend from H to 0 since we want the differences in heights to equal 0 at the end.

Time elapsed would be = $\frac{A_{1}A_{2}}{A_{1}+A_{2}}\left( \frac{1}{a}\right) \sqrt{\frac{2H}{g}}$

问题3：水在A点以5 m/s的速度流过横截面积为4 m ²的水平管道，压力为0.3 MPa。在B点，横截面积为2 m ² 。 B点的水流速度是多少？计算 B 点的压力。

解决方案：

Let’s visualize the case presented to us.

Using the equation of continuity, we can solve for the speed at point B. A₁ x v₁ = A₂ x v₂

Therefore, v₂ = (A₁ x v₁)/A₂

⇒ v₂ = (4 x 5)/2 m/s = 10 m/s

Now let’s calculate the velocity at point B. Notice how the velocity has increased. This means the pressure would decrease in proportion – as per Bernoulli’s Principle. This will help us verify our answer. Using Bernoulli’s Equation:

P₁ + (1/2)ρv₁² + ρgh = P₂ + (1/2)ρv₂² + ρgh ……….since the fluid’s elevation is the same.

⇒ 0.3×10⁶ + 0.5x1000x25 = P₂ + 0.5x1000x10²

⇒ P₂ = 0.2625 MPa

问题 4. 水以 4 m/s 的速度和 250 kPa 的压力流过恒定半径 = 10 cm 的管道。找出出口处的速度，并说明在此计算中使用了哪种守恒定律。 B点的压力是多少？

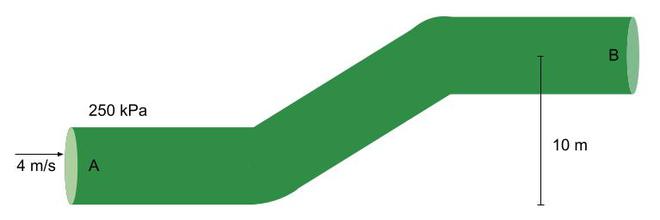
解决方案：

If the radius of the pipe stays constant throughout, the cross-sectional area of the outlet would stay the same. Based on the equation of continuity, A₁ x v₁ = A₂ x v₂, since the areas are the same, the speed of the water at the outlet is 4 m/s. v₂ = 4 m/s . The equation of continuity is based on the Conservation of Mass.

Using the Bernoulli’s Equation, substitute the values of pressure velocity and height at point A and the velocity and elevation at point B. Take fluid density as 1000 kg/m³.

⇒ 250 kPa + 0.5x1000x16 + 1000×9.8×0 = P₂ + 1000×9.8×10 + 0.5x1000x16 

⇒ P₂ = 152 kPa

问题5：参考下图，将A、B、C点的压力按升序排列。对 A、B和 C点的速度执行相同操作。

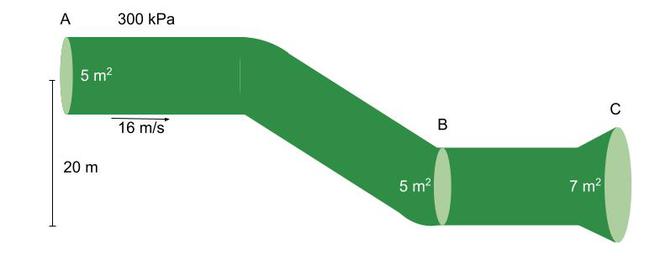
解决方案：

Let’s calculate the speeds first. We know that v_a = 16 m/s. Since the areas at point A and point B are the same, the velocities will also remain the same. Many students may find this confusing since speed increases as you fall down. However, in this case, the pressure is increasing due to a change in height – remember to take note of this. So, v_b = 16 m/s

Using the equation of continuity, A_b x v_b = A_c x v_c . Therefore, v_c = (5/7) x 16 m/s = 11.429 m/s.

⇒ v_a = v_b > v_c

Now we can find the pressure at point B using the speeds obtained. From Bernoulli’s equation between A and B, we can find the pressure at point B.

P_a + (1/2)ρv_a² + ρgh = P_b + (1/2)ρv_b² + ρgh , since the speeds at point A and B are the same.

⇒ 300 kPa + 1000×9.8×16 = P_b + 1000×9.8×0

⇒ P_b = 156.8 kPa

Using Bernoulli’s Equation between points A and C we get the following:

P_a + (1/2)ρv_a² + ρgh = P_c + (1/2)ρv_c² + ρgh ⇒ 300 + 0.5x1000x256 + 1000×9.8×20 = P_c + 0.5x1000x(11.429)² + 1000×9.8×0

⇒ P_c = 258.9 kPa

⇒ P_a < P_b < P_c

问题 6.房屋的设计可承受最大风速为 80 m/s 的巨浪。屋顶的表面积为 A = 450 m。屋顶支架能承受多大的力？取空气密度 = 1.029 kg/m ³

解决方案：

Assume two points around the roof – one outside and the other inside. On a larger scale, the distance between the points would be quite small. Refer to the figure below.

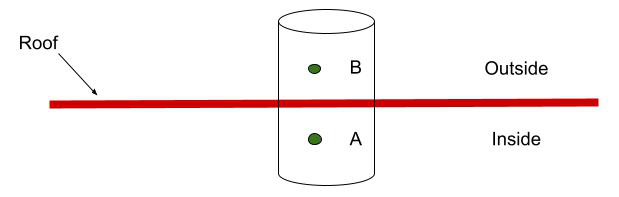
Logically, we must not feel the winds inside the house. So we may assume the velocity inside or v_a = 0. Since, the points are quite close to eachother, they may be assumed as elevated at the same height. Our Bernoulli’s Equation is now quite simple.

⇒ P_A + (1/2)ρv_A² + ρgh = P_B + (1/2)ρv_B² + ρgh 

Since v_A = 0, ⇒ P_A – P_B = (1/2)ρv_B²

⇒ ΔP = (1/2)ρv_B²

⇒ Force / Area of Roof = ΔP

⇒ Force = 0.5ρv_B² x Area

⇒ Force = 0.5 x 1.029 x 80² x 450

The roof can withstand a maximum force of 1.481 x 10⁶ N.