给定图中元素属于不同连通分量的最大对

给定一个具有从0 到 (N-1)编号的N个顶点的图和一个表示图边缘的矩阵edges[][] ，任务是找出可以形成的最大对数，其中每个元素pair 属于图的不同连通分量。

例子：

Input: N = 4, edges = {{1, 2}, {2, 3}}
Output: 3
Explanation: Total nodes 4 (from 0 to 3). 
There are 3 possible pairs: {0, 1}, {0, 2} and {0, 3}.
No pair between {1, 2} or {1, 3} or {2, 3} because they belong to the same connected component.
 

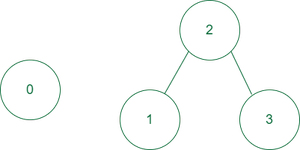
graph with given edges

Input: N = 2, edges = {0, 1}
Output: 0
Explanation: All the elements belong to the same connected component.

方法：可以通过计算连通分量的数量和每个连通分量中的节点数来解决问题。从给定的 N 个节点可以形成总共N*(N-1)/2个节点。但是，要获得所需的对数，减去每个连接组件的节点之间可以形成的对数。请按照以下步骤操作：

• 将total初始化为可能对的总数，即N*(N-1)/2 。
• 使用DFS查找不同的连接组件和每个组件：
  • 找出该连接组件中的节点数并将其存储在变量中（例如cnt ）。
  • 减去这些节点之间可以形成的对数，即cnt*(cnt – 1)/2 。
• 访问完所有节点后， total的剩余值就是最终的答案。

下面是上述方法的实现

C++

// C++ code to implement the approach
#include 
using namespace std;
 
// DFS function
void dfs(vector adj[], int src,
         bool visited[], int& cnt)
{
    visited[src] = true;
 
    // Count number of nodes
    // in current component
    cnt++;
    for (auto it : adj[src]) {
        if (!visited[it]) {
            dfs(adj, it, visited, cnt);
        }
    }
}
 
// Function to count total possible pairs
int maxPairs(int N,
             vector >& edges)
{
    vector adj[N];
 
    // Building the adjacency matrix
    for (int i = 0; i < edges.size(); i++) {
        adj[edges[i][0]].push_back(
            edges[i][1]);
        adj[edges[i][1]].push_back(
            edges[i][0]);
    }
 
    // Maximum total pairs
    int total = N * (N - 1) / 2;
 
    // Array to keep track of components
    bool visited[N + 1] = { false };
 
    // Loop to count total possible pairs
    for (int i = 0; i < N; i++) {
        if (visited[i] == false) {
            int cnt = 0;
 
            dfs(adj, i, visited, cnt);
 
            // Subtract pairs from
            // the same connected component
            total -= (cnt * (cnt - 1) / 2);
        }
    }
    return total;
}
 
// Driver code
int main()
{
    int N = 4;
    vector > edges = { { 1, 2 },
                                   { 2, 3 } };
 
    int result = maxPairs(N, edges);
    cout << result;
    return 0;
}

Java

import java.io.*;
import java.util.*;
 
class GFG {
 
  static int count = 0;
 
  // DFS function
  public static void
    dfs(ArrayList > adj, int src,
        boolean visited[])
  {
    visited[src] = true;
 
    // Count number of nodes
    // in current component
    count++;
    for (int it : adj.get(src)) {
      if (!visited[it]) {
        dfs(adj, it, visited);
      }
    }
  }
 
  // Function to count total possible pairs
  public static int
    maxPairs(int N, ArrayList > edges)
  {
    ArrayList > adj
      = new ArrayList >();
 
    for (int i = 0; i < N; i++) {
 
      ArrayList temp
        = new ArrayList();
      adj.add(temp);
    }
 
    // Building the adjacency matrix
    for (int i = 0; i < edges.size(); i++) {
 
      ArrayList temp
        = adj.get(edges.get(i).get(0));
      temp.add(edges.get(i).get(1));
      adj.set(edges.get(i).get(0), temp);
 
      temp = adj.get(edges.get(i).get(1));
      temp.add(edges.get(i).get(0));
      adj.set(edges.get(i).get(1), temp);
    }
 
    // Maximum total pairs
    int total = N * (N - 1) / 2;
 
    // Array to keep track of components
 
    boolean[] visited = new boolean[N + 1];
 
    for (int i = 0; i <= N; i++) {
      visited[i] = false;
    }
 
    // Loop to count total possible pairs
    for (int i = 0; i < N; i++) {
      if (visited[i] == false) {
 
        count = 0;
 
        dfs(adj, i, visited);
 
        // Subtract pairs from
        // the same connected component
        total -= (count * (count - 1) / 2);
      }
    }
    return total;
  }
  // Driver code
  public static void main(String[] args)
  {
 
    int N = 4;
    ArrayList > edges
      = new ArrayList >();
    edges.add(
      new ArrayList(Arrays.asList(1, 2)));
    edges.add(
      new ArrayList(Arrays.asList(2, 3)));
 
    int result = maxPairs(N, edges);
    System.out.println(result);
  }
}
 
// This code is contributed by Palak Gupta

Python3

# python3 code to implement the approach
 
 
visited = []
cnt = 0
 
# DFS function
 
 
def dfs(adj, src):
    global visited
    global cnt
    visited[src] = True
 
    # Count number of nodes
    # in current component
    cnt += 1
    for it in adj[src]:
        if (not visited[it]):
            dfs(adj, it)
 
 
# Function to count total possible pairs
def maxPairs(N, edges):
    global visited
    global cnt
    adj = [[] for _ in range(N)]
 
    # Building the adjacency matrix
    for i in range(0, len(edges)):
        adj[edges[i][0]].append(edges[i][1])
        adj[edges[i][1]].append(edges[i][0])
 
        # Maximum total pairs
    total = (N * (N - 1)) // 2
 
    # Array to keep track of components
    for i in range(N + 1):
        visited.append(False)
 
        # Loop to count total possible pairs
    for i in range(0, N):
        if (visited[i] == False):
            cnt = 0
 
            dfs(adj, i)
 
            # Subtract pairs from
            # the same connected component
            total -= ((cnt * (cnt - 1)) // 2)
 
    return total
 
 
# Driver code
if __name__ == "__main__":
 
    N = 4
    edges = [[1, 2], [2, 3]]
 
    result = maxPairs(N, edges)
    print(result)
 
    # This code is contributed by rakeshsahni

C#

using System;
using System.Collections.Generic;
class GFG
{
 
  static int count = 0;
 
  // DFS function
  public static void
    dfs(List> adj, int src, bool[] visited)
  {
    visited[src] = true;
 
    // Count number of nodes
    // in current component
    count++;
    foreach (int it in adj[src])
    {
      if (!visited[it])
      {
        dfs(adj, it, visited);
      }
    }
  }
 
  // Function to count total possible pairs
  public static int
    maxPairs(int N, List> edges)
  {
    List> adj = new List>();
 
    for (int i = 0; i < N; i++)
    {
 
      List temp = new List();
      adj.Add(temp);
    }
 
    // Building the adjacency matrix
    for (int i = 0; i < edges.Count; i++)
    {
 
      List temp = adj[edges[i][0]];
      temp.Add(edges[i][1]);
      adj[edges[i][0]] = temp;
 
      temp = adj[edges[i][1]];
      temp.Add(edges[i][0]);
      adj[edges[i][1]] = temp;
    }
 
    // Maximum total pairs
    int total = N * (N - 1) / 2;
 
    // Array to keep track of components
    bool[] visited = new bool[N + 1];
 
    for (int i = 0; i <= N; i++)
    {
      visited[i] = false;
    }
 
    // Loop to count total possible pairs
    for (int i = 0; i < N; i++)
    {
      if (visited[i] == false)
      {
 
        count = 0;
 
        dfs(adj, i, visited);
 
        // Subtract pairs from
        // the same connected component
        total -= (count * (count - 1) / 2);
      }
    }
    return total;
  }
   
  // Driver code
  public static void Main()
  {
 
    int N = 4;
    List> edges = new List>();
    edges.Add(new List());
    edges.Add(new List());
    edges[0].Add(1);
    edges[0].Add(2);
    edges[1].Add(2);
    edges[1].Add(3);
    int result = maxPairs(N, edges);
    Console.Write(result);
  }
}
 
// This code is contributed by Saurabh Jaiswal

Javascript

      // JavaScript code for the above approach
 
      // DFS function
      function dfs(adj, src,
          visited, cnt) {
          visited[src] = true;
 
          // Count number of nodes
          // in current component
          cnt++;
          for (let it of adj[src]) {
              if (!visited[it]) {
                  dfs(adj, it, visited, cnt);
              }
          }
          return cnt;
      }
 
      // Function to count total possible pairs
      function maxPairs(N,
          edges) {
          let adj = new Array(N).fill([]);
 
          // Building the adjacency matrix
          for (let i = 0; i < edges.length; i++) {
              adj[edges[i][0]].push(
                  edges[i][1]);
              adj[edges[i][1]].push(
                  edges[i][0]);
          }
 
          // Maximum total pairs
          let total = N * (N - 1) / 2;
 
          // Array to keep track of components
          let visited = new Array(N + 1).fill(false)
 
          // Loop to count total possible pairs
          for (let i = 0; i < N; i++) {
              if (visited[i] == false) {
                  let cnt = 0;
 
                  dfs(adj, i, visited, cnt);
 
                  // Subtract pairs from
                  // the same connected component
                  total -= (cnt * (cnt - 1) / 2);
              }
          }
          return total / 2;
      }
 
      // Driver code
      let N = 4;
      let edges = [[1, 2],
      [2, 3]];
 
      let result = maxPairs(N, edges);
      document.write(result);
 
     // This code is contributed by Potta Lokesh
  

输出

3

时间复杂度： O(N)
辅助空间： O(N)