弧长公式
数学为与计算相关的不同类型的研究提供了广阔的探索领域。几何也是数学的一个分支,它处理角度、线、线段、点等,并帮助我们确定它们之间的空间关系。它是数学中最古老的部分之一。因为我们有不同种类或类型的几何可以关注。
给定的文章是对弧长、弧和确定弧长的公式的研究。内容通过示例提供了计算弧长的不同方法。并且,还有一些已解决的示例问题可以更好地阐明计算弧长所涉及的公式和方法。
什么是弧长?
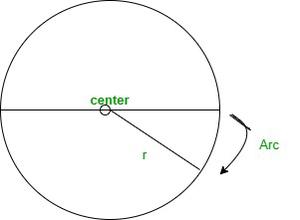
弧长是曲线或圆周的一部分。它是形成弧的曲线部分的距离。形成的所有弧形都是弯曲的,可以沿着曲线的方向覆盖任何距离。
An arc can be defined as a part of a curve or circumference of a circle.
弧长是曲线上两点之间的圆周或距离的一部分。形成弧的两点所对的角称为弧的中心角。
弧长公式
弧长公式是用于计算弧长的公式。确定弧长的公式使用弧的中心角。这些中心角以弧度或度数的形式表示。圆的弧长由 θ 乘以圆的半径计算得出。
在数学上,公式写为
以弧度表示:
Arc length = θ × r
where,
θ is the angle expressed in radian
r is the radius of the circle
学位:
Arc length = θ × (π/180) × r
Where,
θ is the central angle expressed in degree
r is the radius of the circle
如何找到没有圆心角的弧长?
方法一:圆的弧长可以在圆心角未知的情况下,利用圆的半径和扇形面积来确定。不使用圆心角的圆弧长度可以通过给定的方法确定。
- 第 1 步:将给定圆的扇形面积乘以 2。
- 第 2 步:将数字除以半径的平方。中心角将在此步骤中确定。
- 第三步:将得到的圆心角乘以圆的半径,得到弧长。
示例:计算曲线扇形区域的弧长 50cm 2 ,半径测量为 4cm。
解决方案:
Given
The sector area is 50cm2
The radius is 4cm.
Now,
=>sector area×2
=>50×2
=>100
And,
=>100/r2
=>100/4×4
=>6.25
6.25 is the central angle (In radian).
Then,
Arc length= radius×central angle
=>4×6.25
=>25cm
方法二:圆的弧长可以在圆心角未知的情况下,利用圆的半径和弦长来确定。不使用弦长和半径的圆弧长度可以通过给定的方法确定。
- 第 1 步:将给定弦长除以给定半径的两倍。
- 第 2 步:计算所得数字的正弦倒数。
- 步骤 3:乘以数字的正弦倒数得到的结果。在该步骤中,确定中心角。得到的圆心角以弧度表示。
- 第四步:用圆心角乘以半径确定弧长。
示例:计算与长 6cm 的弦相接触且对向 2 弧度的圆心角的曲线的弧长。
解决方案:
Given
The central angle(θ) is 2 radian.
The chord length is 6cm.
Now,
=>central angle/2
=>2/2=1
And,
=>sin(1) = 0.841
And,
=>chord length/(2×0.841)
=>6/1.682 = 3.56
This is the radius of the circle.
Then,
arc length = θ × r
=>arc length = 2×3.56=7.12cm
示例问题
问题1:在半径为4cm、圆心角为2弧度的圆上求曲线的弧长。
解决方案:
Given
The central angle(θ) is 2 radians.
The radius of the circle is 4cm.
Now,
Arc length=θ×r
=>2×4
=>8cm
Hence, the arc length is 8cm.
问题2:在半径为16cm、圆心角为4radians的圆上求曲线的弧长。
解决方案:
Given
The central angle(θ) is 4 radians.
The radius of the circle is 16cm.
Now,
Arc length=θ×r
=>4×16
=>64cm
Hence, the arc length is 8cm.
问题3:计算曲线扇形面积25cm 2的弧长,半径为5cm。
解决方案:
Given
The sector area is 25cm2
The radius is 5cm.
Now,
=>sector area×2
=>25×5
=>125
And,
=>125/r2
=>125/5×5
=>5
5 is the central angle (In radian).
Then,Arc length= radius×central angle
=>4×5
=>20cm
问题4:计算曲线扇形面积40cm 2的弧长,半径为8cm。
解决方案:
Given
The sector area is 40cm2
The radius is 8cm.
Now,
Arc length=sector area×2
=>40×8
=>320
And,
=>320/r2
=>320/8×8
=>5
5 is the central angle (In radian).
Then,
Arc length= radius×central angle
=>8×5
=>40cm
问题 5:计算与 16 厘米长的弦相接触并对着 4 弧度的圆心角的曲线的弧长。
解决方案:
Given
The central angle(θ) is 2radian.
The chord length is 16cm.
Now,
=>central angle/2
=>2/2=1
And,
=>sin(1)=0.841
And,
=>chord length/(2×0.841)
=>16/1.682=9.51cm
This is the radius of the circle.
Then,
arc length =θ×r
=>arc length =2×9.51=19.02cm