问题1.说明图中的哪些三角形对相似。写下您用来回答问题的相似性准则,并以符号形式写下成对的相似三角形:

解决方案:
(i) We have, in ΔABC and ΔPQR,
∠A = ∠P = 60°
∠B = ∠Q = 80°
∠C = ∠R = 40°
By AAA similarity criterion, we have
∴ ΔABC ~ ΔPQR
(ii) We have, in ΔABC and ΔPQR,
Computing the ratios of corresponding sides,
AB/QR = 2.5/5 = 1/2
BC/RP = 2/4 = 1/2
CA/PQ = 3/6 = 1/2
AB/QR = BC/RP = CA/PQ
Now,
By SSS similarity criterion, we have
ΔABC ~ ΔQRP
(iii) We have, in ΔLMP and ΔDEF,
Now,
LM = 2.7, MP = 2, LP = 3, EF = 5, DE = 4, DF = 6
Computing the ratios of the corresponding sides,
MP/DE = 2/4 = 1/2
PL/DF = 3/6 = 1/2
LM/EF = 2.7/5 = 27/50
Since, MP/DE = PL/DF ≠ LM/EF
Therefore, ΔLMP and ΔDEF are not similar.
(iv) In ΔMNL and ΔQPR, it is given,
Computing the ratios of the corresponding sides,
MN/QP = ML/QR = 1/2
And,
∠M = ∠Q = 70°
By SAS similarity criterion
ΔMNL ~ ΔQPR
(v) In ΔABC and ΔDEF, we have,
AB = 2.5, BC = 3, ∠A = 80°, EF = 6, DF = 5, ∠F = 80°
Computing the ratios of the corresponding sides,
AB/DF = 2.5/5 = 1/2
And, BC/EF = 3/6 = 1/2
⇒ ∠B ≠ ∠F
Therefore, ΔABC and ΔDEF are not similar.
(vi) In ΔDEF,
By angle sum property of a triangle,
∠D + ∠E + ∠F = 180°
⇒ 70° + 80° + ∠F = 180°
⇒ ∠F = 180° – 70° – 80°
⇒ ∠F = 30°
Similarly, In ΔPQR,
By angle sum property of a triangle,
∠P + ∠Q + ∠R = 180
⇒ ∠P + 80° + 30° = 180°
⇒ ∠P = 180° – 80° -30°
⇒ ∠P = 70°
Now, in ΔDEF and ΔPQR, we have
∠D = ∠P = 70° [equal corresponding angles]
∠F = ∠Q = 80°
∠F = ∠R = 30°
Therefore,
By AAA similarity criterion,
ΔDEF ~ ΔPQR
问题2。在图中,ΔODC等于1/4ΔOBA,BOC等于125°,CDO等于70°。查找∠DOC,∠DCO和∠OAB。

解决方案:
We know, DOB is a straight line, as given.
Therefore, ∠DOC + ∠COB = 180°
Also,
∠BOC = 125°
⇒ ∠DOC = 180° – 125°
= 55°
In ΔDOC
By angle sum property of a triangle,
∠DCO + ∠CDO + ∠DOC = 180°
∠CDO = 70°
⇒ ∠DCO + 70º + 55º = 180°
⇒ ∠DCO = 55°
Also, given,
ΔODC ∝ ¼ ΔOBA,
Therefore, ΔODC ~ ΔOBA.
Since, we know that the corresponding angles are equal in similar triangles,
Therefore, ∠OAB = ∠OCD
⇒ ∠ OAB = 55°
∠OAB = ∠OCD
∠OAB = 55°
问题3.梯形ABCD与AB ||的对角AC和BD DC在点O处彼此相交。使用两个三角形的相似性准则,表明AO / OC = OB / OD
解决方案:
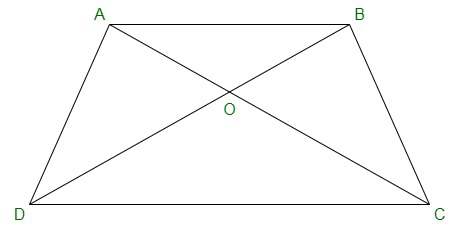
In ΔDOC and ΔBOA,
Since, AB || CD, therefore the alternate interior angles are equal,
That is, ∠CDO = ∠ABO ….(i)
And,
∠DCO = ∠BAO …(ii)
Also, vertically opposite angles are equal;
That is,∠DOC = ∠BOA…(iii)
Hence, by AAA similarity criterion,
ΔDOC ~ ΔBOA [From eq(i) , (ii) and (iii)]
Since the triangles are similar, therefore the corresponding sides are proportional.
DO/BO = OC/OA
⇒OA/OC = OB/OD
Hence, proved.
问题4。在图中,QR / QS = QT / PR,∠1=∠2。证明ΔPQS〜ΔTQR。

解决方案:
We have,
In ΔPQR,
∠PQR = ∠PRQ
That is, PQ = PR .…(i)
Given,
QR/QS = QT/PR
By using eq(i), we obtain,
QR/QS = QT/QP …….(ii)
In ΔPQS and ΔTQR,
QR/QS = QT/QP [By using eq(ii)]
∠Q = ∠Q
By SAS similarity criterion, therefore,
ΔPQS ~ ΔTQR
问题5. S和T是ΔPQR的PR和QR边的点,因此∠P=∠RTS。表明ΔRPQ〜ΔRTS。
解决方案:
We know,
S and T are the points on sides PR and QR of ΔPQR.
And, ∠P = ∠RTS.
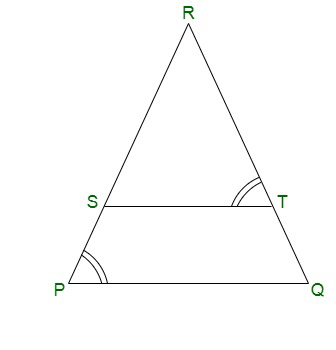
In ΔRPQ and ΔRTS,
Given,
∠RTS = ∠QPS
∠R = ∠R (Common angle)
By AAA similarity criterion,
∴ ΔRPQ ~ ΔRTS
问题6。在图中,如果ΔABE≅ΔACD,则表明ΔADE〜ΔABC。
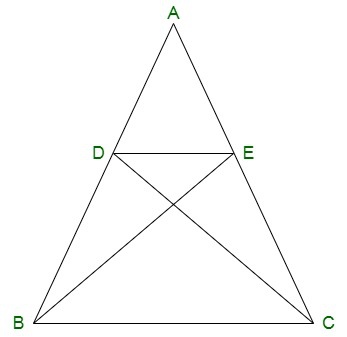
解决方案:
Given, ΔABE ≅ ΔACD.
By CPCT, we have
∴ AB = AC ……….(i)
And, AD = AE …………(ii)
In ΔADE and ΔABC,
On dividing the eq.(ii) by eq(i), we obtain,
AD/AB = AE/AC
Also, by common angle,
∠A = ∠A
By SAS similarity criterion, we have,
∴ ΔADE ~ ΔABC
问题7.在图中,ΔABC的高度AD和CE在点P处相交。

(i)ΔAEP〜ΔCDP
(ii)ΔABD〜ΔCBE
(iii)ΔAEP〜ΔADB
(iv)ΔPDC〜ΔBEC
解决方案:
Given, altitudes AD and CE of ΔABC intersect each other at the point P.
(i) In ΔAEP and ΔCDP,
Since, both of the angles are 90° each.
∠AEP = ∠CDP
Since,
∠APE = ∠CPD (Vertically opposite angles)
By AA similarity criterion, we have,
ΔAEP ~ ΔCDP
(ii) In ΔABD and ΔCBE,
Since, both of the angles are 90° each.
∠ADB = ∠CEB
∠ABD = ∠CBE (Common Angles)
By AA similarity criterion, we have,
ΔABD ~ ΔCBE
(iii) In ΔAEP and ΔADB,
Since, both of the angles are 90° each.
∠AEP = ∠ADB
∠PAE = ∠DAB (Common angles)
By AA similarity criterion, we have,
ΔAEP ~ ΔADB
(iv) In ΔPDC and ΔBEC,
Since, both of the angles are 90° each.
∠PDC = ∠BEC
∠PCD = ∠BCE (Common angles)
By AA similarity criterion, we have,
ΔPDC ~ ΔBEC
问题8. E是平行四边形ABCD产生的AD侧的点,BE在F处与CD相交。表明ΔABE〜ΔCFB。
解决方案:
Given,

E is a point on the side AD produced of a parallelogram ABCD and BE intersects CD at F.
Now,
In ΔABE and ΔCFB,
Since, the opposite angles of a parallelogram are equal, we have,
∠A = ∠C
Also, since AE || BC
∠AEB = ∠CBF (Alternate interior angles)
By AA similarity criterion,
∴ ΔABE ~ ΔCFB
问题9。在图中,ABC和AMP是两个直角三角形,分别与B和M成直角,证明:

(i)ΔABC〜ΔAMP
(ii)CA / PA = BC / MP
解决方案:
Given,
ABC and AMP are two right triangles, right-angled at B and M respectively.
(i) In ΔABC and ΔAMP, we have,
∠CAB = ∠MAP (common angles)
Since, both of the angles are equivalent to 90°
∠ABC = ∠AMP
By AA similarity criterion, we have,
∴ ΔABC ~ ΔAMP
(ii) As, ΔABC ~ ΔAMP (AA similarity criterion) [proved in part (i)]
Therefore, we know,
If two triangles are similar then the corresponding sides are always equal,
Hence, CA/PA = BC/MP
问题10:CD和GH分别是∠ACB和∠EGF的等分线,使得D和H分别位于ΔABC和ΔEFG的AB和FE侧。如果ΔABC〜ΔFEG,则表明:
(i)CD / GH = AC / FG
(ii)ΔDCB〜ΔHGE
(iii)ΔDCA〜ΔHGF
解决方案:
Given,
CD and GH are respectively the bisectors of ∠ACB and ∠EGF such that D and H lie on sides AB and FE of ΔABC and ΔEFG respectively.
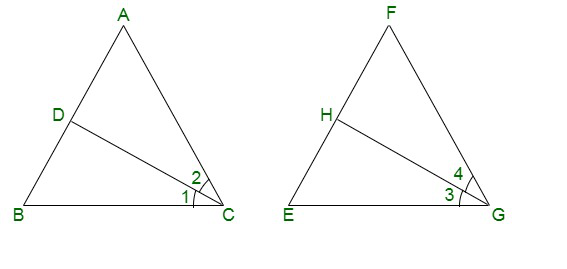
(i) We have,
ΔABC ~ ΔFEG.
Therefore,
∠A = ∠F, ∠B = ∠E, and ∠ACB = ∠FGE
Now, since,
∠ACB = ∠FGE
∴ ∠ACD = ∠FGH (Angle bisector)
And, ∠DCB = ∠HGE
In ΔACD and ΔFGH,
∠A = ∠F
∠ACD = ∠FGH
By AA similarity criterion, we have,
∴ ΔACD ~ ΔFGH
⇒CD/GH = AC/FG
(ii) In ΔDCB and ΔHGE,
Proved in part (i)
∠DCB = ∠HGE
∠B = ∠E
By AA similarity criterion,
∴ ΔDCB ~ ΔHGE
(iii) In ΔDCA and ΔHGF,
Since, we have already proved,
∠ACD = ∠FGH
∠A = ∠F
By AA similarity criterion,
∴ ΔDCA ~ ΔHGF