问题1.证明在直角三角形中,斜边是最长的边。
解决方案:
Given: Right angle triangle intersect ∠B=90°
To show: AC>AB and AC>BC
Solution:∠A+∠B+∠C=180° —————-[angle sum property]
∠A+90°+∠C=180°
∠A+∠C=180°=90°
∠A+∠C=90°
∴∠A<90° and ∠C<90°
BC ∴Hypotenuse is the longest side.
问题2.在图7.48中,ΔABC的边AB和AC分别延伸到点P和Q。此外,∠PBC<∠QCB。显示AC> AB。
解决方案:
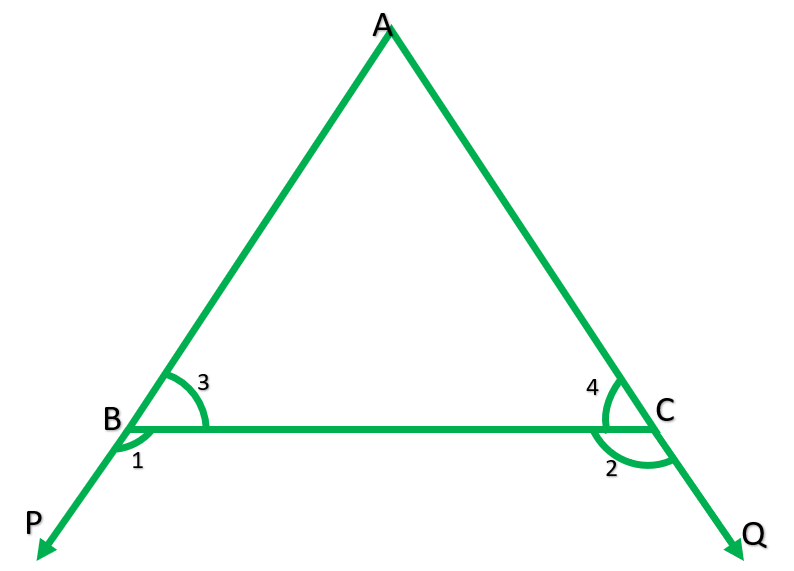
Given : ∠PBC < ∠QCB LET this be [∠1 < ∠2]
To Show : AD < BC
Solution: ∠1 < ∠2 ——–[given]
-∠1 > -∠2
180-∠1>180-∠2
∠3>∠4 ———[linear pair]
In ∆ABC,
∠3>∠4
AC>AB
问题3.在图7.49中,, B <∠A和∠C<∠D。证明AD
解决方案:

Given: ∠B < ∠A and ∠C < ∠D
To show: AD Solution: In ∆BOA ∠B < ∠A ∴AO In ∆COD ∠C < ∠D ∴OD Adding 1 and 2 AO+OD+ AD
问题4. AB和CD分别是四边形ABCD的最小和最长边(见图7.50)。显示∠A>∠C和∠B>∠D。
解决方案:

Given: AB is smaller side
CD is longest side
To show: ∠A > ∠C and ∠B > ∠D.
Solution : In ∆ABC
BC>AB
∠1>∠2 ———-[angle opposite to greater side is greatest]-1
In ∆ABC
CD>AD
∠3>∠4 ———[ angle opposite to greater side is greatest]-2
Adding 1 and 2
∠1+∠2+∠3+∠4
∠A>∠C
ii) In ∆ABD
AD>AB
∠5>∠6 ——————-[ angle opposite to greater side is greatest]-3
In ∆BCD
CD>BC
∠7>∠8 ——————-[ angle opposite to greater side is greatest]-4
Adding 3 and 4
∠5+∠6+∠7+∠8
∠B > ∠D
问题5。在图7.51中,PR> PQ和PS对分∠QPR。证明∠PSR>∠PSQ。
解决方案:

Given: PR>PQ
PS is angle bisects ∠QPR
To show: ∠PSR > ∠PSQ
Solution: PR>PQ
∴∠3+∠4 ————[angle opposite to grater side is larger]
∠3+∠1+x=180° ————-[angle sum property of ∆]
∠3=180°-∠1-x ————1
In ∆PSR
4+∠2+x=180° ————-[angle sum property of ∆]
∠4=180°-∠2-x ————2
Because ∠3>∠4
180°-∠1-x >180°-∠2-x
-∠1>-∠2
∠1<∠2
∠PSQ<∠PSR OR ∠PSR>∠PSQ
问题6.显示从给定点不在其上的所有线段中,垂直线段最短
解决方案:

Given: Let P be any point not lying on a line L.
PM ⊥ L
Now, ∠ is any point another than M lying on line=L
In ∆PMN
∠M90° ———-[ PM ⊥ L]
∠L<90° ——-[∴∠M90° ∠L<90° ∠L<90°]
∠L<∠M
AM