问题25.证明矩阵A = 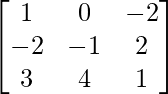 满足方程A 3 – A 2 – 3A – I 3 =0。因此,找到A -1 。
满足方程A 3 – A 2 – 3A – I 3 =0。因此,找到A -1 。
解决方案:
Here, A = 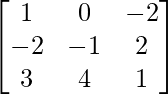
A2 = 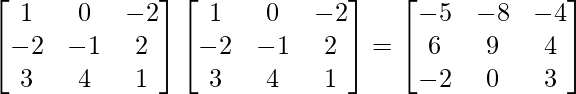
A3 = 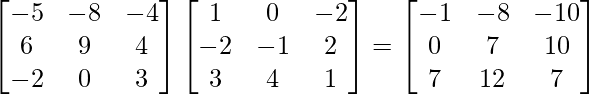
Now A3 – A2 – 3A – I3 = 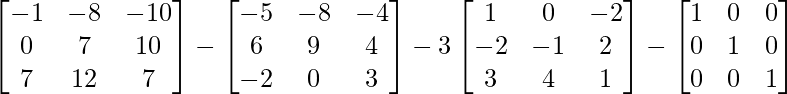
=
= 
So, A3 – A2 – 3A – I3 = 0
⇒ A-1(AAA) – A-1(AA) – 3A-1A – A-1I = 0
⇒ A2 – A – 3I – A-1 = 0
⇒ A-1 = A2 – A – 3I
= 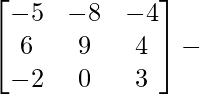
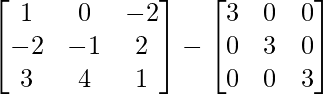
Therefore, A-1 =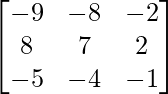
问题26.如果A = 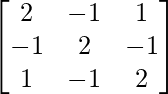 。验证A 3 – 6A 2 + 9A – 4I = O,从而找到A -1 。
。验证A 3 – 6A 2 + 9A – 4I = O,从而找到A -1 。
解决方案:
Here, A = 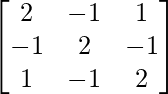
A2 = 
=
= 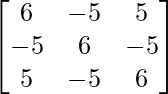
A3 = A2A = 
= 
= 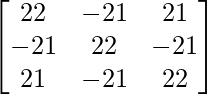
Now, A3 – 6A2 + 9A – 4I
= 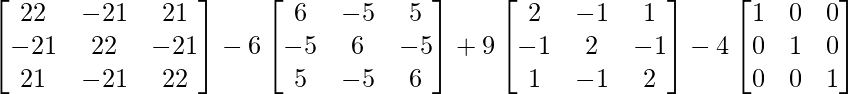
= 
= 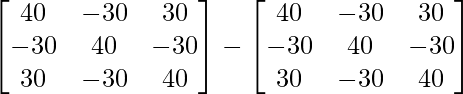
= 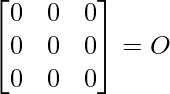
So, A3 – 6A2 + 9A – 4I = O
Multiplying both side by A-1
⇒ A-1(AAA) – 6A-1(AA) 9A-1A – 4A-1I = O
⇒ AAI – 6AI + 9I = 4A-1
⇒ 4A-1 = A2I – 6AI + 9I
=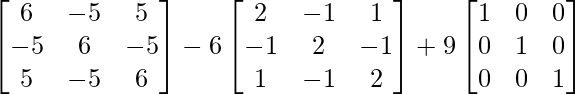
=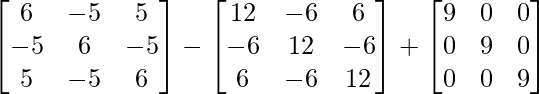
=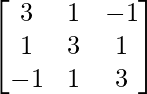
⇒ A-1 = 
问题27.如果A = 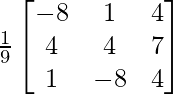 证明A -1 = A T。
证明A -1 = A T。
解决方案:
Here, A = 
AT = 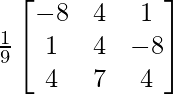
Now, Finding A-1
|A| = 1/9[-8(16 + 56) – 1(16 – 7) + 4(-32 – 4)]
= -81
Therefore, inverse of A exists
Cofactors of A are:
C11 = 72 C12 = -9 C13 = -36
C21 = -36 C22 = -36 C23 = -63
C31 = -9 C32 = 72 C33 = -36
adj A = 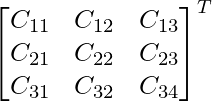
=
=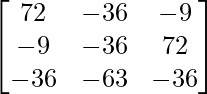
A-1 = 1/|A|. adj A
Hence, A-1 = 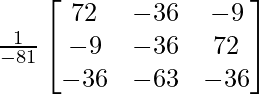
= 
= AT
Hence Proved
问题28.如果A = 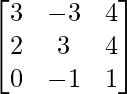 ,表明A -1 = A 3 。
,表明A -1 = A 3 。
解决方案:
Here, A = 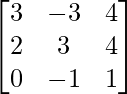
|A| = 3(-3 + 4) + 3(2 – 0) + 4(-2 – 0)
= 3 + 6 – 8
= 1
Therefore, inverse of A exists
Cofactors of A are:
C11 = 1 C12 = -2 C13 = -2
C21 = -1 C22 = 3 C23 = 3
C31 = 0 C32 = -4 C33 = -3
adj A =
A-1= 1/|A|. adj A
= 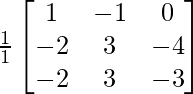
Now, A2 = 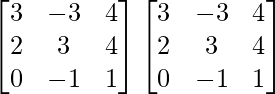
=
A3 = 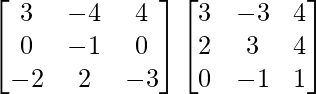
=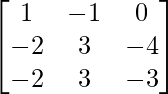
= A-1
Hence Proved
问题29:如果A = 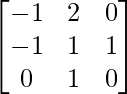 ,表明A 2 = A -1 。
,表明A 2 = A -1 。
解决方案:
Here, A = 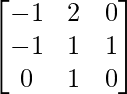
LHS = A2
= 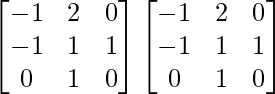
= 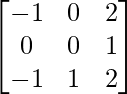
|A| = -1(1 – 0) – 2(-1 – 0) + 0
= 1
Therefore, inverse of A exists
Cofactors of A are:
C11 = -1 C12 = 0 C13 = -1
C21 = 0 C22 = 0 C23 = 1
C31 = 21 C32 = 1 C33 = 1
adj A = 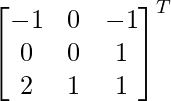
=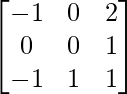
A-1 = 1/|A|. adj A
Hence, A-1 = 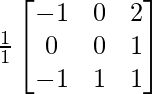
=
= A2
Hence Proved
问题30.求解矩阵方程 ,其中X是2×2矩阵。
,其中X是2×2矩阵。
解决方案:
We have, 
Let A =  and B =
and B = 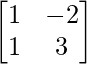
So. AX = B
⇒ X = A-1B
Now, |A| = 5 – 4 = 1
Co factors of A are:
C11 = 1 C12 = -1
C21 = -4 C22 = 5
adj A = 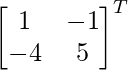
=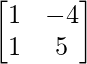
A-1 = 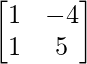
Therefore, X = 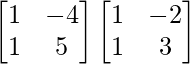
X = 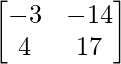
问题31.找到满足矩阵方程的矩阵X: 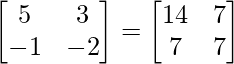 。
。
解决方案:
We have, 
Let A = and B =
and B = 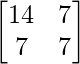
So, XA = B
XAA-1 = BA-1
XI = BA-1 ………..(i)
Now, |A| = -7
Co factors of A are:
C11 = -2 C12 = 1
C21 = -3 C22 = 5
adj A = 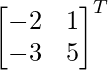
=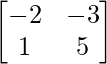
A-1 = 1/|A|.adj (A)
=
Therefore, X = 
=
X = 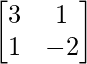
问题32.找到矩阵X,其矩阵为:  X
X 
解决方案:
Let, A = 
B = 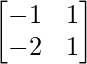
C = 
Then the given equation becomes
A × B = C
⇒ X = A-1CB-1
Now |A| = 35 -14 = 21
|B| = -1 + 2 = 1
A-1 = adj (A)/|A| = 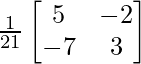
B-1 = adj (B)/|A| = 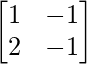
X = A-1 CB-1 = 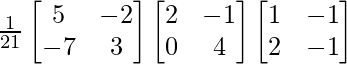
= 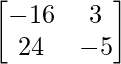
问题33.找到满足方程式的矩阵X: 
解决方案:
Let A =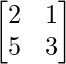 B =
B = 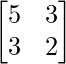
AXB = I
X = A-1B-1
|A| = 6 – 5 = 1
|B| = 10 – 9 = 1
A-1 = adj A /|A| = 
B-1 = adj B/|B| = 
X = 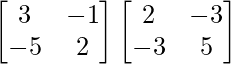
= 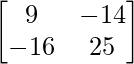
问题34.如果A =  ,找到A -1并证明A 2 – 4A – 5I =O。
,找到A -1并证明A 2 – 4A – 5I =O。
解决方案:
Here, A = 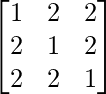
A2 = 
= 
Now, A2 + 4A – 5I
= 
= 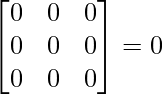
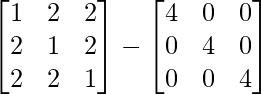
Now, A2 – 4A – 5I = O
⇒ A-1AA – 4A-1A – 5A-1I = O
⇒ 5A-1 = [A – 4I]
= 
= 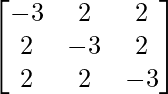
A-1 = 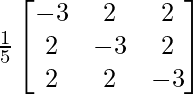
问题35.如果A是n阶方阵,则证明| A adj A | = | A | n 。
解决方案:
Given, |A adj A| = |A|n
Taking LHS = |A Adj A|
= |A| |Adj A|
= |A| |A|n-1
= |A|n-1+1
= |A|n = RHS
Hence Proved
问题36.如果A -1 =  和B =
和B =  ,找到(AB) -1 。
,找到(AB) -1 。
解决方案:
Here, B = 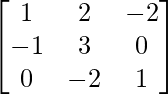
|B| = 1(3 – 0) – 2(-1 – 0) – 2(2 – 0)
= 3 + 2 – 4 = 1
Therefore, inverse of B exists
Cofactors of B are:
C11 = 3 C12 = 1 C13 = 2
C21 = 2 C22 = 1 C23 = 2
C31 = 6 C32 = 2 C33 = 5
adj A = 
= 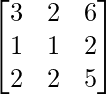
Therefore,
B-1 = 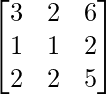
Hence, (AB)-1 = B-1A-1
= 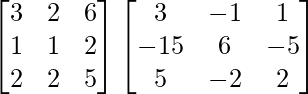
= 
问题37.如果A =  ,找到(A T ) -1 。
,找到(A T ) -1 。
解决方案:
Assuming B = AT = 
|B| = 1(-1 – 8) – 0 – 2(-8 + 3)
= -9 + 10 = 1
Therefore, inverse of B exists
Cofactors of B are:
C11 = -9 C12 = 8 C13 = -5
C21 = -8 C22 = 7 C23 = -4
C31 = -2 C32 = 2 C33 = -1
adj B = 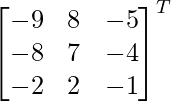
=
B-1 = 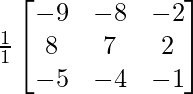
or (AT)-1 = 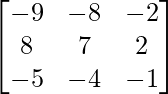
问题38.求矩阵A的伴随 因此表明A(adj A)= | A | I 3 。
因此表明A(adj A)= | A | I 3 。
解决方案:
Here, A = 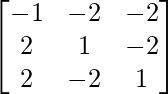
|A| = -1(1 – 4) – 2(2 + 4) – 2(-4 – 2)
= 3 + 12 + 12 = 27
Therefore, inverse of A exists
Cofactors of A are:
C11 = -3 C12 = -6 C13 = -6
C21 = 6 C22 = 3 C23 = -6
C31 = 6 C32 = -6 C33 = 3
adj A = 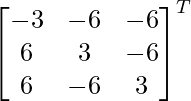
= 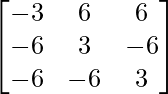
A (adj A) = 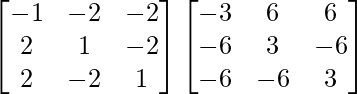
= 
or A (adj A) = 27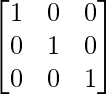
Hence, A (adj A) = |A|I3
Hence Proved
问题39.如果A = 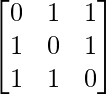 ,A -1 ,表明A -1 = 1/2(A 2 – 3I)。
,A -1 ,表明A -1 = 1/2(A 2 – 3I)。
解决方案:
Here, A = 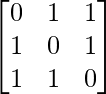
|A| = 0 – 1(0 – 1) + 1(1 – 0)
= 1 + 1 = 2
Therefore, inverse of A exists
Cofactors of A are:
C11 = -1 C12 = 1 C13 = 1
C21 = 1 C22 = -1 C23 = 1
C31 = 1 C32 = 1 C33 = -1
adj A = 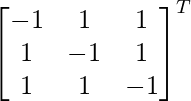
= 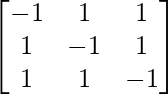
A-1 = 1/|A|. adj A
Hence, A-1 = 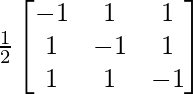
Now, A2 – 3I = 
=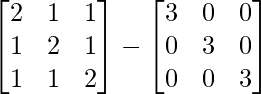
=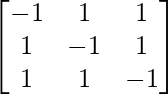
Hence, A-1 = 1/2(A2 – 3I)
Hence Proved