8类NCERT解决方案-第8章比较数量–练习8.3

问题1.计算金额和复利

(i)10,800卢比，有效期3年，收费12 $\mathbf{\frac{1}{2}}$每年％复利。

(ii)18,000卢比/ 2 $\mathbf{\frac{1}{2}}$年，每年以10％的比例递增。

(iii)62,500卢比换1 $\mathbf{\frac{1}{2}}$每年8％，每半年复利一次。

(iv)一年为8,000卢比，每年9％，每半年复利一次。

(v)一年为10,000卢比，每年8％，每半年复利一次。

解决方案：

(i) Given values are,

P = Rs 10,800

R = 12 $\frac{1}{2}$ % per annum = $\frac{25}{2}$ %

T = 3 Years

As it is compounded annually then, n = 3 times.

We have,

A = P (1 + $\mathbf{\frac{R}{100}}$)ⁿ

A = 10,800 (1+ $\frac{25}{2*100}$)³ 

A = 10,800 (1+ $\frac{1}{8}$)³

A = 10,800 ($\frac{9}{8}$)³

A = Rs 15,377.34

CI = A – P

CI = 15,377.34 – 10,800

CI = Rs 4,577.34

Hence, the amount = Rs 15,377.34 and 

Compound interest = Rs 4,577.34

(ii) Given values are,

P = Rs 18,000

R = 10 % per annum 

T = 2$\frac{1}{2}$ Years

As it is compounded annually then, n = 2$\frac{1}{2}$ times.

We have,

A = P (1 + $\mathbf{\frac{R}{100}}$)ⁿ

A = 18,000 (1+ $\frac{10}{100}$)^2½

What we will do here is Firstly we know 2$\frac{1}{2}$ Years is 2 years and 6 months which can be calculated by first calculating the amount to 2 years using CI formula and then calculating the simple interest by using SI formula.

The amount for 2 years has to be calculated : 

A = 18,000 (1+ $\frac{1}{10}$)²

A = 18,000 ($\frac{11}{10}$)²

A = Rs 21,780

CI = A – P

CI = 21,780 – 18,000

CI = Rs 3,780

Now, The amount for $\frac{1}{2}$ year has to be calculated: 

New P is equal to the amount after 2 Years. Hence, 

P = Rs 21,780

R = 10 % per annum 

T = $\frac{1}{2}$ year

SI = $\frac{PRT}{100}$

SI = $\frac{21,780 × 10 × \frac{1}{2}}{100}$

SI = $\frac{21,780 × 10 × 1}{200}$

SI = Rs 1,089

Hence, the Total amount = A + SI

                                           = 21,780 + 1,809

                                           = Rs 22,869  

Total compound interest = CI + SI

                                          = 3,780 + 1,809

                                          = Rs 4,869   

(iii) Given values are,

P = Rs 62,500

R = 8 % per annum hence 4% Half Yearly

T = 1$\frac{1}{2}$ Years

As it is compounded Half yearly then, n = 3 times. (1$\frac{1}{2}$ Years contains 3 half years)

We have,

A = P (1 + $\mathbf{\frac{R}{100}}$)ⁿ

A = 62,500 (1+ $\frac{4}{100}$)³

A = 62,500 (1+ $\frac{1}{25}$)³

A = 62,500 ($\frac{26}{25}$)³

A = Rs 70,304

CI = A – P

CI = 70,304 – 62,500

CI = Rs 7,804

Hence, the amount = Rs 70,304 and

Compound interest = Rs 7,804

(iv) Given values are,

P = Rs 8,000

R = 9 % per annum hence, $\frac{9}{2}$ % Half Yearly

T = 1 Year

As it is compounded Half yearly then, n = 2 times. (1 Year contains 2 half years)

We have,

A = P (1 + $\mathbf{\frac{R}{100}}$)ⁿ

A = 8,000 (1+ $\frac{9}{2*100}$)²

A = 8,000 (1+ $\frac{9}{200}$)²

A = 8,000 ($\frac{209}{200}$)²

A = Rs 8,736.20

CI = A – P

CI = 8,736.20 – 8,000

CI = Rs 736.20

Hence, the amount = Rs 8,736.20 and

Compound interest = Rs 736.20

(v) Given values are,

P = Rs 10,000

R = 8 % per annum  hence, 4% Half Yearly

T = 1 Year

As it is compounded Half yearly then, n = 2 times. (1 Year contains 2 half years)

We have,

A = P (1+  $\mathbf{\frac{R}{100}}$)ⁿ

A = 10,000 (1+ ($\frac{4}{100}$))²

A =10,000 (1+ ($\frac{1}{25}$))²

A = 10,000 ($\frac{26}{25}$)²

A = Rs 10,816

CI = A – P

CI = 10,816- 10,000

CI = Rs 816

Hence, the amount = Rs 10,816 and

Compound interest = Rs 816

问题2. Kamala向银行借了26,400卢比，以每年15％的复合年利率购买踏板车。她将在2年零4个月末偿还多少贷款清算贷款?

解决方案：

Here, Given values are,

P = Rs 26,400

R = 15 % per annum 

T = 2 Years and 4 months, which is 2 $\frac{1}{3}$ years

As it is compounded annually then, n = 2$\frac{1}{3}$ times

We have,

A = P (1 + $\mathbf{\frac{R}{100}}$)ⁿ

A = 26,400 (1 + ($\frac{15}{100}$)^2(1/3)

What we will do here is Firstly 2 years and 4 months which can be calculated by first calculating the amount to 2 years using CI formula and then calculating the simple interest by using SI formula.

The amount for 2 years has to be calculated:

A = 26,400 (1+ ($\frac{15}{100}$)²

A = 26,400 (1+ ($\frac{3}{20}$)²

 A = 26,400 ($\frac{23}{20}$)²

A = Rs 34,914

Now, The amount for (1/3) year (4 months) has to be calculated :

New P is equal to the amount after 2 Years. Hence,

P = Rs 34,914

R = 15 % per annum 

T = $\frac{1}{3}$ year

SI = $\frac{P × R × T}{100}$

SI = $\frac{(34,914 × 15 × (1/3)}{100}$

SI = $\frac{(34,914 × 15 × 1)}{300}$

SI = 1,745.70

Hence, the Total amount = A + SI

                                       = 34,914 + 1,745.70

                                       = Rs 36,659.70

Hence, the amount to be paid by Kamla = ₹ 36,659.70

问题3. Fabina以单利的利率借入12,500卢比，年利率为12年，为期3年，Radha在同一时期以每年10％的利率借入相同金额，每年复利。谁支付的利息更高，利息多少?

解决方案：

Let’s see each case 

Fabina Case: at simple interest

P = 12,500

R = 12% per annum  

T = 3 Years

SI = $\frac{(P × R × T)}{100}$

SI = $\frac{(12,500 × 12 × 3)}{100}$

SI = Rs 4,500

Radha Case: at compound interest

P = 12,500

R = 10% per annum  

T = 3 Years

As it is compounded annually then, n = 3 times

We have,

A = P (1 + $\mathbf{\frac{R}{100}}$)ⁿ

A = 12,500 (1 + ($\frac{10}{100}$))³

A =12,500 (1 + $\frac{1}{10}$)³

A = 12,500 ($\frac{11}{10}$)³

A = Rs 16,637.5

CI = A – P

CI = 16,637.5 – 12,500

CI = 4,137.5

Clearly we can see that Fabina paid more interest, and she paid

4,500 – 4,137.5 = Rs 362.5 more than Radha

问题4：我以每年6％的单利从Jamshed借了12,000卢比，为期2年。如果我以每年6％的复利利率借入这笔款项，我还需要支付额外的金额吗?

解决方案：

Lets see each case First

At simple interest

P = 12,000

R = 6% per annum  

T = 2 Years

SI = $\frac{(P × R × T)}{100}$

SI = $\frac{(12,000 × 6 × 2)}{100}$

SI = Rs 1,440

At compound interest

P = 12,000

R = 6% per annum  

T = 2 Years

As it is compounded annually then, n = 2 times

We have,

A = P (1 + $\mathbf{\frac{R}{100}}$)ⁿ

A = 12,000 (1+ ($\frac{6}{100}$))²

A =12,000 (1+ ($\frac{3}{50}$))²

A = 12,000 ($\frac{53}{50}$)²

A = Rs 13,483.2 

CI = A – P

CI = 13,483.2 – 12,000

CI = 1,483.2

Clearly we can see that,

1,483.2 – 1,440 = Rs 43.2

Hence, the extra amount to be paid = ₹ 43.20

问题5. Vasudevan投资了60,000卢比，年利率为12％，每半年复利一次。他会得到多少

(a)6个月后?

(b)一年后?

解决方案：

Let’s see each case 

(a) 

P = 60,000

R = 12% per annum (6% Half yearly)

T = 6 Months

As it is compounded Half Yearly then, n = 1 times (as 6 months is 1 half year)

We have,

A = P (1 + $\mathbf{\frac{R}{100}}$)ⁿ

A =60,000 (1+ ($\frac{6}{100}$))¹

A =60,000 (1+ ($\frac{3}{50}$))¹

A = 60,000 ($\frac{53}{25}$)¹

A = Rs 63,600

He would get Rs 63,600 after 6 Months.

(b)

P = 60,000

R = 12% per annum (6% Half yearly)

T = 1 Year

As it is compounded Half Yearly then, n = 2 times (as 1 Year is 2 half year)

We have,

A = P (1 + $\mathbf{\frac{R}{100}}$)ⁿ

A = 60,000 (1+ ($\frac{6}{100}$))²

A = 60,000 (1+ ($\frac{3}{50}$))²

A = 60000 ($\frac{53}{25}$)²

A = Rs 67,416

He would get Rs 67,416 after 1 Year.

问题6.阿里夫(Arif)从银行借了80,000卢比。如果利率为每年10％，请找出他在1后将要支付的金额之差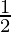$\frac{1}{2}$年，如果利息是

(a)每年复利。

(b)每半年复利一次。

解决方案：

Let’s see each case 

(a) Compounded Annually

P = 80,000

R = 10% per annum

T = 1$\frac{1}{2}$ Year

As it is compounded annually then, n = 1 $\frac{1}{2}$ times

We have,

A = P (1 + $\mathbf{\frac{R}{100}}$)ⁿ

A = 80,000 (1 + ($\frac{10}{100}$)^1½

What we will do here is Firstly we know 1$\frac{1}{2}$ Years is 1 year and 6 months which can be calculated by first calculating the amount to 1 year using CI formula and then calculating the simple interest by using SI formula.

The amount for 1 years has to be calculated :

A = 80,000 (1+ ($\frac{10}{100}$))¹

A = 80,000 (1+ ($\frac{1}{10}$)¹

A = 80,000 ($\frac{11}{10}$)¹

A = Rs 88,000

Now, The amount for $\frac{1}{2}$ Year (6 months) has to be calculated :

New P is equal to the amount after 1 Year. Hence,

P = Rs 88,000

R = 10 % per annum

T =$\frac{1}{2}$ Year

SI = $\frac{(P × R × T)}{ 100}$

SI = $\frac{(88,000 × 10 × \frac{1}{2})}{100}$

SI = $\frac{(88,000 × 10 × 1)}{200}$

SI = 4,400

Hence, the Total amount = A + SI

                                        = 88,000 + 4,400

                                        = Rs 92,400

(b) Compounded Half-yearly

P = 80,000

R = 10% per annum (5 % Half Yearly)

T = 1$\frac{1}{2}$ Year

As it is compounded annually then, n = 3 times (as 1$\frac{1}{2}$ Year is 3 half year)

We have,

A = P (1 + $\mathbf{\frac{R}{100}}$)ⁿ

A = 80,000 (1+ ($\frac{5}{100}$)³

A = 80,000 (1+ ($\frac{1}{20}$)³

A = 80,000 ($\frac{21}{20}$)³

A = Rs 92,610

Hence, the Total amount = Rs 92,610

问题7.玛丽亚(Maria)在一家公司投资了8,000卢比。她将按每年5％的复合年利率支付利息。找

(a)第二年末根据她的名字贷记的数额。

(b)第三年的利息。

解决方案：

Let’s see each case 

Here, 

P = 8,000

R = 5% Per annum

(a) The amount credited against Maria’s name at the end of the second year.

T = 2 Year

As it is compounded annually then, n = 2 times

We have,

A = P (1 + $\mathbf{\frac{R}{100}}$)ⁿ

A = 8,000 (1+ ($\frac{5}{100}$))²

A = 8,000 (1+ ($\frac{1}{20}$))²

A = 8,000 ($\frac{21}{20}$)²

A = Rs 8,820

Hence, the amount credited against Maria’s name at the end of the second year = Rs 8,820

(b) The interest for the 3rd year.

T = 3 Year

As it is compounded annually then, n = 3 times

We have,

A = P (1+ $\mathbf{\frac{R}{100}}$)ⁿ

A = 8,000 (1+ ($\frac{5}{100}$))³

A = 8,000 (1+ ($\frac{1}{20}$))³

A = 8,000 ($\frac{21}{20}$)³

A = Rs 9,261

The interest for the 3rd year = Amount after 3 years – Amount after 2 Years

                                                 = 9,261 – 8,820

                                                 = Rs 441

Another Solution for (b)

As we can calculate interest of 3^rd year by having 2^nd Year Amount as P.

P = 8,820

R = 5% per annum

T = 1 Year (2^nd to 3^rd year)

SI = $\frac{(P × R × T)}{100}$

SI = $\frac{(8,820 × 5 × 1)}{100}$

SI = Rs 441

The interest for the 3rd year = Rs 441

问题8.找出1的金额和10,000卢比的复利$\frac{1}{2}$年，每年10％，每半年复利一次。这种利息是否会超过他每年增加的利息呢?

解决方案：

Let’s see each cases

Compounded Annually

P = 10,000

R = 10% per annum

T = 1$\frac{1}{2}$ Year

As it is compounded annually then, n = 1 $\frac{1}{2}$ times

We have,

A = P (1 + $\mathbf{\frac{R}{100}}$)ⁿ

A = 10,000 (1 + ($\frac{10}{100}$)^1½

What we will do here is Firstly we know 1½ Years is 1 year and 6 months which can be calculated by first calculating the amount to 1 year using CI formula and then calculating the simple interest by using SI formula.

The amount for 1 year has to be calculated:

A = 10,000 (1 + $\frac{10}{100}$)¹

A = 10,000 (1+ $\frac{1}{10}$)¹

A = 10,000 ($\frac{11}{10}$)¹

A = Rs 11,000

CI = A – P

CI = 11,000-10,000

CI = 1,000

Now, The amount for $\frac{1}{2}$ Year (6 months) has to be calculated :

New P is equal to the amount after 1 Year. Hence,

P = Rs 11,000

R = 10 % per annum

T =$\frac{1}{2}$ Year

SI = $\frac{(P × R × T)}{100}$

SI = $\frac{(11,000 × 10 × \frac{1}{2})}{100}$

SI = $\frac{11,000 × 10}{200}$

SI = 550

Hence, the Total Interest (compounded annually)= CI + SI

                                       = 1,000 + 550

                                       = Rs 1,550

Compounded Half-yearly

P = 10,000

R = 10% per annum (5 % Half Yearly)

T = 1$\frac{1}{2}$ Year

As it is compounded annually then, n = 3 times (as 1$\frac{1}{2}$ Year is 3 half year)

We have,

A = P (1 + $\mathbf{\frac{R}{100}}$)ⁿ

A = 10,000 (1 + ($\frac{5}{100}$)³

A = 10,000 (1+ $\frac{1}{20}$)³

A = 10,000 ($\frac{21}{20}$)³

A = Rs 11,576.25

CI = A – P

CI = 11,576.25 – 10,000

CI = 1,576.25

Hence, the Total Interest (compounded Half Yearly) = Rs 11576.25

Difference between the two interests = 1,576.25 – 1,550 = Rs 26.25

Hence, the interest will be Rs 26.25 more when compounded half-yearly than the interest when compounded annually.

问题9.如果Ram在12个月内给了18个月，请找出Ram可获得的Rs 4096金额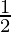$\frac{1}{2}$每年％，每半年复利一次。

解决方案：

Let’s see this case

P = Rs 4,096

R = 12 $\frac{1}{2}$ % per annum ($\frac{25}{4}$ % Half yearly)

T = 18 Months = 1$\frac{1}{2}$ Year

As it is compounded Half yearly then, n = 3 Times

We have,

A = P (1 + $\mathbf{\frac{R}{100}}$)ⁿ

A = 4,096 (1+ ($\frac{\frac{25}{4}}{100}$)³

A = 4,096 (1+ $\frac{25}{400}$)³

A = 4,096 (1+ ($\frac{1}{16}$)³

A = 4,096 ($\frac{17}{16}$)³

A = Rs 4,913

Ram will get the amount = Rs 4,913

问题10：一个地方的人口在2003年以每年5％的速度增加到54,000

(a)找到2001年的人口。

(b)2005年的人口是多少?

解决方案：

Here,

P = 54,000 (in 2003)

R = 5% per annum

(a) Population in 2001

T = 2 Years (back)

n = 2

Population in 2003 = Population in 2001 (1 + $\mathbf{\frac{R}{100}}$)ⁿ

54,000 = P₁ (1+($\frac{5}{100}$))²

54,000 = P1 ($\frac{21}{20}$)²

54,000 = P1 ($\frac{441}{400}$)

P1 = 54,000 ($\frac{441}{400}$)

P1 = 48,979.59

P1 = 48,980 (approx.).

Population in 2001 was 48,980 (approx.).

(b) Population in 2005

T = 2 Years

n = 2

We have,

A = P (1 + $\mathbf{\frac{R}{100}}$)ⁿ

A = 54,000 (1+ $\frac{5}{100}$)²

A = 54,000 (1+ ($\frac{1}{20}$)²

A = 54,000 ($\frac{21}{20}$)²

A = 59,535

Population in 2005 will be 59,535

问题11：在实验室中，某个实验中的细菌数量以每小时2.5％的速度增加。如果最初计数为5，06,000，则在2小时结束时找到细菌。

解决方案：

Here,

P = 5,06,000

R = 2.5% per hour

T = 2 hours

We have,

A = P (1 + $\mathbf{\frac{R}{100}}$)ⁿ

A = 5,06,000 (1+ $\frac{2.5}{100}$)²

A = 5,06,000 (1+ $\frac{25}{1000}$)²

A = 5,06,000 (1+ $\frac{1}{40}$)²

A = 5,06,000 ($\frac{41}{40}$)²

A = 5,31,616.25

A = 5,31,616 (approx.)

Bacteria at the end of 2 hours = 5,31,616 (approx.)

问题12.一辆踏板车的价格为42,000卢比。它的价值每年贬值8％。一年后找到它的价值。

解决方案：

Here,

P = 42,000

R = 8% per annum (depreciated)

T = 1 Year

We have,

A = P (1 + $\mathbf{\frac{R}{100}}$)ⁿ

A = 42,000 (1- $\frac{8}{100}$)¹ (negative sign because the price is reduced)

A = 42,000 (1- ($\frac{2}{25}$)¹

A = 42,000 ($\frac{23}{25}$)¹

A = Rs 38,640

The value of scooter after one year will be = Rs 38,640