问题1.在数字行上代表这些数字吗?
(i)7/4(ii)-5/6
解决方案:
(i)在数字行中,我们必须覆盖零到正整数1,这表示整数1,之后必须将1和2分成4部分,并且必须覆盖0以外的3个位置,这表示3/4 。 0以外的七个位置代表7/4。 P代表7/4。
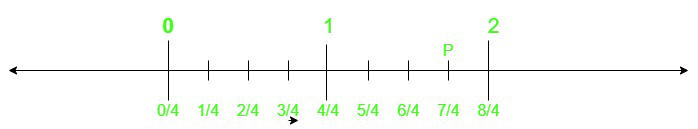
(ii)为了表示– 5/6,我们必须将0到– 1的整数除以6部分,并且对于– 5/6,我们必须从0移5个位。

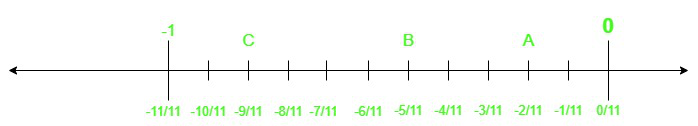
问题2:在数字行上代表2/11,-5 / 11,-9 / 11?
解决方案:
我们必须将0到– 1的整数划分为11个部分,从0到它的左侧的2、5、9的距离代表– 2/11,-5 / 11,-9 / 11,分别标记为A,B,C,分别。
问题3.写出小于2的五个有理数?
解决方案:
We can write the number 2 as 6 / 3
Hence, we can write, the five rational numbers which are smaller than 2 are:
1 / 3 , 2 / 3 , 3 / 3 , 4/ 3 , 5 / 3
问题4.找到介于2/5和1/2之间的十个有理数吗?
解决方案:
For finding rational numbers between fractions we have to take L. C. M. of their denominators or its multiples. Here L. C. M. Of 5 and 2 is 10 and for finding fractions between them we have to take multiple of 10. Let us take 20 as denominator.
So,
-2 / 5 = (- 2 / 5) × (4 / 4) = -8 / 20
Also,
1 / 2 = (1 / 2) × (10 / 10) = 10 / 20
Hence ten rational numbers between – 2 / 5 to 1 / 2 are same as rational numbers between – 8 / 20 and 10 / 20. And those are as follows
-7 / 20, -6 / 20, -5 / 20, -4 / 20, -3 / 20, -2 / 20, -1 / 20, 0, 1 / 20, 2 / 20
问题5.找到五个有理数
(i)2/3和4/5(ii)– 3/2和5/3(iii)1/4和1/2
解决方案:
(i) 2 / 3 and 4 / 5
For finding rational numbers between fractions we have to take L. C. M. of their denominators or its multiples.
Here L. C. M. Of 3 and 5 is 15
And we take the denominators as multiple of 15, as 60
Hence
2 / 3 = ( 2 / 3 ) × ( 20 / 20 ) = 40 / 60
4 / 5 = ( 4 / 5 ) × ( 12 / 12 ) = 48 / 60
Five rational numbers between 2 / 3 and 4 / 5 same as five rational numbers between
40 / 60 and 48 / 60
Therefore, Five rational numbers between 40 / 60 and 48 / 60 are as follows
41 / 60, 42 / 60, 43 / 60, 44 / 60, 45 / 60
(ii) -3 / 2 and 5 / 3
Similarly,
L. C. M. of 2 and 3 is 6.
Here we take denominators same as 6.
-3 / 2 = ( -3 / 2 ) × ( 3 / 3 ) = -9 / 6
5 / 3 = ( 5 / 3 ) × ( 2 / 2 ) = 10 / 6
Hence five rational numbers between -3 / 2 and 5 / 3 are same as five rational numbers between -9 / 6 and 10 / 6 and those are as follows
-8 / 6, -7 / 6, -1, -5 / 6, -4 / 6
(iii) 1 / 4 and 1 / 2
Here L. C. M. of 4 and 2 is 8.
Here we take denominator as multiple of 8 say 32.
Hence
1 / 4 = ( 1 / 4 ) × (8 / 8) = 8 / 32
1 / 2 = ( 1 / 2 ) × ( 16 / 16 ) = 16 / 32
Hence five rational numbers between 1 / 4 and 1 / 2 are same as five rational numbers between 8/32 and 16/32 and those are as follows
9 / 32, 10 / 32, 11 / 32, 12 / 32, 13 / 32
问题6.写出大于–2的五个有理数?
解决方案:
We can write -2 as -10 / 5
Hence five rational numbers greater than -2 are as follows
-1 / 5, -2 / 5, -3 / 5, -4 / 5 ,-1
问题7.找到3/5和3/4之间的十个有理数?
解决方案:
L .C. M. of 4 and 5 is 20. For finding rational number between them we should make denominator same or multiple of L .C.M.
Here we take 80.
3 / 5 = ( 3 / 5) × ( 16 / 16 ) = 48 / 80
3 / 4 = ( 3 / 4 ) × ( 20 / 20 ) = 60 / 80
Ten rational numbers between 3 / 5 and 3 / 4 are same as ten rational numbers between 48 / 80 and 60 / 80
Ten rational numbers between 48 / 80 and 60 / 80 are as follows
49 / 80, 50 / 80, 51 / 80, 52 / 80, 54 / 80, 55 / 80, 56 / 80, 57 / 80, 58 / 80, 59 / 80