8类RD Sharma解–第8章代数表达式的除法–练习8.4 |套装2

问题19.将30x ⁴ + 11x ³ – 82x ² – 12x + 48除以3x ² + 2x – 4

解决方案：

We have to divide 30x⁴ + 11x³ – 82x² – 12x + 48 by 3x² + 2x – 4

So by using long division method we get

Quotient = 10x² – 3x – 12

Remainder = 0

问题20.将9x ⁴ – 4x ² + 4除以3x ² – 4x + 2

解决方案：

We have to divide 9x⁴ – 4x² + 4 by 3x² – 4x + 2

So by using long division method we get

Quotient = 3x² + 4x + 2

Remainder = 0

问题21.在以下每个中，验证除法算法，即，除数=除数*商+余数。另外，写出商和余数：

(i)股息= 14x ² + 13x – 15，除数= 7x – 4

解决方案：

Dividing the Dividend by divisor, we get

Quotient = 2x + 3

Remainder = -3

(ii)股息= 15z ³ – 20z ² + 13z – 12，除数= 3z – 6

解决方案：

Dividing the Dividend by divisor, we get

33z – 66

Quotient = 5z² + (10/3)z + 11

Remainder = 54

(iii)股息= ^{6y 5} – 28y ³ + 3y ² + 30y – 9，除数= 2y ² – 6

解决方案：

Dividing the Dividend by divisor, we get

3y² – 9

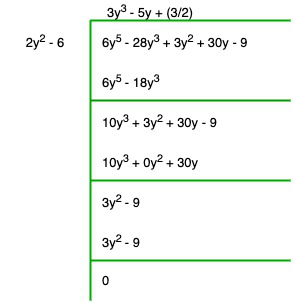
Quotient = 3y³ – 5y + (3/2)

Remainder = 0

(iv)股息= 34x – 22x ³ – 12x ⁴ – 10x ² – 75，除数= 3x + 7

解决方案：

Dividing the Dividend by divisor, we get

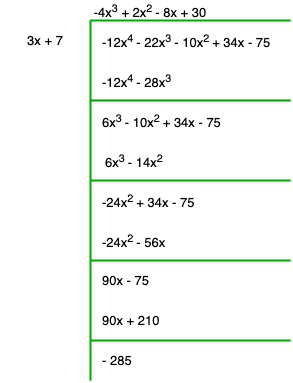
Quotient = -4x³ + 2x² – 8x + 30

Remainder = -285

(v)股息= 15y ⁴ – 16y ³ + 9y ² –(10/3)y + 6，除数= 3y – 2

解决方案：

Dividing the Dividend by divisor, we get

Quotient = 5y³ – 2y² + (5/3)y

Remainder = 6

(vi)股息= 4y ³ + 8y + 8y ² + 7，除数= 2y ² – y + 1

解决方案：

Dividing the Dividend by divisor, we get

Quotient = 2y + 5

Remainder = 11y + 2

(vii)股息= 6y ⁵ + 4y ⁴ + 4y ³ + 7y ² + 27y + 6，除数= 2y ³ +1

解决方案：

Dividing the Dividend by divisor, we get

Quotient = 3y² + 2y + 2

Divisor = 4y² + 25y + 4

问题22.将15y ⁴ + 16y ³ +(10/3)y – 9y ² – 6除以3y – 2。写下商中的系数。

解决方案：

We have to divide 15y⁴ + 16y³ + (10/3) y – 9y² – 6 by 3y – 2

So by using long division method we get

Quotient = 5y³ + (26/3)y² + (25/9)y + (80/27)

Remainder = (-2/27)

Co-efficient of y³ is 5

Co-efficient of y² is 26/9

Co-efficient of y is 25/9 and,

Constant term = 80/27

问题23：使用多项式除法说明是否。

(i)x + 6是x ² – x – 42的因数

解决方案：

Dividing x² – x – 42 by x + 6, we get

-7x – 42

Remainder = 0

Therefore, x + 6 is a factor of x² – x – 42

(ii)4x – 1是4x ² – 13x – 12的系数

解决方案：

On dividing 4x² – 13x – 12 by 4x – 1

Remainder = -15

Therefore, 4x-1 is not a factor of 4x² – 13x – 12

(iii)2y – 5是4y ⁴ – 10y ³ – 10y ² + 30y – 15的因数

解决方案：

On dividing 4y⁴ – 10y³ – 10y² + 30y – 15 by 2y – 5, we get

Remainder = -5/2

Therefore, 2y – 5 is not a factor of 4y⁴ – 10y³ – 10y² + 30y – 15

(iv)3y ² + 5是6y ⁵ + 15y ⁴ + 16y ³ + 4y ² + 10y – 35的因数

解决方案：

On dividing 6y⁵ + 15y⁴ + 16y³ + 4y² + 10y – 35 by 3y² + 5, we get

Remainder = 0

Therefore, 3y² + 5 is a factor of 6y⁵ + 15y⁴ + 16y³ + 4y² + 10y – 35

(v)z ² + 3是z ⁵ – 9z的因数

解决方案：

On dividing z⁵ – 9z by z² + 3, we get

-3z³ – 9z

Remainder = 0

Therefore, z² + 3 is a factor of z⁵– 9z

(vi)2x ² – x + 3是6x ⁵ – x ⁴ + 4x ³ – 5x ² – x – 15的系数

解决方案：-

On dividing 6x⁵ – x⁴ + 4x³ – 5x² – x – 15 by 2x² – x + 3 

-10x² + 5x – 15

Remainder = 0

Therefore, 2x² – x + 3 is a factor of 60x⁵ – x⁴ + 4x³ – 5x² – x – 15 

^{问题24.如果x + 2是4x 4} + 2x ³ – 3x ² + 8x + 5a的因数，则求出’a’的值。

解决方案：

Given that, x + 2 is a factor of 4x⁴ + 2x³ – 3x² + 8x + 5a,

On dividing 4x⁴ + 2x³ – 3x² + 8x + 5a by x + 2, we get

Remainder = 5a + 20

5a + 20 = 0

a = -4

问题25.必须对x ⁴ + 2x ³ – 2x ² + x – 1加什么，以便所得的多项式可以被x ² + 2x – 3整除。

解决方案：

On dividing x⁴ + 2x³ – 2x² + x – 1 by x² + 2x – 3, we get

Remainder = 0

The No. added to given polynomial to get remainder 0 will be :

x + 2 = 0