问题1.两个半径为5cm和3cm的圆在两个点处相交,并且它们的中心之间的距离为4 cm。找到普通和弦的长度。
解决方案:
Given: OP=4cm, AP=3cm, QR=5cm
To find: In ∆APO:
AO²=5²=25
OP²=4²=16
AP²=3²=9
OP²+AP²=AO²
BY converse of Pythagoras theorem
ΔAPO: is a right ∠D=P
Now, in the bigger circle OP is perpendicular AB
AP=½AB —————-(perpendicular from the center of circle to a chord bisect the chord )
3=½AB
6=AB
∴Therefore the length of common chord is 6cm.

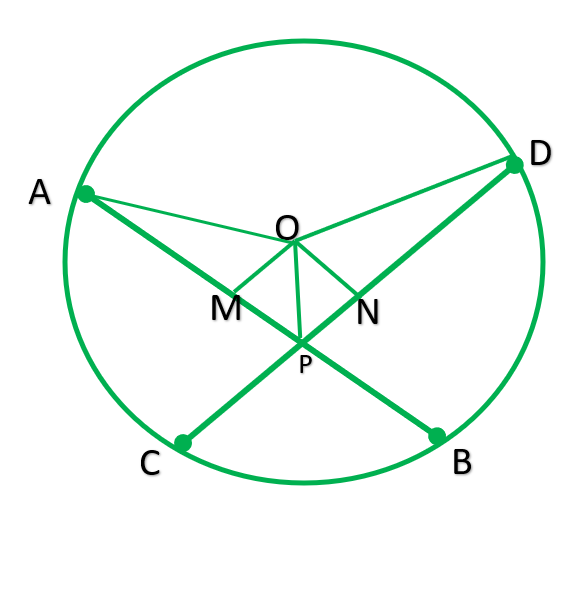
问题2.如果一个圆的两个相等的弦在圆内相交,请证明将相交点连接到中心的线与该弦成相等的角度。
解决方案:
Given: Equal chord AB & CD intersect at P.
To find: AP=PD and PB=PC
Construction: Draw OM perpendicular AB ,ON perpendicular CD and join OP.
Because perpendicular from center bisect the chord
∴AM=MB=½AB also CN=ND=½CD
AM=MB=CN=ND ——————1
Now, In ∆OMP and ∆ONP
ANGLE M=ANGLE N [90° both]
OP=OP [COMMON]
ON=OM [equal chords are equilateral from center]
∴∆OMP≅∆ONP
Therefore MP=PN (C.P.C.T.) ——————2
i)from 1 and 2
AM+MP=ND+AN
AP=PD
ii)MB-MP=CN=PN
PB=PC
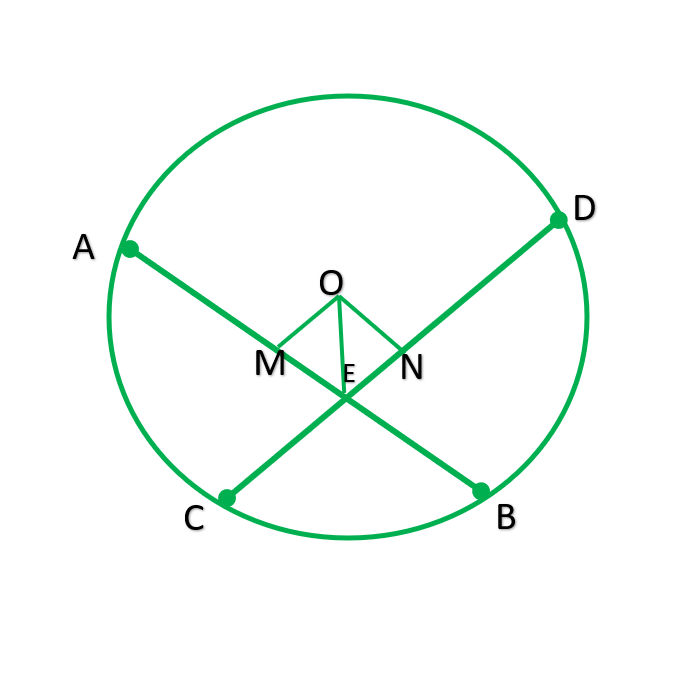
问题3.如果一个圆的两个相等的弦在圆内相交,请证明将相交点连接到中心的线与该弦成相等的角度。
解决方案:
Given: Equal chords AB and CD intersect at P.
To prove: angle1=angle=2
Construction: Draw OM perpendicular AB & ON perpendicular CD.
Solution: In ∆OMP & ∆ONP
Angle M= Angel N [90 ° each]
OP=OP [common]
OM=ON —————[ Equal chords are equal distant from center]
∴∆OMP≅∆ONP ———-[R.H.S]
∴∠1=∠2 ———–[C.P.C.T]
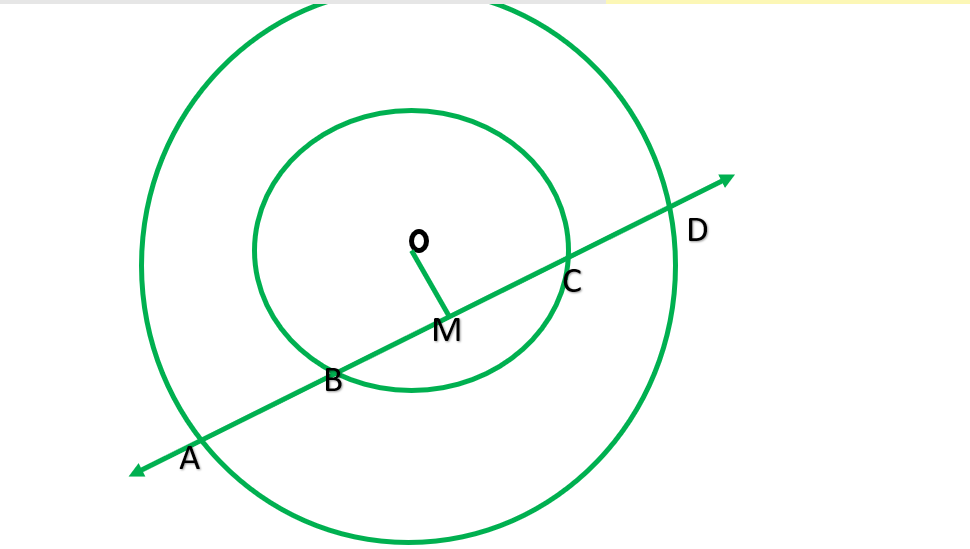
问题4.如果一条线与两个同心圆(具有相同中心的圆)相交,且其中心O在A,B,C和D处,则证明AB = CD(见图)。
解决方案:
Given : two concentric circle with O. A line intersect them at A, B, C , and D
To prove: AB=CD
construction: Draw OM ⊥ AD ,In bigger circle AD is chord OM ⊥ AD.
∴AM=MD —————-[⊥ from center of circle of a circle bisects the chord] __________ 1
The smaller circle :
BC is chord OM ⊥ BC
BM=MC ——————-[⊥ from center of circle of a circle bisects the chord] __________ 2
subtracting 1-2
AM-BM=MD-MC
AB=CD
问题5。三个女孩Reshma,Salma和Mandip站在公园绘制的半径5m的圆圈上,正在玩游戏。 Reshma将球丢给Salma,Salma丢给Mandip,Mandip丢给Reshma。如果Reshma和Salma之间以及Salma和Mandip之间的距离均为6m,那么Reshma和Mandip之间的距离是多少?
Solution:
To find RM=?
Let Reshma, Salma and Mandip be R,S,M
Construction: Draw OP ⊥ RS join OR and OS.
RP=½RS ___________[⊥ from center bisects the chord]
RP=½*6=3m
In right ΔORP
OP²=OR²- PR²
OP= √ 5² -3²
=√259 =√16 =4
Area of ΔORS=½*RS*OP
=½*6*4=12m² —————–1
Now, ∠N=90°
Area of ΔORS=½*SO*RN
=½*SO*RN ——————-2
Above ,1=2
12=½*5*RN
12/5*2=RN
RN=4.8
RM=2*RN _________________[⊥ from center bisects the chord]
=2*4.8
9.6m

问题6:一个殖民地内有一个半径为20m的圆形公园。三个男孩Ankur,Syed和David在其边界上等距坐着。每个男孩手里都拿着玩具电话互相交谈。查找每个电话的字符串长度。
解决方案:
Draw AM⊥SD
AS=SD=AD
∴ASD is the equilateral Δ
Let each side of Δ-2xm
SM=2x/2=x
Now in Δ DMS, by the Pythagoras theorem
AM²+SM²=AS²
AM²= AS²- SM²
AM=√(2x²+x² )
==√(3x² )
AM =√3x
OM=AM-AO
OM=√3x-20
Now in right ΔOMS
OM²+SM²=SO²
(√3x-20)²+2x²+x²=20²
20²+400-40√3x+x^2=400
4x²=40√3x
4xx=40√3x
X=(40√3)/4
X=10√3x
Length of each string =2x
=2*10√3xm
