第 12 类 RD Sharma 解决方案 – 第 22 章微分方程 – 练习 22.2 |设置 1

问题 1. 形成由 y ² = (x – c) ³表示的曲线族的微分方程

解决方案：

y² = (x – c)³     

On differentiating the given equation w.r.t x,

2y(dy/dx) = 3(x – c)²

(x – c)² = (2y/3)(dy/dx)

(x – c) = [(2y/3)(dy/dx)]^1/2          -(1)

On putting the value of (x – c) in equation (1), we get

y² = [(2y/3)(dy/dx)]^3/2

On squaring both side, we get

y⁴ = [(2y/3)(dy/dx)]³

y⁴ = (8y³/27)(dy/dx)³  

27y⁴ = 8y³(dy/dx)³ 

27y = 8(dy/dx)³                 

问题2. 消去m，形成y = e ^mx对应的微分方程。

解决方案：

y = e^mx          -(1)

On differentiating the given equation w.r.t x,

dy/dx = me^mx          -(2)

From eq(1), we get

y = e^mx 

logy = mx

m = (logy/x)

Now, put the value of m in equation(2), we get

x(dy/dx) = ylogy

问题 3. 由以下原语形成微分方程，其中常数是任意的。

(i) y ² = 4ax

解决方案：

y² = 4ax          -(1)

y²/4x = a

On differentiating the given equation w.r.t x,

2y(dy/dx) = 4a          -(2)

Now, put the value of a in equation(2), we get

2y(dy/dx) = 4(y²/4x)  

2y(dy/dx) = y²/x

2x(dy/dx) = y

(ii) y = cx + 2c ² + c ³

解决方案：

y = cx + 2c² + c³          -(1)

On differentiating the given equation w.r.t x,

dy/dx = c          -(2)

Now, put the value of c in equation(1), we get

y = x(dy/dx) + 2(dy/dx)² + (dy/dx)³

(iii) xy = a ²

解决方案：

xy = a²          -(1)

On differentiating the given equation w.r.t x,

x(dy/dx) + y = 0

(iv) y = ax ² + bx + c

解决方案：

y = ax² + bx + c          -(1)

On differentiating the given equation w.r.t x,

dy/dx = 2ax + b          -(2)

Again differentiating the given equation w.r.t x,

d²y/dx² = 2a          -(3)

Again, differentiating the given equation w.r.t x, we get

d³y/dx³ = 0  

问题 4. 求曲线族 y = Ae ^2x + Be ^-2x的微分方程，其中 A 和 B 是任意常数。

解决方案：

y = Ae^2x + Be^-2x          -(1)

On differentiating the given equation w.r.t x,

(dy/dx) = 2Ae^2x – 2Be^-2x          -(2)

Again, differentiating the given equation w.r.t x,

d²y/dx² = 4Ae^2x + 4Be^-2x  

d²y/dx² = 4(Ae^2x + Be^-2x)

d²y/dx² = 4y

问题 5. 求曲线族的微分方程 x = Aconst + Bsinnt 其中 A 和 B 是任意常数。

解决方案：

x = Acosnt + Bsinnt          -(1)

On differentiating the given equation w.r.t x,

dy/dx = -Ansinnt + Bncosnt          -(2)

Again, differentiating the given equation w.r.t x,

d²y/dx² = -An²cosnt – Bn²sinnt 

d²y/dx² = -n²(Acosnt + Bsinnt) 

d²y/dx² + n²x = 0

问题 6. 消去 a 和 b，形成 y ² = a(b – x ² ) 对应的微分方程。

解决方案：

y² = a(b – x²) 

On differentiating the given equation w.r.t x,

2y(dy/dx) = a(0 – 2x)

Again, differentiating the given equation w.r.t x,

$2[y\frac{d^2y}{dx^2}+(\frac{dy}{dx})^2]=-2a$

$[y\frac{d^2y}{dx^2}+(\frac{dy}{dx})^2]=-(\frac{y}{-x}*\frac{dy}{dx})$

x[$x[y\frac{d^2y}{dx^2}+(\frac{dy}{dx})^2]=y\frac{dy}{dx}$

问题7. 消去a，形成y ² – 2ay + x ² = a ²对应的微分方程。

解决方案：

y² – 2ay + x² = a²          -(1)

On differentiating the given equation w.r.t x,

2y(dy/dx) – 2a(dy/dx) + 2x = 0

2y(dy/dx) + 2x = 2a(dy/dx)

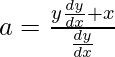$a=\frac{y\frac{dy}{dx}+x}{\frac{dy}{dx}}$        -(2)

Let us considered dy/dx = y’ 

On putting the value of ‘a’ in the eq(1), we get 

$y^2-2[\frac{y\frac{dy}{dx}+x}{\frac{dy}{dx}}]y+x^2=[\frac{y\frac{dy}{dx}+x}{\frac{dy}{dx}}]^2$

$\frac{y'y^2-2y'y^2-2xy+y'x^2}{y'}=\frac{y'^2y^2+x^2+2xyy'}{y'^2}$       

On solving this equation, we get

(x² – 2y²)y’² – 4xyy’ – x² = 0

(x² – 2y²)(dy/dx)² – 4xy(dy/dx) – x² = 0

问题 8. 消去 a 和 b，形成对应于 (x – a) ² + (y – b) ² = r ²的微分方程。

解决方案：

(x – a)² + (y – b)² = r²          -(1)

On differentiating the given equation w.r.t x,

2(x – a) + 2(y – b)(dy/dx) = 0   

(x – a) + (y – b)(dy/dx) = 0          -(2)

Again, differentiating the given equation w.r.t x,

1 + (y – b)(d²y/dx²) + (dy/dx)(dy/dx) = 0    

$(y-b)=-[\frac{(\frac{dy}{dx})^2+1}{\frac{d^2y}{dx^2}}]$           -(3)

On putting the value of (y – b) in eq(2),

$(x-a)-[\frac{(\frac{dy}{dx})^2+1}{\frac{d^2y}{dx^2}}]\frac{dy}{dx}=0$

(x – a)(d²y/dx²) – (dy/dx)³ – (dy/dx) = 0  

$(x-a)=\frac{(\frac{dy}{dx})^3+(\frac{dy}{dx})}{(\frac{d^2y}{dx^2})}$        -(4)

On putting the value of (x – a) and (y – b) in eq(1)

$[\frac{(\frac{dy}{dx})^3+(\frac{dy}{dx})}{(\frac{d^2y}{dx^2})}]^2+ [\frac{(\frac{dy}{dx})^2+1}{\frac{d^2y}{dx^2}}]^2=r^2$

Put (dy/dx) = y’ and d²y/dx² = y” 

y’²(1 + y’²)² + (1 + y’²)² = r²y”²   

问题 9. 求所有通过原点且圆心在轴上的圆的微分方程。

解决方案：

Equation of the circle is (x – a)² + (y – b)² = r² 

Here, a and b are the centre of the circle.

(x – a)² + (y – b)² = r²          -(1)

When the centre lies on the y-axis, so a = 0 

x² + (y – b)² = r²          -(2)

So, when the circle is passing through origin, so the equation is

0² + b² = r²          -(3)

x² + (y – b)² = r² 

On squaring both side, we have

x² + y² – 2yr + r² = r²          -(Since  r² = b²)

2yr = x² + y²

r = (x² + y²)(2y)

On differentiating the equation(1) w.r.t x, we get

$0=\frac{2y(2x+2y\frac{dy}{dx})-(x^2+y^2)2\frac{dy}{dx}}{(2y)^2}$

0 = 4xy + 4y²(dy/dx) – 2x²(dy/dx) – 2y²(dy/dx)

0 = y²(dy/dx) – x²(dy/dx) + 2xy

(x² – y²)(dy/dx) = 2xy 

问题 10. 求所有过原点且圆心在 x 轴上的圆的微分方程。

解决方案：

Equation of the circle is (x – a)² + (y – b)² = r² 

Here, a and b are the centre of the circle.

When the centre lies on the x-axis, so b = 0 

(x – a)² + y² = r²          -(1)

When the circle is passing through origin, so the equation is

a² + 0² = r²          -(2)

(x – a)² + y² = r²

On squaring both side, we get,

x² – 2ax + a² + y² = r²

x² + y² – 2xr + r² = r²           -(Since  r² = a²)

2xr = x² + y²  

r = (x² + y²)(2x)          -(3)

On differentiating the equation w.r.t x, we get

$0=\frac{2x(2x+2y\frac{dy}{dx})-(x^2+y^2)2}{(2x)^2}$

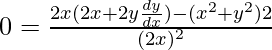
0 = 2x² + 2xy(dy/dx) – x² – y²

(x² – y²) + 2xy(dy/dx) = 0