第 12 类 RD Sharma 解决方案 – 第 22 章微分方程 – 练习 22.2 |设置 2

问题 11. 假设雨滴的蒸发速度与其表面积成正比。形成一个涉及雨滴半径变化率的微分方程。

解决方案：

Let us considered ‘r’ be the radius of rain drop, volume of the drop be ‘V’ and area of the drop be ‘A’

(dV/dt) proportional to A

(dV/dt) – kA             -(V decreases with increasing in t so negative sing)

Here, k is proportionality constant,

$\frac{d(\frac{4π}{3}r^3)}{dt}$= -k(4π r²)

4πr²(dr/dt) = -k(4πr²)

(dr/dt) = -k

问题 12. 找出所有直角为 4a' 且轴平行于 x 轴的抛物线的微分方程。

解决方案：

Equation of parabola whose area is parallel to x-axis and vertices at (h, k).

(y – k)² = 4a(x – h)          -(1)

On differentiating w.r.t x,

2(y – k)(dy/dx) = 4a  

(y – k)(dy/dx) = 2a 

$(y-k)=\frac{2a}{\frac{dy}{dx}}$    -(2)

Again, differentiating w.r.t x,

d²y/dx²(y – k) + (dy/dx)(dy/dx) = 0   

$(\frac{2a}{\frac{dy}{dx}})\frac{d^2y}{dx^2}+(\frac{dy}{dx})^2=0$

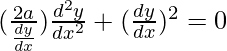
2a(d²y/dx²) + (dy/dx)³ = 0

问题 13. 证明其中的微分方程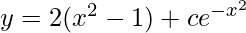$y=2(x^2-1)+ce^{-x^2}$是一个解，是 (dy/dx) + 2xy = 4x ³

解决方案：

$y=2(x^2-1)+ce^{-x^2}$        -(1)

On differentiating w.r.t x,

$\frac{dy}{dx}=4x-2cxe^{-x^2}$

On adding 2xy in R.H.S and L.H.S,

$\frac{dy}{dx}+2xy=4x-2cxe^{-x^2}+2xy$

On putting the value of y in above equation,

$\frac{dy}{dx}+2xy=4x-2cxe^{-x^2}+2x(2(x^2-1)+ce^{-x^2})$

$4x-2cxe^{-x^2}+4x^3-4x+2xce^{-x^2}$=$4x^3$

(dy/dx) + 2xy = 4x³

问题 14. 从 y = (sin ^-1 x) ² + A cos ^-1 x + B 的微分方程，其中 A 和 B 是任意常数，作为其通解。

解决方案：

y = (sin^-1x)² + A cos^-1x + B

On differentiating w.r.t x,

$\frac{dy}{dx} = 2sin^{-1}x(\frac{1}{\sqrt{1-x^2}})+A(\frac{-1}{\sqrt{1-x^2}})+0$

$\sqrt{1-x^2}\frac{dy}{dx}=2sin^{-1}x - A$

Again, on differentiating w.r.t x,

$\sqrt{1-x^2}\frac{d^2y}{dx^2}+\frac{dy}{dx}\frac{1}{\sqrt{1-x^2}}(-2x)\\ = 2(\frac{1}{\sqrt{1-x^2}})-0\\ =(1-x^2)\frac{d^2y}{dx^2}-2x\frac{dy}{dx}-2=0$

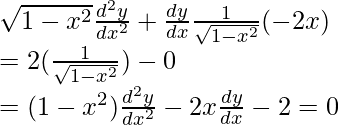
问题 15. 形成方程表示的曲线族的微分方程（a为参数）

(i) (2x + a) ² + y ² = a ²

解决方案：

(2x + a)² + y² = a²          -(1)

On differentiating w.r.t x,

2(2x + a) + 2y(dy/dx) = 0 

(2x + a) + y(dy/dx) = 0 

a = -2x – y(dy/dx)          -(2)

On putting the value of ‘a’ in eq(1), we have

$(2x-2x-y\frac{dy}{dx})^2+y^2=(-2x-y\frac{dy}{dx})^2$

$(y\frac{dy}{dx})^2+y^2=(4x^2+4xy\frac{dy}{dx}+y^2(\frac{dy}{dx})^2)$

y² = 4x² + 4xy(dy/dx)

y² – 4x² – 4xy(dy/dx) = 0

(ii) (2x – a) ² – y ² = a ²

解决方案：

(2x – a)² – y² = a²          

4x² – 4ax + a² – y² = a² 

4ax = 4x² – y²

a = (4x² – y²)/4x        

On differentiating w.r.t x,

$[\frac{4x(8x-2y\frac{dy}{dx}-4(4x^2-y^2}{(4x)^2}]=0$

$32x^2-8xy\frac{dy}{dx}-16x^2+4y^2=0$

$16x^2+4y^2-8xy\frac{dy}{dx}=0$

4x² + y² = 2xy(dy/dx)

(iii) (x – a) ² + 2y ² = a ²

解决方案：

(x – a)² + 2y² = a²          -(1)

On differentiating w.r.t x,

2(x – a) + 4y(dy/dx) = 0

(x – a) + 2y(dy/dx) = 0

a = x + 2y(dy/dx)          -(2)

On putting the value of a in eq(1)

$(x-x-2y\frac{dy}{dx})^2+2y^2=(x+2y\frac{dy}{dx})^2$

$4y^2(\frac{dy}{dx})^2+2y^2=x^2+4xy(\frac{dy}{dx})+4y^2(\frac{dy}{dx})^2$

2y² – 4xy(dy/dx) – x² = 0

问题 16. 通过形成相应的微分方程（a，b 为参数）表示以下曲线族：

(i) x ² + y ² = a ²

解决方案：

x² + y² = a²

On differentiating w.r.t x,

2x + 2y(dy/dx) = 0

x + y(dy/dx) = 0

(ii) x ² – y ² = a ²

解决方案：

x² – y² = a² 

On differentiating w.r.t x,

2x – 2y(dy/dx) = 0

x – y(dy/dx) = 0

(iii) y ² = 4ax

解决方案：

y² = 4ax 

(y2/x) = 4a

On differentiating w.r.t x,

$[\frac{2xy\frac{dy}{dx}-y^2}{x^2}]=0$

2xy(dy/dx) – y² = 0

2x(dy/dx) – y = 0

(iv) x ² + (y – b) ² = 1

解决方案：

x² + (y – b)² = 1          -(1)

On differentiating w.r.t x,

2x + 2(y – b)(dy/dx) = 0

$(y-b)=-\frac{x}{\frac{dy}{dx}}$

On putting the value of (y – b) in eq(1)

$x^2+[-\frac{x}{\frac{dy}{dx}}]^2=1$

x²(dy/dx)² + x² = (dy/dx)²

x²[(dy/dx)² + 1] = (dy/dx)²

(v) (x – a) ² – y ² = 1

解决方案：

(x – a)² – y² = 1           -(1)

On differentiating w.r.t x,

2(x – a) – 2y(dy/dx) = 0

(x – a) – y(dy/dx) = 0

(x – a) = y(dy/dx)

On putting the value of (y – b) in eq(i), we get

y²(dy/dx)² – y² = 1

y²[(dy/dx)2 – 1] = 1

(六) 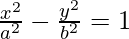$\frac{x^2}{a^2}-\frac{y^2}{b^2}=1$

解决方案：

We have,

$\frac{x^2}{a^2}-\frac{y^2}{b^2}=1$       -(1)

$\frac{b^2x^2-a^2y^2}{a^2b^2}=1$

{(bx)² – (ay)²} = (ab)²           -(2)

On differentiating w.r.t x,

2xb² – 2a²y(dy/dx) = 0

xb² – a²y(dy/dx) = 0           -(3)

Again, differentiating w.r.t x,

$b^2-a^2[y(\frac{dy}{dx})(\frac{dy}{dx})+y\frac{d^2y}{dx^2}]$

$b^2=a^2[y(\frac{dy}{dx})(\frac{dy}{dx})+y\frac{d^2y}{dx^2}]$

On putting the value of b² in equation(3), we get

xb² – a²y(dy/dx) = 0  

$xa^2[y(\frac{dy}{dx}^2)+y\frac{d^2y}{dx^2}]-a^2y\frac{dy}{dx}=0$

$xy\frac{d^2y}{dx^2}+x(\frac{dy}{dx})^2-y(\frac{dy}{dx})=0$

$x[y\frac{d^2y}{dx^2}+(\frac{dy}{dx})^2]=y(\frac{dy}{dx})$

(vii) y ² = 4a(x – b)

解决方案：

We have,

y² = 4a(x – b)          

On differentiating w.r.t x,

2y(dy/dx) = 4a

Again differentiating w.r.t x,

$2[(\frac{dy}{dx})(\frac{dy}{dx})+y\frac{d^2y}{dx^2}]=0$

[(dy/dx)² + y(d²y/dx²)] = 0

(viii) y = 斧头³

解决方案：

We have,

y = ax³         -(1)

On differentiating w.r.t x,

(dy/dx) = 3ax²

From eq(1),

a=(y/x³         -(1)

On putting the value of a in eq(1)

dy/dx = 3(y/x³) × x²

x(dy/dx) = 3y

(ix) x ² + y ² = 斧头³

解决方案：

We have,

x² + y² = ax³       

a = (x² + y²)/(x³) 

On differentiating w.r.t x,

$\frac{(2x+2y\frac{dy}{dx})(x^3)-(3x^2)(x^2+y^2)}{(x^3)^2}=0$

$2x^3y\frac{dy}{dx}+2x^4-3x^4-3x^2y^2=0$

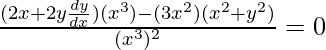
2x³y(dy/dx) = x⁴ + 3x²y²

2x³y(dy/dx) = x²(x² + 3y²)

2xy(dy/dx) = (x² + 3y²)

(x) y = e ^ax

解决方案：

We have,

y = e^ax         -(1)

On differentiating w.r.t x,

dy/dx = ae^ax

dy/dx = ay         -(2)

y = e^ax 

On taking log both side, we get

logy = ax

a = (logy/x)

Now, put the value of ‘a’ in eq(2)

(dy/dx) = logy/x) × y

x(dy/dx) = ylogy

问题 17. 形成代表椭圆族的微分方程，这些椭圆的焦点在 x 轴，中心在原点。

解决方案：

We have,

Equation of ellipse having foci on the x-axis,

 $\frac{x^2}{a^2}+\frac{y^2}{b^2}=1$       -(where a > b)

$\frac{b^2x^2+a^2y^2}{a^2b^2}=1$

(bx)² + (ay)² = (ab)²         -(1)

On differentiating above equation w.r.t x,

2b²x + 2a²y(dy/dx) = 0

b²x + a²y(dy/dx) = 0         -(2)

Again, differentiating w.r.t x,

$b^2+a^2[y(\frac{dy}{dx})(\frac{dy}{dx})+y\frac{d^2y}{dx^2}]=0$

$b^2=-a^2[y(\frac{dy}{dx})(\frac{dy}{dx})+y\frac{d^2y}{dx^2}]$

On putting the value of b² in eq(2),

xb² + a²y(dy/dx) = 0

$-xa^2[y(\frac{dy}{dx}^2)+y\frac{d^2y}{dx^2}]+a^2y\frac{dy}{dx}=0$

$xy\frac{d^2y}{dx^2}+x(\frac{dy}{dx})^2-y(\frac{dy}{dx})=0$

x[y(d²y/dx²) + (dy/dx)²] = y(dy/dx)

问题 18. 形成焦点在 X 轴和中心在原点的双曲线族的微分方程

解决方案：

We have,

Equation of a hyperbola having a Centre at the origin and foci along x-axis

$\frac{x^2}{a^2}-\frac{y^2}{b^2}=1$       -(1)

$\frac{b^2x^2-a^2y^2}{a^2b^2}=1$

(bx)²-(ay)²=(ab)²         -(2)

On differentiating above equation w.r.t x,

2xb²-2a²y(dy/dx)=0

xb²-a²y(dy/dx)=0          -(3)

Again, differentiating above equation w.r.t x,

$b^2-a^2[y(\frac{dy}{dx})(\frac{dy}{dx})+y\frac{d^2y}{dx^2}]=0$

$b^2=a^2[y(\frac{dy}{dx})(\frac{dy}{dx})+y\frac{d^2y}{dx^2}]$

Putting the value of b² in equation(3),

$xb^2-a^2y\frac{dy}{dx}=0$

$xa^2[y(\frac{dy}{dx}^2)+y\frac{d^2y}{dx^2}]-a^2y\frac{dy}{dx}=0$

xy(d²y/dx²) + x(dy/dx)² – y(dy/dx) = 0

x[y(d²y/dx²) +(dy/dx)²] = y(dy/dx)

This is required differential equation.

问题 19. 形成第二象限与坐标轴相接触的圆族的微分方程。

解决方案：

We have,

Let (-a, a) be the coordinates of the centre of circle 

So, the equation of circle is given by,

(x + a)² + (y – b)² = a²         -(1)

x² + 2ax + a² + y² – 2ay + a² = 0         -(2)

On differentiating above equation w.r.t x,

2x + 2a + 2y(dy/dx) – 2a(dy/dx) = 0

x + a + y(dy/dx) – a(dy/dx) = 0

$x+y\frac{dy}{dx}=a(\frac{dy}{dx}-1)$

$a=\frac{x+y\frac{dy}{dx}}{\frac{dy}{dx}-1}$

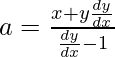
On substituting the value of ‘a’ in eq(2)

$[x+\frac{x+y\frac{dy}{dx}}{\frac{dy}{dx}-1}]^2+[y-\frac{x+y\frac{dy}{dx}}{\frac{dy}{dx}-1}]^2=[\frac{x+y\frac{dy}{dx}}{\frac{dy}{dx}-1}]^2$

Let, (dy/dx) = p 

[xp – x + x + yp]² + [yp – y – x – yp]² = [x + yp]²

(x + y)²p² + (x + y)² = (x + yp)²

(x + y)²[p² + 1] = (x + yp)²          -(where (dy/dx) = p)