角速度公式

速度就像您知道物体移动的快慢程度一样，就像您驾驶汽车的速度一样。现在，我们在这里谈论一种特定类型的速度。角速度只是速度的一种，但在这里身体必须沿圆形路径移动。

角速度公式

角速度定义为角位移的变化率，即物体沿圆形路径经过的角度。角速度是根据物体在所花费的时间内旋转/公转的次数来计算的。角速度用希腊字母“ω”表示，称为欧米茄。角速度的 SI 单位是 rad/s。

使用两个不同的公式计算角速度，

• ω = θ/t
• ω = v/r

公式推导

Let’s consider a body moving in a circular path with radius r shown above with a linear speed v. Let’s suppose that the body moves from point A to B covering a distance s through the circular arc and traversing an angle θ in time period t. 

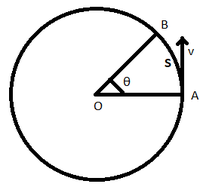
Circular path covered by a body

As known the angular speed is rate of change of displacement – Angular speed, ω = θ/t

So the formula for angular speed is ω = θ/t .

Another formula for angular speed

Despite the formula stated above, there is another and more widely used formula for calculation of angular speed from the point of view of competitive exams.

As ω = θ/t ⇢ (1)

Now we know that distance moved across arc of a circle is equal to radius times angle traversed. So,

s = rθ

=> θ = s/r ⇢ (2)

From (1) and (2),

ω = s/(rt) ⇢ (3)

Also from general understanding of linear speeds, 

v = s/t ⇢ (4)

From (3) and (4),

ω = v/r

示例问题

问题 1：考虑一个物体沿着半径为 5m 的圆形路径移动。它在 5 秒内覆盖了半圈。计算其角速度。

解决方案：

In half revolution, the angle traversed is 180 degrees. In radians, it is equal to π radians.

ω = θ/t

=> ω = π/5 = 0.628 rad/s

问题2：半径为2m的汽车车轮以10m/s的线速度旋转。计算它的角速度。

解决方案：

ω = v/r

ω = 10/2 

= 5 rad/s

问题 3：假设一辆赛车在速度为 18 km/hr 的圆形赛道上行驶，赛道半径为 0.2 m。计算汽车的角速度。

解决方案：

v = 18 km/hr = 5 m/s

r = 0.2 m

ω = v/r 

= 5/0.2 

= 25 rad/s

问题 4：一辆汽车沿半径为 2m 的圆形路径以 2 rad/s 的角速度运动。计算汽车在 2 秒内移动的角度（以度为单位）。

解决方案：

Given,  ω = 2 rad/s and t = 2s

Since ω = θ/t => θ = ωt

=> θ = (2 × 2) = 4 rad

In degrees, θ = 4 × (180/π) = 229.18 degree

问题 5：一个物体在 0.5 秒内以 7π rad/s 的角速度沿圆形路径移动了多少转？

解决方案：

Given ω = 7π rad/s and t = 0.5s

Since ω = θ/t => θ = ωt

θ = (7π × 0.5) = 3.5π

In 2π rad, revolutions covered is 1

=> In 1 rad, revolution covered is (1/2π)

=> In 3.5π rad, revolutions = 3.5π/2π = 1.75 revolutions

So, the body will complete 1 complete revolution and 3/4th of next revolution in time period of 0.5 s.

问题 6：物体在半径为 2m、弧长为 5s 的 4m 的圆形路径上运动的角速度是多少。

解决方案：

Given s = 4m, r = 2m, t = 5s

Using formula s = rθ => θ = s/r

θ = 4/2 = 2 rad

Since ω = θ/t

=> ω = 2/5 = 0.4 rad/s