体积公式

在几何中，三维形状是具有三维的实体。尺寸包含对象的长度，宽度和高度。 3D形状的一些示例包括立方体，圆锥体，圆柱体，金字塔，球体等。我们找到这些形状的体积，以便可以测量物体存储液体，气体等所占用的空间。存在不同的体积公式为不同的形状。通过使用这些公式，我们可以找到形状的体积。

在本节中，我们将学习所有三维形状的体积公式。

卷

对象占据的空间量称为该对象的体积，其中对象是3D对象。我们找到用于测量物体或容器容量的体积。通过使用公式，我们可以轻松找到任何3D形状的体积。如果3D形状很复杂，我们将使用积分计算来找到体积。

该体积由字母V表示。我们以立方单位或^3为单位测量体积。在下表中，我们将所有体积公式与该图进行了汇总，以更好地理解。

Shape	Figure	Volume Formula	Variables	Constant
Cube		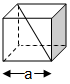V=a3 When diameter is given:	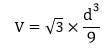a: is the length of the side d: is the length of the diameter	–
Cone		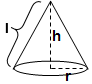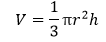When slant height is given:	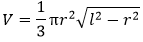r: is the radius h: is the perpendicular height l: is the slant height	π: is a constant whose value is 3.14 or 22/7
Cylinder		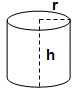V=πr2 h	r: is the radius h: is the height	π: is a constant whose value is 3.14 or 22/7
Hollow Cylinder		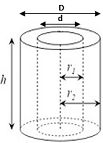V=πh(r22-r12)	r1: is the internal radius r2: is the external radius h: is the height of the cylinder D: is the external diameter d: is the internal diameter	π: is a constant whose value is 3.14 or 22/7
Cuboid		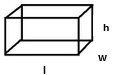V=l×w×h	l: is the length w: is the width h: is the height	–
Sphere		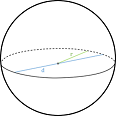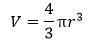When diameter is given: 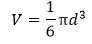When circumference is given: 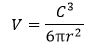When area is given:	r: is the radius d: is the diameter of the sphere C: is the circumference of the sphere A: is the area of the sphere	π: is a constant whose value is 3.14 or 22/7
Hemisphere		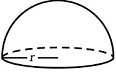	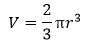r: is the radius	π: is a constant whose value is 3.14 or 22/7
Pyramid		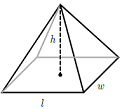	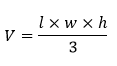l: is the base length w: is the base width h: is the height of the pyramid	–
Rectangular Prism		V=l×w×h	l: is the length w: is the width h: is the height	–
Triangular Prism		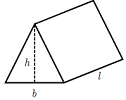	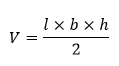l: is the length w: is the width h: is the height	–
Ellipsoid		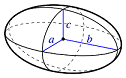	a, b, and c: are semi-axes of ellipsoid	π: is a constant whose value is 3.14 or 22/7
Tetrahedron		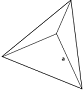	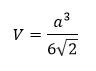a: is edge of tetrahedron	–