球体体积公式

测量是一门数学学科，涉及对各种几何形式的研究。它还解决了这种几何形状的面积和体积。它是与测量有关的数学领域，例如代数方程在几何形式的面积、体积和其他特征的测量中的表达和应用，而几何是与空间联系有关的数学分支。

领域

球体是几何形状为圆形的三维立体物体。从数学的角度来看，它是一组点的三维组合，这些点由一个等距离的公共点连接。与其他三维形状不同，球体没有顶点或边。它的中心与表面上的所有地方距离相等。换句话说，球体中心与其表面上任何一点之间的距离是相同的。

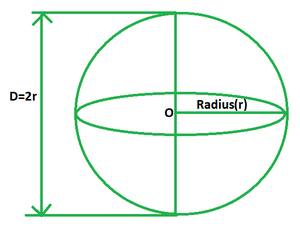
球体的体积

球体的体积是它在其中占据的空间量。球体是一个三维圆形实心形状，其表面上的所有点都与其中心等距。固定距离称为球体半径，固定点称为球体中心。当圆圈转动时，我们会注意到形式的变化。作为被称为圆的二维物体的旋转的结果，获得了球体的三维形状。

球体的体积公式由下式给出，

V = $\frac{4}{3}\pi R^3$

where,

R = radius of the sphere

π = 22/7

推导

Using the integration approach, we can simply calculate the volume of a sphere.

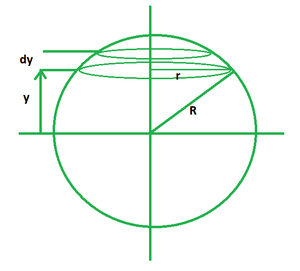
Suppose the sphere’s volume is made up of a series of thin circular discs stacked one on top of the other, as drawn in the diagram above. Each thin disc has a radius of r and a thickness of dy that is y distance from the x-axis.

Let the volume of a disc be dV. The value of dV is given by,

dV = (πr²)dy

dV = π (R² – y²)dy

The total volume of the sphere will be the sum of volumes of all these small discs. The required value can be obtained by integrating the expression from limit -R to R.

So, the volume of sphere becomes,

V = $\int_{y=-R}^{y=R} dV$

= $\int_{y=-R}^{y=R}π(R^2 - y^2)dy$

= $\pi|(R^2y - \frac{y^3}{3})dy|_{y=-R}^{y=R}$

= $\pi \left[R^3-\frac{R^3}{3}-(-R^3+\frac{R^3}{3})\right]$

= $\pi \left[2R^3-\frac{2R^3}{3}\right]$

= $\frac{4}{3}\pi R^3$

This derives the formula for volume of sphere.

示例问题

问题 1. 求一个半径为 9 厘米的球体的体积。

解决方案：

We have, r = 9.

Volume of sphere = 4/3 πr³

= (4/3) (3.14) (9) (9) (9)

= (4) (3.14) (3) (9) (9)

= 3052 cm³

问题 2. 求一个半径为 12 厘米的球体的体积。

解决方案：

We have, r = 12

Volume of sphere = 4/3 πr³

= (4/3) (3.14) (12) (12) (12)

= (4) (3.14) (4) (12) (12)

= 7234.56 cm³

问题 3. 求一个半径为 6 厘米的球体的体积。

解决方案：

We have, r = 6.

Volume of sphere = 4/3 πr³

= (4/3) (3.14) (6) (6) (6)

= (4) (3.14) (2) (6) (6)

= 904.32 cm³

问题 4. 求一个半径为 4 厘米的球体的体积。

解决方案：

We have, r = 4.

Volume of sphere = 4/3 πr3

= (4/3) (3.14) (4) (4) (4)

= (1.33) (3.14) (4) (4) (4)

= 267.27 cm³

问题 5. 求一个直径为 10 厘米的球体的体积。

解决方案：

We have, 2r = 10

=> r = 10/2

=> r = 5

Volume of sphere = 4/3 πr³

= (4/3) (3.14) (5) (5) (5)

= (1.33) (3.14) (5) (5) (5)

= 522.025 cm³

问题 6. 求一个直径为 16 厘米的球体的体积。

解决方案：

We have, 2r = 16

=> r = 16/2

=> r = 8

Volume of sphere = 4/3 πr³

= (4/3) (3.14) (8) (8) (8)

= (1.33) (3.14) (8) (8) (8)

= 2138.21 cm³

问题 7. 求一个直径为 14 厘米的球体的体积。

解决方案：

We have, 2r = 14

=> r = 14/2

=> r = 7

Volume of sphere = 4/3 πr³

= (4/3) (3.14) (7) (7) (7)

= (1.33) (3.14) (7) (7) (7)

= 1432.43 cm³