第 12 类 RD Sharma 解——第 19 章不定积分——练习 19.1

问题 1. 对以下关于 x 的积分求积分：

(i) ∫ x ⁴ dx

解决方案：

∫ x⁴ dx = x⁴⁺¹/(4+1) + Constant

= x⁵/5 + C

(ii) ∫ x ^5/4 dx

解决方案：

∫ x^5/4 dx = x^{5/4 + 1}/(5/4 +1) + Constant

= 4/9 x^9/4 + C

(iii) ∫ 1/x ⁵ dx

解决方案：

∫ 1/x⁵ dx = ∫ x^-5 dx

= x^-5+1/(-5+1) + Constant

= x^-4/(-4)+ C

= -1/(4x⁴) + C

(iv) ∫ 1/x ^3/2 dx

解决方案：

∫ x^-3/2 dx = x^{-3/2 + 1}/(-3/2 +1) + Constant

= x^-1/2/(-1/2) + C

= -2/(√x)+ C

(v) ∫ 3 ^x dx

解决方案：

∫ 3^x dx = 3^x/log3 + Constant

(vi) ∫ 1/x ^2/3 dx

解决方案：

∫ 1/x^2/3 dx = ∫ x^-2/3 dx

= x^{-2/3 + 1}/(-2/3+1) + Constant

= x^1/3/(1/3) + C

= 3x^1/3 + C

(vii) ∫ 3 ^{2log ₃ x} dx

解决方案：

∫ 3^{2log₃ x} dx = $∫ 3^{log_3 x^2} dx$

= ∫ x² dx

= x²⁺¹/(2+1) + Constant

= x³/3 + C

问题 2. 评估

（一世） $∫\sqrt{\frac{(1 + cos 2x)}{2} }dx$

解决方案：

 $∫\sqrt{\frac{(1 + cos 2x)}{2} }dx$ 

= $∫\sqrt{\frac{(1 + 2cos^2x - 1)}{2}} dx$

We know, cos 2x = 2cos² x – 1

=$∫\sqrt{\frac{(2cos^2x)}{2}} dx$ 

= ∫cos x dx

= sin x + Constant

(二) 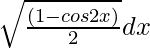$∫\sqrt{\frac{(1 - cos 2x)}{2} }dx$

解决方案：

$∫\sqrt{\frac{(1 - cos 2x)}{2} }dx$  

= $∫\sqrt{\frac{(1 - 1 + 2sin^2x)}{2}} dx$

We know, cos 2x = 1 – 2sin² x

= $∫\sqrt{\frac{(2sin^2 x)}{2}} dx$

= ∫ sin x dx

= -cos x + Constant

问题 3. 评估$∫ \frac{e^{6log_ex}-e^{5log_ex}}{e^{4log_ex}-e^{3log_ex}}$

解决方案：

 $∫ \frac{e^{6log_ex}-e^{5log_ex}}{e^{4log_ex}-e^{3log_ex}}$dx 

= $∫\frac{(x^6 - x^5)}{(x^4 - x^3)} dx$

We know, e ^{log_e x} = x

= $∫ \frac{x^5(x - 1)}{x^3(x - 1)} dx$

= ∫ x² dx

= x²⁺¹/2+1 + Constant

= x³/3 + C

问题 4. 评估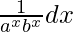： $∫ \frac{1}{a^x b^x}dx$

解决方案：

 $∫ \frac{1}{a^x b^x}dx$ = ∫ a^-x b^-x dx

= ∫ (ab)^-x dx

= (ab)^-x/log_e (ab)^-1 + Constant

= -a^-x b^-x/log_e (ab) + C

or

= -a^-x b^-x/ ln(ab) + C

问题 5. 评估

（一世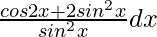） $∫ \frac{cos 2x + 2sin^2 x}{sin^2 x} dx$

解决方案：

 $∫ \frac{cos 2x + 2sin^2 x}{sin^2 x} dx$

= $∫ \frac{1 - 2sin^2x + 2sin^2x}{sin^2x} dx$

We know, cos 2x = 1 – 2sin² x

= ∫ 1/sin²x dx = ∫ cosec²x dx

= -cot x + Constant

(二) 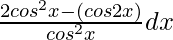$∫\frac{2cos^2x - (cos2x)}{cos^2x}dx$

解决方案：

 $∫\frac{2cos^2x - (cos2x)}{cos^2x}dx$

$∫\frac{2cos^2x - (2cos^2x -1)}{cos^2x}dx$

We know, cos 2x = 2cos² x – 1

= ∫ 1/cos² x dx = ∫ sec² x dx

= tan x + Constant

问题 6. 评估：∫ e ^log√x /x dx

解决方案：

∫ e^{log_e √x} /x dx = ∫√x/x dx

= ∫ x^-1/2 dx = x^{-1/2 + 1}/(-1/2 + 1) + Constant

= x^1/2 /(1/2) + C

= 2√x + C