问题1 计算以下数据的平均值和标准差:
| Expenditure (in ₹): | 0-10 | 10-20 | 20-30 | 30-40 | 40-50 |
| Frequency: | 14 | 13 | 27 | 21 | 15 |
解决方案:
| CI | f | x | u = (x – A)/h | fu | u2 | fu2 |
| 0 – 10 | 14 | 5 | -2 | -28 | 4 | 56 |
| 10 – 20 | 13 | 15 | -1 | -13 | 1 | 13 |
| 20 – 30 | 27 | 25 | 0 | 0 | 0 | 0 |
| 30 – 40 | 21 | 35 | 1 | 21 | 1 | 21 |
| 40 – 50 | 15 | 45 | 2 | 30 | 4 | 60 |
| 90 | 10 | 150 | ||||
Given:
Number of observations, N = 90 and A = 25
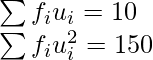
h = 10
Mean = ![]()
![]() = 25 + 10(10/90) = 26.11
= 25 + 10(10/90) = 26.11
![]()
= 10[(150/90) – (10/90)2]
= 165.4
Standard Deviation = √var(x) = √165.4 = 12.86
问题2.计算以下数据的标准差:
| Class: | 0-30 | 30-60 | 60-90 | 90-120 | 120-150 | 150-180 | 180-210 |
| Frequency: | 9 | 17 | 43 | 82 | 81 | 44 | 24 |
解决方案:
| CI | f | x | u = (x – A)/h | f × u | u2 | fu2 |
| 0 – 30 | 9 | 15 | -3 | -27 | 9 | 81 |
| 30 – 60 | 17 | 45 | -2 | -34 | 4 | 68 |
| 60 – 90 | 43 | 75 | -1 | -43 | 1 | 43 |
| 90 – 120 | 82 | 105 | 0 | 0 | 0 | 0 |
| 120 – 150 | 81 | 135 | 1 | 81 | 1 | 81 |
| 150 – 180 | 44 | 165 | 2 | 88 | 4 | 176 |
| 180 – 210 | 24 | 195 | 3 | 72 | 9 | 216 |
| 300 | 137 | 665 | ||||
Given: N = 300 and A =105
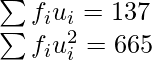
h = 30
Mean = ![]()
![]() = 105 + 30(137/300) = 118.7
= 105 + 30(137/300) = 118.7
![]()
= 900[(665/300) – (137/300)2]
= 1807.31
Standard Deviation = √var(x) = √1807.31 = 42.51
问题3.计算以下分布的AM和SD:
| Class: | 0-10 | 10-20 | 20-30 | 30-40 | 40-50 | 50-60 | 60-70 | 70-80 |
| Frequency: | 18 | 16 | 15 | 12 | 10 | 5 | 2 | 1 |
解决方案:
| CI | f | x | u = (x – A)/h | f × u | u2 | fu2 |
| 0 – 10 | 18 | 5 | -3 | -54 | 9 | 162 |
| 10 – 20 | 16 | 15 | -2 | -32 | 4 | 64 |
| 20 – 30 | 15 | 25 | -1 | -15 | 1 | 15 |
| 30 – 40 | 12 | 35 | 0 | 0 | 0 | 0 |
| 40 – 50 | 10 | 45 | 1 | 10 | 1 | 10 |
| 50 – 60 | 5 | 55 | 2 | 10 | 4 | 20 |
| 60 – 70 | 2 | 65 | 3 | 6 | 9 | 18 |
| 70 – 80 | 1 | 75 | 4 | 4 | 16 | 16 |
| 79 | -71 | 305 | ||||
Given: N = 79 and A =35
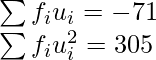
h = 10
Mean = ![]()
![]() = 35 + 10(-71/79) = 26.01
= 35 + 10(-71/79) = 26.01
![]()
= 100[(305/79) – (-71/79)2]
= 305.30
Standard Deviation = √var(x) = √305.30 = 17.47
问题4.一个学生分别获得100个观测值的平均值和标准差,分别为40和5.1。后来发现,一个观测值被错误地复制为50,正确的数字是40。找到正确的均值和SD
解决方案:
According to question, we have,
n = 100 , 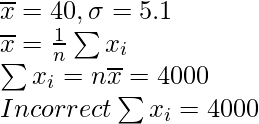
And, also
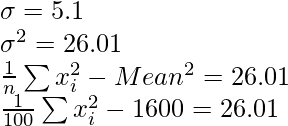
![]() = 1626.01 x 100
= 1626.01 x 100
Incorrected ![]() = 162601
= 162601
On replacing the incorrect observation of 50 by 40, we get,
Incorrect ![]() = 4000
= 4000
Corrected ![]() = 4000 – 50 + 40 = 3990
= 4000 – 50 + 40 = 3990
Incorrected ![]() = 162601
= 162601
Corrected ![]() = 162601 – 502 + 402 = 161701
= 162601 – 502 + 402 = 161701
Now, we have,
Corrected Mean = 39.90
Corrected Variance = (1/100)(Corrected ![]() ) – (Corrected mean)2
) – (Corrected mean)2
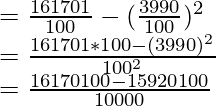
= 25
Corrected standard deviation = √25 = 5
问题5.计算以下分布的平均值,中位数和标准偏差:
| Class-interval | 31-35 | 36-40 | 41-45 | 46-50 | 51-55 | 56-60 | 61-65 | 66-70 |
| Frequency: | 2 | 3 | 8 | 12 | 16 | 5 | 2 | 3 |
解决方案:
| CI | Freq | Mid Value | ui | fiui | fiui2 |
| 31 – 35 | 2 | 33 | -4 | -8 | 32 |
| 36 – 40 | 3 | 38 | -3 | -9 | 27 |
| 41 – 45 | 8 | 43 | -2 | -16 | 32 |
| 46 – 50 | 12 | 48 | -1 | -12 | 12 |
| 51 – 55 | 16 | 53 | 0 | 0 | 0 |
| 56 – 60 | 5 | 58 | 1 | 5 | 5 |
| 61 – 65 | 2 | 63 | 2 | 4 | 8 |
| 66 – 70 | 2 | 68 | 3 | 6 | 18 |
| N = 50 | Total = – 30 | Total = 134 |
Now, using the given values, we have
Mean = 53 + 5 x (-30/50)
= 50
Variance = 25 x ((134/50) – (9/25)
= 58
Standard Deviation = √58
= 7.62
问题6.找到下面给出的频率分布的均值和方差:
| xi | 1 ≤ x < 3 | 3 ≤ x < 5 | 5 ≤ x < 7 | 7 ≤ x < 9 |
| fi | 6 | 4 | 5 | 1 |
解决方案:
The data can be converted to a continuous frequency distribution by subtracting 0.5 from lower limit and adding 0.5 to upper limit of each of the class interval.
Given: N = 16 and A = 5.5
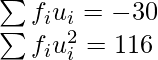 and h=1
and h=1
Mean = ![]()
![]() = 5.5 + 1((1/6) x (-30))
= 5.5 + 1((1/6) x (-30))
= 3.625
![]()
= 1 [((1/16) x 116) – ((1/16) x (-30)2]
= 3.74
问题7.下表显示了70罐咖啡的重量:
| Class Interval | fi | xi | ui | fiui | ui2 | fiui2 |
| 1 – 2 | 6 | 1.5 | -4 | -24 | 16 | 96 |
| 3 – 4 | 4 | 3.5 | -2 | -8 | 4 | 16 |
| 5 – 6 | 5 | 5.5 | 0 | 0 | 0 | 0 |
| 7 – 8 | 1 | 7.5 | 2 | 2 | 4 | 4 |
| N = 16 | Total = -30 | Total = 116 |
计算均值,方差和标准差。
解决方案:
| Weight (in grams) | 200-201 | 201-202 | 202-203 | 203-204 | 204-205 | 205-206 |
| Frequency | 13 | 27 | 18 | 10 | 1 | 1 |
Now, using the given values, we have
Mean = 203.5 + 2 x (-54/70)
= 201.9
Variance = 4 x (62/70) – (-54/70)
= 0.98
Standard Deviation = √0.98
= 0.099
问题8:100个观测值的平均值和标准偏差分别为40和10。如果在计算时错误地将两个观测值分别取为30和70而不是3和27,则找到正确的标准偏差。
解决方案:
Mean = 40
Standard Deviation = 10
n = 100
![]()
Corrected Sum = 4000 – 30 +70 + 3 + 27 = 3930
Corrected mean = 39.3
Variance = 100
![]()
Incorrect \sum x_i^2 = 170000
So, Corrected \sum x_i^2 = Incorrect ![]() – (Sum of squares of incorrect values) +
– (Sum of squares of incorrect values) +
(Sum of squares of corrected values)
Corrected![]() = 170000 – (900 + 4900) + (9+729)
= 170000 – (900 + 4900) + (9+729)
= 164938
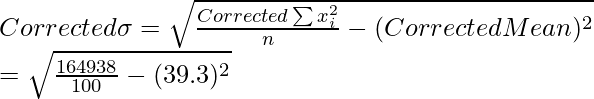
= 10.24
问题9.在计算10个读数的均值和方差时,一名学生错误地将52的读数用于正确的读数25。他获得的均值和方差分别为45和16。找到正确的均值和方差。
解决方案:
Mean = 45
Variance = 16
n = 10
![]()
So, Corrected Sum = 450 – 52 + 25 = 423
Corrected mean = 42.3
Variance = 16
1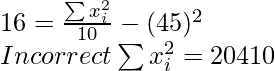
Corrected ![]() = Incorrect
= Incorrect ![]() – (Sum of squares of incorrect values) +
– (Sum of squares of incorrect values) +
(Sum of squares of corrected values)
Corrected ![]() = 20410 – 2704 + 625 = 18331
= 20410 – 2704 + 625 = 18331
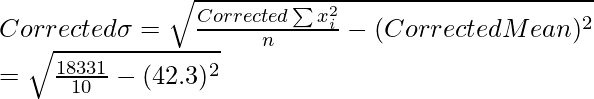
= 6.62
So, Corrected variance = 6.62 * 6.62 = 43.82
问题10.计算以下频率分布的均值,方差和标准偏差:
| CI | xi | fi | ui | fiui | fiui2 |
| 200 – 201 | 200.5 | 13 | -15 | -19.5 | 29.25 |
| 201 – 202 | 201.5 | 27 | -1 | -27 | 27 |
| 202 – 203 | 202.5 | 18 | -0.5 | -9 | 4.5 |
| 203 – 204 | 203.5 | 10 | 0 | 0 | 0 |
| 204 – 205 | 204.5 | 1 | 0.5 | 0.5 | 0.25 |
| 205 – 206 | 205.5 | 1 | 1 | 1 | 1 |
| N = 70 | Total = – 54 | Total = 62 |
解决方案:
| Class | 0-10 | 10-20 | 20-30 | 30-40 | 40-50 | 50-60 |
| Frequency | 11 | 29 | 18 | 4 | 5 | 3 |
Given:
Number of observations, N = 70 and A = 35
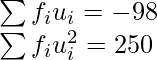
h = 10
Mean = ![]()
![]() = 35 + 10(-98/70) = -21
= 35 + 10(-98/70) = -21
![]()
= 100[(1/70) x 250 – (1/70) x (-98)2]
= 161
Standard Deviation = √var(x)
= √161
= 12.7