第11类RD Sharma解决方案–第32章统计–练习32.1

问题1.计算以下观察值的中位数的平均偏差：

(i)3011、2780、3020、2354、3541、4150、5000

(ii)38、70、48、34、42、55、63、46、54、44

(iii)34、66、30、38、44、50、40、60、42、51

(iv)22、24、30、27、29、31、25、28、41、42

(v)38、70、48、34、63、42、55、44、53、47

解决方案：

(i) 3011, 2780, 3020, 2354, 3541, 4150, 5000

Calculating Median (M) of the following observation:

Arranging numbers in ascending order,

2354, 2780, 3011, 3020, 3541, 4150, 5000

Median is the middle number of all the observations.

Therefore, Median = 3020 and n = 7

Calculating Mean Deviation:

$MD = \frac{1}{n}\sum_{i=1}^{n}|d_i|$

= 1/7 × 4546

= 649.42

Hence, Mean Deviation is 649.42.

(ii) 38, 70, 48, 34, 42, 55, 63, 46, 54, 44

Calculating Median (M) of the following observation:

Arranging numbers in ascending order,

34, 38, 42, 44, 46, 48, 54, 55, 63, 70

Median is the middle number of all the observations.

Here, the number of observations are even, 

therefore the Median = (46 + 48)/2 = 47

Median = 47 and n = 10

xi	|di| = |xi – 47|
38	9
70	23
48	1
34	13
42	5
55	8
63	16
46	1
54	7
44	3
Total	86

Calculating Mean Deviation:

$MD = \frac{1}{n}\sum_{i=1}^{n}|d_i|$

= 1/10 × 86

= 8.6

Hence, Mean Deviation is 8.6.

(iii) 34, 66, 30, 38, 44, 50, 40, 60, 42, 51

Calculating Median (M) of the following observation:

Arranging numbers in ascending order,

30, 34, 38, 40, 42, 44, 50, 51, 60, 66

Median is the middle number of all the observations.

Here, the number of observations are even, 

therefore the Median = (42 + 44)/2 = 43

Median = 43 and n = 10

xi	|di| = |xi – 43|
30	13
34	9
38	5
40	3
42	1
44	1
50	7
51	8
60	17
66	23
Total	87

Calculating Mean Deviation:

$MD = \frac{1}{n}\sum_{i=1}^{n}|d_i|$

= 1/10 × 87

= 8.7

Hence, Mean Deviation is 8.7.

(iv) 22, 24, 30, 27, 29, 31, 25, 28, 41, 42

Calculating Median (M) of the following observation:

Arranging numbers in ascending order,

22, 24, 25, 27, 28, 29, 30, 31, 41, 42

Median is the middle number of all the observations.

Here, the number of observations are even, 

therefore the Median = (28 + 29)/2 = 28.5

Median = 28.5 and n = 10

xi	|di| = |xi – 28.5|
22	6.5
24	4.5
30	1.5
27	1.5
29	0.5
31	2.5
25	3.5
28	0.5
41	12.5
42	13.5
Total	47

Calculating Mean Deviation:

$MD = \frac{1}{n}\sum_{i=1}^{n}|d_i|$

= 1/10 × 47

= 4.7

Hence, Mean Deviation is 4.7.

(v) 38, 70, 48, 34, 63, 42, 55, 44, 53, 47

Calculating Median (M) of the following observation:

Arranging numbers in ascending order,

34, 38, 43, 44, 47, 48, 53, 55, 63, 70

Median is the middle number of all the observation.

Here, the number of observations are even,

therefore the Median = (47 + 48)/2 = 47.5

Median = 47.5 and n = 10

xi	|di| = |xi – 47.5|
38	9.5
70	22.5
48	0.5
34	13.5
63	15.5
42	5.5
55	7.5
44	3.5
53	5.5
47	0.5
Total	84

Calculating Mean Deviation:

$MD = \frac{1}{n}\sum_{i=1}^{n}|d_i|$

= 1/10 × 84

= 8.4

∴ The Mean Deviation is 8.4.

问题2.计算以下数据的均值与均值的偏差：

(i)4，7，8，9，10，12，13，17

(ii)13、17、16、14、11、13、10、16、11、18、12、17

(iii)38、70、48、40、42、55、63、46、54、44

(iv)36、72、46、42、60、45、53、46、51、49

(v)57、64、43、67、49、59、44、47、61、59

解决方案：

(i) 4, 7, 8, 9, 10, 12, 13, 17

We know, Mean Deviation, 

$MD = \frac{1}{n}\sum_{i=1}^{n}|d_i|$

Where, |d_i| = |x_i – x|

So, let us assume x to be the mean of the given observation.

Now, x = [4 + 7 + 8 + 9 + 10 + 12 + 13 + 17]/8

= 80/8

= 10

Number of observations, n = 8

xi	|di| = |xi – 10|
4	6
7	3
8	2
9	1
10	0
12	2
13	3
17	7
Total	24

MD = 1/8 * 24

= 3

(ii) 13, 17, 16, 14, 11, 13, 10, 16, 11, 18, 12, 17

Since,

Mean Deviation, $MD = \frac{1}{n}\sum_{i=1}^{n}|d_i|$

Where, |d_i| = |x_i – x|

So, let us assume x to be the mean of the given observation.

x = [13 + 17 + 16 + 14 + 11 + 13 + 10 + 16 + 11 + 18 + 12 + 17]/12

= 168/12

= 14

Number of observations, n = 12

xi	|di| = |xi – 14|
13	1
17	3
16	2
14	0
11	3
13	1
10	4
16	2
11	3
18	4
12	2
17	3
Total	28

Now, 

MD = 1/12 × 28

= 2.33

(iii) 38, 70, 48, 40, 42, 55, 63, 46, 54, 44

We know that,

Mean Deviation, $MD = \frac{1}{n}\sum_{i=1}^{n}|d_i|$

Where, |d_i| = |x_i – x|

So, let us assume x to be the mean of the given observation.

x = [38 + 70 + 48 + 40 + 42 + 55 + 63 + 46 + 54 + 44]/10

= 500/10

= 50

Number of observations, n = 10

xi	|di| = |xi – 50|
38	12
70	20
48	2
40	10
42	8
55	5
63	13
46	4
54	4
44	6
Total	84

MD = 1/10 × 84

= 8.4

(iv) 36, 72, 46, 42, 60, 45, 53, 46, 51, 49

Mean Deviation, 

$MD = \frac{1}{n}\sum_{i=1}^{n}|d_i|$

Where, |d_i| = |x_i – x|

So, let us assume x to be the mean of the given observation.

x = [36 + 72 + 46 + 42 + 60 + 45 + 53 + 46 + 51 + 49]/10

= 500/10

= 50

Number of observations, n = 10

xi	|di| = |xi – 50|
36	14
72	22
46	4
42	8
60	10
45	5
53	3
46	4
51	1
49	1
Total	72

MD = 1/10 × 72

= 7.2

(v) 57, 64, 43, 67, 49, 59, 44, 47, 61, 59

Mean Deviation, 

$MD = \frac{1}{n}\sum_{i=1}^{n}|d_i|$

Where, |d_i| = |x_i – x|

So, let us assume x to be the mean of the given observation.

x = [57 + 64 + 43 + 67 + 49 + 59 + 44 + 47 + 61 + 59]/10

= 550/10

= 55

Number of observations, n = 10

xi	|di| = |xi – 55|
57	2
64	9
43	12
67	12
49	6
59	4
44	11
47	8
61	6
59	4
Total	74

MD = 1/10 × 74

= 7.4

问题3。 计算以下五个和七个成员的收入组与中位数的平均偏差：

xi	|di| = |xi – 3020|
3011	9
2780	240
3020	0
2354	666
3541	521
4150	1130
5000	1980
Total	4546

解决方案：

Dataset I : 

Since the data is arranged in ascending order,

4000, 4200, 4400, 4600, 4800

Median (Middle of ascending order observation) = 4400

Total observations, n = 5

Now, Mean Deviation, 

$MD = \frac{1}{n}\sum_{i=1}^{n}|d_i|$

xi	|di| = |xi – 4400|
4000	400
4200	200
4400	0
4600	200
4800	400
Total	1200

MD(I) = 1/5 × 1200

= 240

Dataset II :

Since the data is arranged in ascending order,

3800, 4000, 4200, 4400, 4600, 4800, 5800

Median (Middle of ascending order observation) = 4400

Total observations, n = 7

Now, Mean Deviation, 

$MD = \frac{1}{n}\sum_{i=1}^{n}|d_i|$

xi	|di| = |xi – 4400|
3800	600
4000	400
4200	200
4400	0
4600	200
4800	400
5800	1400
Total	3200

MD(II) = 1/7 × 3200

= 457.14

Therefore, the Mean Deviation of set 1, MD(I) is 240 and set 2, MD(II) is 457.14

问题4.商店中10根棒的长度(厘米)如下：

40.0、52.3、55.2、72.9、52.8、79.0、32.5、15.2、27.9、30.2

(i)找到与中位数的平均偏差。

(ii)还找到与均值的均值偏差。

解决方案：

(i) The mean deviation from the median

Arranging the data in ascending order,

15.2, 27.9, 30.2, 32.5, 40.0, 52.3, 52.8, 55.2, 72.9, 79.0

We know that,

$MD = \frac{1}{n}\sum_{i=1}^{n}|d_i|$

Since, the number of observations are even, 

therefore Median = (40 + 52.3)/2 = 46.15

Median = 46.15

Also, number of observations, n = 10

xi	|di| = |xi – 46.15|
40.0	6.15
52.3	6.15
55.2	9.05
72.9	26.75
52.8	6.65
79.0	32.85
32.5	13.65
15.2	30.95
27.9	19.25
30.2	15.95
Total	167.4

MD = 1/10 * 167.4

=16.74

(ii) Mean deviation from the mean also.

$MD = \frac{1}{n}\sum_{i=1}^{n}|d_i|$

Where, |d_i| = |x_i – x|

So, let us assume x to be the mean of the given observation.

Now, x = [40.0 + 52.3 + 55.2 + 72.9 + 52.8 + 79.0 + 32.5 + 15.2 + 27.9 + 30.2]/10

= 458/10

= 45.8

And, number of observations, n = 10

xi	|di| = |xi – 45.8|
40.0	5.8
52.3	6.5
55.2	9.4
72.9	27.1
52.8	7
79.0	33.2
32.5	13.3
15.2	30.6
27.9	17.9
30.2	15.6
Total	166.4

MD = 1/10 * 166.4

= 16.64

问题5.在问题1(iii)，(iv)，(v)中，找出介于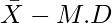$\bar{X}-M.D$和$\bar{X}+M.D$ ，其中MD是从均值的均值偏差。

解决方案：

(iii) 34, 66, 30, 38, 44, 50, 40, 60, 42, 51

We know that,

$MD = \frac{1}{n}\sum_{i=1}^{n}|d_i|$

Where, |d_i| = |x_i – x|

So, let us assume x to be the mean of the given observation.

x = [34 + 66 + 30 + 38 + 44 + 50 + 40 + 60 + 42 + 51]/10

= 455/10

= 45.5

And, number of observations, n = 10

xi	|di| = |xi – 45.5|
34	11.5
66	20.5
30	15.5
38	7.5
44	1.5
50	4.5
40	5.5
60	14.5
42	3.5
51	5.5
Total	90

MD = 1/10 × 90

= 9

Now,

$\overline{X}-M D = 45.5 - 9 = 36.5 \\ \overline{X}+MD = 45.5 + 9 = 54.5$

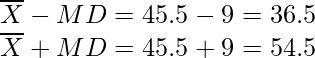
So, There are total 6 observation between $\overline{X}-MD$  and $\overline{X}+MD$

(iv) 22, 24, 30, 27, 29, 31, 25, 28, 41, 42

We know that,

$MD = \frac{1}{n}\sum_{i=1}^{n}|d_i|$

Where, |d_i| = |x_i – x|

So, let us assume x to be the mean of the given observation.

x = [22 + 24 + 30 + 27 + 29 + 31 + 25 + 28 + 41 + 42]/10

= 299/10

= 29.9

Also, number of observations, n = 10

xi	|di| = |xi –  29.9|
22	7.9
24	5.9
30	0.1
27	2.9
29	0.9
31	1.1
25	4.9
28	1.9
41	11.1
42	12.1
Total	48.8

MD = 1/10 × 48.8

= 4.88

And,

$\overline{X}-M D = 29.9 - 4.88 = 25.02 \\ \overline{X}+MD = 29.9 + 4.88 = 34.78$

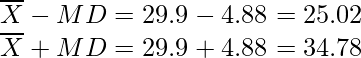
So, there are 5 observations in between. 

(v) 38, 70, 48, 34, 63, 42, 55, 44, 53, 47

We know that,

$MD = \frac{1}{n}\sum_{i=1}^{n}|d_i|$

Where, |d_i| = |x_i – x|

So, let us assume x to be the mean of the given observation.

x = [38 + 70 + 48 + 34 + 63 + 42 + 55 + 44 + 53 + 47]/10

= 494/10

= 49.4

Number of observations, n = 10

xi	|di| = |xi – 49.4|
38	11.4
70	20.6
48	1.4
34	15.4
63	13.6
42	7.4
55	5.6
44	5.4
53	3.6
47	2.4
Total	86.8

MD = = 1/10 × 86.8

= 8.68

Also, 

$\overline{X}-M D = 49.4 - 8.68 = 40.72 \\ \overline{X}+MD = 49.4 + 8.68 = 58.08$

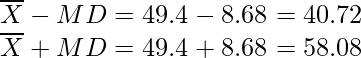
There are 6 observations in between. 

问题6.显示未分组数据的标准偏差的两个公式$\sigma = \sqrt{\frac{1}{n}\sum((x_i-\overline x)^{2}}$和$\sigma = \sqrt{\frac{1}{n}\sum((x_i^2 -\overline x)^{2}}$等价，在哪里$\overline x = \frac{1}{n}\sum x_i$

解决方案：

$\sigma = \sqrt{\frac{1}{n}\sum(x_i - \overline x)^2} \\ \sigma' = \sqrt{\frac{1}{n}\sum x_i^2 - \overline x^2} \\ (x_i - \overline x)^2 = x_i^2 + \overline x^2 - 2x_i\overline x \\ \sum 2x_i\overline x = 2\overline x\sum x_i = 2n\overline x^2 \\ \frac{1}{n}\sum(x_i - \overline x)^2 = \frac{\sum(x_i^2+\overline x^2-2x_i\overline x)}{n} \\= \frac{1}{n} \sum x_i^2 - \overline x^2$