问题1.找到以下数据的均值,方差和标准差:
(i)2、4、5、6、8、17
(ii)6,7,10,12,13,13,4,8,12
(iii)227、235、255、269、292、299、312、321、333、348
(iv)15、22、27、11、9、21、14,9
解决方案:
(i)
| x | d = (x – Mean) | d2 |
| 2 | -5 | 25 |
| 4 | -3 | 9 |
| 5 | -2 | 4 |
| 6 | -1 | 1 |
| 8 | 1 | 1 |
| 17 | 10 | 100 |
| Total = 42 | Total = 140 |
![]()
= 1/6[42] = 7
![]()
= 1/6[140] = 23.33
Standard deviation = √Var(x) = √23.33 = 4.8
(ii)
| x | d = (x – Mean) | d2 |
| 6 | -3 | 9 |
| 7 | -2 | 4 |
| 10 | 1 | 1 |
| 12 | 3 | 9 |
| 13 | 4 | 16 |
| 4 | -5 | 25 |
| 8 | -1 | 1 |
| 12 | 3 | 9 |
| Total = 72 | Total = 74 |
Mean = ![]()
= 1/8[72] = 9
![]()
= 1/8[74] = 9.25
Standard deviation = √Var(x) = √9.25 = 3.04
(iii)
|
xi |
di = xi – 299 |
di2 |
|
227 |
-72 |
5184 |
|
235 |
-64 |
4096 |
|
255 |
-44 |
1936 |
|
269 |
-30 |
900 |
|
292 |
-7 |
49 |
|
299 |
0 |
0 |
|
312 |
13 |
169 |
|
321 |
22 |
484 |
|
333 |
34 |
1156 |
|
348 |
49 |
2401 |
|
|
Total = -99 |
Total = 16375 |
Mean =![]() = 299 + (-99/10) = 289.1
= 299 + (-99/10) = 289.1
![]()
= 16375/10 – (-99/10)2
= 1637.5 – 98.01
= 1539.49
Standard deviation = √Var(x) = √1539.49 = 39.24
(iv)
| xi | di = xi – 15 | di2 |
|
15 |
0 |
0 |
|
22 |
7 |
49 |
|
27 |
12 |
144 |
|
11 |
-4 |
16 |
|
9 |
-6 |
36 |
|
21 |
6 |
36 |
|
14 |
-1 |
1 |
|
9 |
-6 |
36 |
|
|
Total = 8 |
Total = 318 |
Mean =![]() = 15 + 8/8 = 16
= 15 + 8/8 = 16
![]()
= 318/8 – 1 = 38.75
Standard deviation = √Var(x) = √38.75 = 6.22
问题2。20个观察值的方差是4。如果每个观察值乘以2,则求出结果观察值的方差。
解决方案:
Given: n = 20, and ![]()
Now multiply each observation by 2, we get
Suppose X = 2x be the new data.
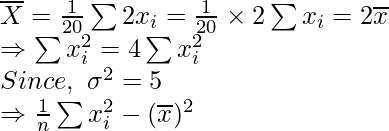 =5
=5
So, for the new data, we have
![]()
= 4 × 5
= 20
问题3. 15个观察值的方差是4。如果每个观察值增加9,则求出结果观察值的方差。
解决方案:
Given: n = 15, and ![]()
Now increase each observation by 9, we get
Suppose X = x + 9 be the new data.
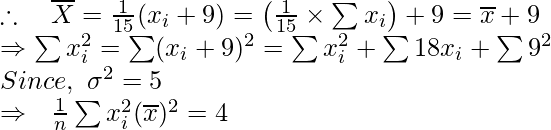
So for the new data:
![Rendered by QuickLaTeX.com \sigma^2=\frac{1}{n}\sum X_i^2-(\overline{X})^2=\frac{1}{15}(\sum x_i^2+\sum18x_i+\sum9^2)-(\overline{x}+9)^2\\ =\frac{1}{15}\sum x_i^2+15\sum18x_i+\frac{1}{15}\sum9^2-(9)^2-(18\overline{x})-(\overline{x})^2\\ =\left[\frac{1}{15}\sum x_i-(\overline{x})^2\right]+\left[\frac{1}{15}\sum18x_i-(18\overline{x})\right]+\left[\frac{1}{15}\sum9^2-(9)^2\right]](https://mangdo-1254073825.cos.ap-chengdu.myqcloud.com//front_eng_imgs/geeksforgeeks2021/Class%2011%20RD%20Sharma%20Solutions%20%E2%80%93%20Chapter%2032%20Statistics%20%E2%80%93%20Exercise%2032.4_13.jpg)
![Rendered by QuickLaTeX.com =\left[\frac{1}{15}\sum x_i^2-(\overline{x})^2\right]+\left[18\times\frac{1}{15}\sum x_i-(18\overline{x})\right]+\left[\frac{1}{15}\times15\times(9)^2-(9)^2\right]\\ \frac{1}{15}\sum x_i^2-(\overline{x})^2](https://mangdo-1254073825.cos.ap-chengdu.myqcloud.com//front_eng_imgs/geeksforgeeks2021/Class%2011%20RD%20Sharma%20Solutions%20%E2%80%93%20Chapter%2032%20Statistics%20%E2%80%93%20Exercise%2032.4_14.jpg)
= 4
问题4:5个观察值的平均值为4.4,其方差为8.24。如果三个观测值分别为1、2和6,请查找其他两个观测值。
解决方案:
Let us considered the other two observations are x and y
Given: The mean of 5 observations is 4.4 and their variance is 8.24
So,
Mean = 1 + 2 + 6 + x + y = 5 × 4.4
= x + y = 13
Variance = [(1 – 4.4)2 + (2 – 4.4)2 + (6 – 4.4)2 + (x – 4.4)2 + (y – 4.4)3]
11.56 + 5.76 + 2.56 + (x – 4.4)2 + (y – 4.4)2 = 41.2
(x – 4.4)2 + (y – 4.4)2 = 21.32
On solving this equation, we get
(x – 4.4)2 + (13 – x – 4.4)2 = 21.32
(x – 4.4)2 + (8.6 – x)2 = 21.32
x2 – 8.8x + 19.36 + 73.96 – 17.2x + x2 = 21.32
2x2 – 26x + 72 = 0
x2 – 13x + 36 = 0
(x – 4)(x – 9) = 0
x = 4 or x = 9
So, the other two observation are 4 and 9.
问题5. 6个观测值的平均值和标准偏差分别为8和4。如果每个观察值都乘以3,则找到结果观察值的新均值和新标准差。
解决方案:
Given: Mean of 6 observations = 8
Standard Deviation of 6 observation = 4
k = 3
So, let us considered mean and Standard Deviation of the observation are ![]() and
and ![]()
then the mean and Standard Deviation of the observation multiplied by a constant ‘k’ are

So, the new mean = 8 × 3 = 24
New Standard Deviation = 4 × 3 = 12
问题6:8个观测值的均值和方差分别为9和9.25。如果六个观测值分别为6、7、10、12、12和13,则找到其余两个观测值。
解决方案:
Given: Mean of 8 observations = 9
Standard Deviation of 8 observations = 9.25
Observations = 6, 7, 10, 12, 12, and 13
So, let us considered the other two observations are x and y
Mean = (6 + 7 + 10 + 12 + 12 + 13 + x + y)/8 = 9
= 60 + x + y =72
= x + y = 12 -(1)
Variance = 1/8(62 + 72 + 102 + 122 + 122 + 132 + x2 + y2) – (81)2 = 9.25
= 642 + x2 + y2 = 722
= x2 + y2 = 80 -(2)
Now, (x + y)2 + (x – y)2 = 2(x2 + y2)
= 144 + (x – y)2 = 2 × 80
= (x – y)2 = 16
= x – y = ±4
If x – y = 4, then x + y = 12 and x – y = 4
So, x = 8, y = 4
If x – y = -4 then x + y = 12 and x – y = -4
So, x = 4, y = 8
Hence, the remaining two observations are 4 and 8.
问题7:对于一组200名候选人,发现分数的平均值和标准偏差分别为40和15。后来发现,分数43和35分别被误读为34和53。找到正确的平均值和标准偏差。
解决方案:
Given: n = 200, 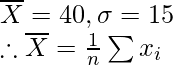
![]() = 200 × 40 = 8000
= 200 × 40 = 8000
Corrected ![]() = Incorrect
= Incorrect ![]() – (sum of incorrect values) + (sum of correct values)
– (sum of incorrect values) + (sum of correct values)
= 8000 – 34 – 53 + 43 + 35 = 7991
Corrected mean = ![]()
= 7991/200 = 39.955
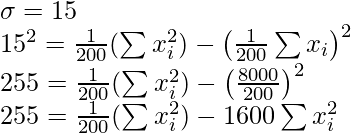
= 200 × 1825 = 365000
Incorrect ![]() = 36500
= 36500
Corrected ![]() = (incorrect
= (incorrect ![]() ) – (sum of squares of incorrect value) +
) – (sum of squares of incorrect value) +
(sum of squares of correct values)
= 365000 – (34)2 – 532 + (43)2 + 352 = 364109
So, Corrected 
= 14.97
问题8.一个学生错误地将50个观测值而不是40个观测值计算为一个观测值,分别计算出100个观测值的平均值和标准偏差为40和5.1。正确的均值和标准差是多少?
解决方案:
Given: n = 100, 
![]() = 100 × 1626.01 = 162601
= 100 × 1626.01 = 162601
Incorrect = 162601
Corrected = (incorrect ) – (sum of squares of incorrect values) + (sum of squares of correct values)
= 162601 – (50)2 + (40)2 = 161701
So, Corrected 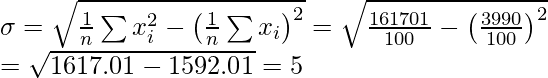
问题9:20个观测值的平均值和标准偏差分别为10和2。重新检查后,发现观察值8不正确。在以下每种情况下,计算正确的均值和标准差:
(i)如果遗漏了错误的项目。
(ii)如果被12取代。
解决方案:
Given: n = 20, 
(i) If we remove 8 from the given observation then 19 observation are left.
Now, Incorrect ![]() = 200
= 200
⇒ Corrected ![]() + 8 = 200
+ 8 = 200
⇒ Corrected ![]() = 192
= 192
and,
⇒ Incorrect ![]() = 2080
= 2080
⇒ Corrected ![]() + 82 = 2080
+ 82 = 2080
⇒ Corrected ![]() = 2080 – 64
= 2080 – 64
⇒ Corrected ![]() = 2016
= 2016
Therefore,
Corrected mean = ![]() = 10.10
= 10.10
⇒ So, corrected variance = ![]()
= 2016/19 – (192/19)2
= (38304 -36864)/361
= 1440/361
So, the corrected standard deviation = ![]() = 1.997
= 1.997
(ii) If we replace the incorrect observation(i.e., 8) by 12
Given: Incorrect ![]() = 200
= 200
Therefore, Corrected ![]() = 200 – 8 + 12 = 204
= 200 – 8 + 12 = 204
Incorrect ![]() = 2080
= 2080
Therefore, Corrected ![]() = 2080 – 82 + 122 = 2160
= 2080 – 82 + 122 = 2160
Now, Corrected mean = 204/20 = 10.2
Corrected variance = ![]()
= 2016/20 – (204/20)2
= ![]()
= ![]()
= 1584/400
So, the corrected standard deviation = ![]()
= 19.899/10 = 1.9899
问题10:100个观察组的平均值和标准差分别为20和3。后来发现三个观测值是不正确的,分别记录为21、21和18。如果省略了不正确的观测值,则求出均值和标准差。
解决方案:
(i) Given: n = 100, ![]()
Mean = 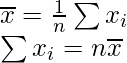
= 20 × 100 = 2000
Incorrect ![]() = 2000
= 2000
and,

Incorrect ![]() = 40900.
= 40900.
When the incorrect observations 21, 21, 18 are removed from the data
then the total number of observation are n = 97
Now,
Incorrect ![]() = 2000
= 2000
Corrected ![]() = 2000 – 21 – 21 – 18 = 1940
= 2000 – 21 – 21 – 18 = 1940
and,
Incorrect ![]() = 40900
= 40900
Corrected ![]() = 40900 – 212 – 212 – 182
= 40900 – 212 – 212 – 182
= 40900 – 1206
= 39694
Therefore, Corrected mean = 1940/97 = 20
Corrected variance = ![]()
= (39694/97) – (20)2 = 409.22 – 400 = 9.22
So, the corrected standard deviation = √9.22 = 3.04
问题11:表明未分组数据的标准差的两个公式
等价,在哪里
解决方案:
Given:

On dividing both the sides by n we get,
![]()
Now, taking square root on both the sides, we get
![]()