第12类RD Sharma解-第32章随机变量的均值和方差–练习32.1 |套装1

问题1.随机变量概率的以下哪些分布是它们的概率分布?

一世。

X	3	2	1	0	-1
P(X)	0.3	0.2	0.4	0.1	0.05

解决方案：

We know that the sum of probability distribution is always 1.

Sum of probabilities (P(X))=P(X=3)+P(X=2)+P(X=1)+P(X=0)+P(X=-1)

                                          =0.3+0.2+0.4+0.1+0.05=1.05>1

The sum of probability distribution is not equal to 1. Hence, it is not the probability distribution of the given random variables.

ii。

X	0	1	2
P(X)	0.6	0.4	0.2

解决方案：

Sum of probabilities (P(X))=P(X=0)+P(X=1)+P(X=2)

                                          =0.6+0.4+0.2=1.2>1

The sum of probability distribution is not equal to 1. Hence, it is not the probability distribution of the given random variables.

iii。

X	0	1	2	3	4
P(X)	0.1	0.5	0.2	0.1	0.1

解决方案：

Sum of probabilities (P(X))=P(X=0)+P(X=1)+P(X=2)+P(X=3)+P(X=4)

                                          =0.1+0.5+0.2+0.1+0.1=1

The sum of probability distribution is equal to 1. Hence, it is the probability distribution of the given random variables.

iv。

X	0	1	2	3
P(X)	0.3	0.2	0.4	0.1

解决方案：

Sum of probabilities (P(X))=P(X=0)+P(X=1)+P(X=2)+P(X=3)

                                          =0.3+0.2+0.4+0.1=1

The sum of probability distribution is equal to 1. Hence, it is the probability distribution of the given random variables.

问题2.随机变量X具有以下概率分布：

X	-2	-1	0	1	2	3
P(X)	0.1	k	0.2	2k	0.3	k

找出k的值。

解决方案：

We know that the sum of probability distribution is always 1.

Sum of probability distribution (P(X))=P(X=-2)+P(X=-1)+P(X=0)+P(X=1)+P(X=2)+P(X=3)=1

            =>0.1+k+0.2+2k+0.3+k=1

            =>0.6+4k=1

            =>4k=1-0.6

            =>k=0.1

问题3.随机变量X具有以下概率分布：

X	0	1	2	3	4	5	6	7	8
P(X)	a	3a	5a	7a	9a	11a	13a	15a	17a

一世。查找a的值。

解决方案：

We know that the sum of probability distribution is always 1.

Sum of probability distribution (P(X))=P(X=0)+P(X=1)+P(X=2)+P(X=3)+P(X=4)+P(X=5)+P(X=6)+P(X=7)+P(X=8)=1

         =>a+3a+5a+7a+9a+11a+13a+15a+17a=1

         =>81a=1

         =>a=1/81

ii。求P(X <3)。

解决方案：

P(X<3)=P(X=0)+P(X=1)+P(X=2)

           =1/81+3/81+5/81

           =9/81=1/9

iii。找出P(X> = 3)。

解决方案：

P(X>=3)=P(X=3)+P(X=4)+P(X=5)+P(X=6)+P(X=7)+P(X=8)

              =7/81+9/81+11/81+13/81+15/81+17/81

               =72/81=8/9

iv。求P(0

P(0

                =3/81+5/81+7/81+9/81

                =24/81=8/27=0.296

问题4.1。随机变量X的概率分布函数为

X	0	1	2
P(X)	3c3	4c-10c2	5c-1

其中c> 0。查找c。

解决方案：

We know that the sum of probability distributions of a random variable is always 1.

=>3c³ +4c-10c²+5c-1=1

=>3c³-10c²+9c-2=0

Let c=1

3(1)-10(1)+9(1)-2=12-12=0

Therefore c=1.

By horn’s method :

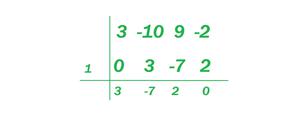
We get a quadratic equation : 3c²-7c+2=0

From this quadratic equation we get,

=>3c²-6c-c+2=0

=>3c(c-2)-1(c-2)=0

=>(3c-1)(c-2)=0

=>3c-1=0;    c-2=0

=>3c=1;     c=2

=>c=1/3;    c=2;    c=1

We know that a single probability distribution cannot be 1 or more than one. So we take c=1/3.

Therefore, c=1/3.

问题4.2。求P(X <2)。

解决方案：

P(X<2)=P(X=0)+P(X=1)

            =3(1/3)³+4(1/3)-10(1/3)²

            =1/9+4/3-10/9

            =1/3=0.33

问题4.3。求P(1

解决方案：

P(1

                  =5(1/3)-1

                  =5/3-1

                 =2/3=0.66

问题5.令X是一个随机变量，它假设值x ₁ ，x ₂ ，x ₃ ，x ₄使得2P(X = x ₁ )= 3P(X = x ₂ )= P(X = x ₃ )= 5P (X ＝ x ₄ )。求出X的概率分布。

解决方案：

Sum of probability distributions= P(X=x₁)+P(X=x₂)+P(X=x₃)+P(X=x₄)=1

Given,

      2P(X=x1)=3P(X=x2)=P(X=x3)=5P(X=x4)

=>P(X=x₂)=2/3P(X=x₁) ; P(X=x₃)=2/1(P(X=x₁) ; P(X=x₄)=2/5(P(X=x₁)

=>P(X=x₁)+2/3(P(X=x₁)+2/1(P(X=x₁)+2/5(P(X=x₁)=1

=>61/15(P(X=x₁)=1

=>P(X=x₁)=15/61=0.24

=>P(X=x₂)=2/3(P(X=x₁)

                 =2/3(15/61)

                 =10/61=0.16

=>P(X=x₃)=2/1(P(X=x₁)

                 =2(15/61)

                 =30/61=0.49

=>P(X=x₄)=2/5(P(X=x₁))

                 =2/5(15/61)

                 =6/61=0.09

问题6.随机变量X的取值为0,1,2和3，使得：P(X = 0)= P(X> 0)= P(X <0); P(X = -3)= P(X = -2)= P(X = -1); P(X = 1)= P(X = 2)= P(X = 3)。获得X的概率分布。

解决方案：

We know that the sum of probability distributions is equal to 1.

=>P(X=0)+P(X>0)+P(X<0)=1

Given,

P(X=0)=P(X>0)=P(X<0)

=>P(X=0)+P(X=0)+P(X=0)=1

=>3P(X=0)=1

=>P(X=0)=1/3

=>P(X>0)=1/3

=>P(X=1)+P(X=2)+P(X=3)=1/3

Given,

P(X=1)=P(X=2)=P(X=3)

=>3P(X=1)=1/3

=>P(X=1)=1/9 ; P(X=2)=1/9 ; P(X=3)=1/9

=>P(X<0)=1/3

=>P(X=-1)+P(X=-2)+P(X=-3)=1/3

Given,

P(X=-3)=P(X=-2)=P(X=-1)

=>3P(X=-1)=1/3

=>P(X=-1)=1/9 ; P(X=-2)=1/9 ; P(X=-3)=1/9

问题7：两张纸牌是从52张纸牌中随机抽取的一组中抽出的。找到王牌数的概率分布。

解决方案：

Given that two cards are drawn from a well shuffled deck of 52 cards.

Then the random variables for the probability distribution of the number of aces could be 

i. No ace is drawn

ii. One ace is drawn

iii. Two aces are drawn

i. No ace is drawn:

P(X=0)=^52-4C₂/⁵²C₂

              =⁴⁸C₂/⁵²C₂

          =48!/2!x46!/52!/2!x50!

          =48×47/52×51

          =188/221

          =0.85

ii. One ace is drawn:

P(X=1)=⁴C₁x⁴⁸C₁/⁵²C₂

           =4x48x2/52×51

           =32/221

           =0.14

iii. Two aces are drawn:

P(X=2)=⁴C₂/⁵²C₂

           =6×2/52×51

           =1/221

           =0.004

问题8.掷三枚硬币时，求出正面数目的概率分布。

解决方案：

Given that three coins are tossed simultaneously.

Then the random variables for the probability distribution of the number of heads could be,

i. No heads

ii. One head

iii. Two heads

iv. Three heads

i. No heads:

P(X=0)=¹C₁x¹C₁x¹C₁/ ²C₁x ²C₁x ²C₁

           =1x1x1/2x2x2

           =1/8

           =0.125

ii. One head:

P(X=1)=¹C₁+¹C₁+¹C₁/8

          =1+1+1/8

          =3/8

          =0.37

iii. Two heads:

P(X=2)=¹C₁+¹C₁+¹C₁/8

           =3/8

           =0.37

iv. Three heads:

P(X=3)=1/8

           =0.125

问题9.从一张经过充分洗净的52张纸牌中同时抽出4张纸牌。找到王牌数的概率分布。

解决方案：

Given that four cards are drawn simultaneously from a well shuffled pack of 52 cards.

Then the random variables for the probability distribution of the number of aces drawn could be,

i. No aces

ii. One ace

iii. Two aces

iv. Three aces

v. Four aces

i. No aces

P(X=0)=⁴⁸C₄/ ⁵²C₄

           =48x47x46x45/49x50x51x52

           =0.71

ii. One ace

P(X=1)=⁴C₁x⁴⁸C₃/⁵²C₄

           =4x48x47x46x4/49x50x51x52

           =0.25

iii. Two aces

P(X=2)= ⁴C₂x⁴⁸C₂/ ⁵²C₄

           =6x48x47x12/49x50x51x52

           =0.024

iv. Three aces

P(X=3)= ⁴C₃x⁴⁸C₁/⁵²C₄

           = 4x48x24/49x50x51x52

           = 0.0007

v. Four aces

P(X=4)=⁴C₄/ ⁵²C₄

           =1/ 270725

           =0.000003694

问题10.一个袋子包含4个红色和6个黑色的球。随机抽出三个球。求出红球数量的概率分布。

解决方案：

Given that three balls are drawn at random from a bag.

Then the value of random variable for the probability distribution of number of red balls could be,

i. No red ball

ii. One red ball

iii. Two red balls

iv. Three red balls

i. No red balls:

P(X=0)=⁶C₃/¹⁰C₃

           =6x5x4/10x9x8

           =1/6=0.16

ii. One red ball:

P(X=1)=⁶C₂x⁴C₁/¹⁰C₃

           =6x5x4x3/10x9x8

           =1/2=0.5

iii. Two red balls:

P(X=2)=⁶C₁x⁴C₂/¹⁰C₃

           =6x4x3x3/10x9x8

           =3/10=0.3

iv. Three red balls:

P(X=3)=⁴C₃/¹⁰C₃

           =4x3x2/10x9x8

           =1/30=0.03

问题11.五个有缺陷的芒果与十五个好芒果意外地混合在一起。从这批中随机抽取四个芒果。求出芒果缺陷数量的概率分布。

解决方案：

Given that five defective mangoes are mixed with 15 good ones.

Then the values of random variable for the probability distribution could be,

i. No defective

ii. One defective

iii. Two defective

iv. Three defective

v. Four defective

i. No defective:

P(X=0)=¹⁵C₄/²⁰C₄

           =15x14x13x12/20x19x18x17

           =91/323=0.28

ii. One defective:

P(X=1)=¹⁵C₃x⁵C₁/²⁰C₄

           =15x14x13x5x4/20x19x18x17

           =455/969=0.469

iii. Two defective:

P(X=2)=¹⁵C₂x⁵C₂/²⁰C₄

           =15x14x5x4x6/20x19x18x17

           =70/323=0.21

iv. Three defective:

P(X=3)=¹⁵C₁x⁵C₃/²⁰C₄

           =15x5x4x3x4/20x19x18x17

           =10/323=0.03

v. Four defective:

P(X=4)=⁵C₄/²⁰C₄

           =5x4x3x2/20x19x18x17

           =1/969=0.001

问题12：将两个骰子扔在一起，并记下出现在上面的数字。 X表示两个数字的总和。假设所有36个结果均具有相同的可能性，那么X的概率分布是多少?

解决方案：

Given that two dice are thrown simultaneously.

Then the outcomes would be as follows:

(1,1) ; (1,2) ; (1,3) ; (1,4) ; (1,5) ; (1,6) ;

(2,1) ; (2,2) ; (2,3) ; (2,4) ; (2,5) ; (2,6) ;

(3,1) ; (3,2) ; (3,3) ; (3,4) ; (3,5) ; (3,6) ;

(4,1) ; (4,2) ; (4,3) ; (4,4) ; (4,5) ; (4,6) ;

(5,1) ; (5,2) ; (5,3) ; (5,4) ; (5,5) ; (5,6) ;

(6,1) ; (6,2) ; (6,3) ; (6,4) ; (6,5) ; (6,6) 

The values of the random variable could be: 2,3,4,5,6,7,8,9,10,11,12

P(X=2)=1/36=0.02

P(X=3)=2/36=1/18=0.05

P(X=4)=3/36=1/12=0.08

P(X=5)=4/36=1/9=0.11

P(X=6)=5/36=0.13

P(X=7)=6/36=1/6=0.16

P(X=8)=5/36=0.13

P(X=9)=4/36=1/9=0.11

P(X=10)=3/36=1/12=0.08

P(X=11)=2/36=1/18=0.05

P(X=12)=1/36=0.02

问题13：一个班级有15名学生，年龄分别为14,17,15,14,21,19,20,16,18,17,20,17,16,19和20岁。以这样一种方式选择一个学生，使得每个人都有相同的被选中机会，并记录所选学生的年龄X。随机变量X的概率分布是多少?

解决方案：

Given that the students are selected without any bias.

Then the values of the random variable X could be: 14,15,16,17,18,19,20,21

P(X=14)=2/15=0.13

P(X=15)=1/15=0.06

P(X=16)=2/15=0.13

P(X=17)=3/15=0.2

P(X=18)=1/15=0.06

P(X=19)=2/15=0.13

P(X=20)=3/15=0.2

P(X=21)=1/15=0.06

问题14：五个有缺陷的螺栓意外地与二十个优质的螺栓混合在一起。如果从该批次中随机抽出四个螺栓，请找到有缺陷螺栓数量的概率分布。

解决方案：

Given that five defective bolts are mixed with 20 good ones.

Then the values of random variable for the probability distribution would be,

i. No defective

ii. One defective

iii. Two defective

iv. Three defective

v. Four defective

i. No defective:

P(X=0)=²⁰C₄/²⁵C₄

           =20x19x18x17/25x24x23x22

           =969/2530=0.38

ii. One defective:

P(X=1)=²⁰C₃x⁵C₁/²⁵C₄

           =20x19x18x5x4/25x24x23x22

           =114/253=0.45

iii. Two defective:

P(X=2)=²⁰C₂x⁵C₂/²⁵C₄

           =20x19x5x4x6/25x24x23x22

           =38/253=0.15

iv. Three defective:

P(x=3)=²⁰C₁x⁵C₂/²⁵C₄

           =20x5x4x4x3/25x24x23x22

           =4/253=0.015

v. Four defective:

P(X=4)=⁵C₄/²⁵C₄

           =5x4x3x2/25x24x23x22

           =1/2530=0.0004

问题15.从洗净后的52张纸牌中依次抽出两张纸牌，并进行替换。找到王牌数的概率分布。

解决方案：

Given that two cards are drawn with replacement from well shuffled pack of 52 cards.

Then the values of random variable for the probability distribution could be,

i. No ace

ii. One ace

iii. Two aces

i. No ace:

P(X=0)=(48/52 )x(48/52)

           =144/169=0.85

ii. One ace:

P(X=1)=(48/52)x(4/52)+(4/52)x(48/52)

           =24/169=0.14

iii. Two aces:

P(X=2)=(4/52)x(4/52)

           =1/169=0.005