11类RD Sharma解决方案–第29章限制–练习29.4 |套装1

评估以下限制：

问题1. Lim _x→0 {√(1 + x + x ² )– 1} / x。

解决方案：

We have, Lim_x→0{√(1 + x + x²) – 1}/x

Find the limit of the given equation when x =>0. 

When we put x = 0, this expression takes the form of 0/0.

So, on rationalizing the given equation we get

= $Lim_{x→0}\frac{\sqrt{(1 + x + x^2)} - 1}{x}\times \frac{\sqrt{1 + x + x^2}+1}{\sqrt{1 + x + x^2}+1}$

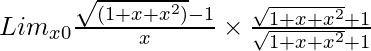
= Lim_x→0(1 + x + x² – 1)/{x√(1 + x + x²) + 1}

= Lim_x→0{x(x + 1)}/{x√(1 + x + x²) + 1}

= Lim_x→0(x + 1)/{√(1 + x + x²) + 1}

Now put x = 0, we get

= (0 + 1)/{√(1 + 0 + 0) + 1}

= 1/2

问题2. Lim _x→0 (2x)/ {√(a + x)–√(a – x)}

解决方案：

We have, Lim_x→0(2x)/{√(a + x) – √(a – x)}

Find the limit of the given equation when x =>0. 

When we put x = 0, this expression takes the form of 0/0.

So, on rationalizing the given equation we get

= $Lim_{x→0}\frac{(2x)}{\sqrt{a + x} - \sqrt{a - x}} \times \frac{\sqrt{a + x} + \sqrt{a - x}}{\sqrt{a + x} + \sqrt{a - x}}$

= Lim_x→0[2x{√(a + x) + √(a – x)}]/{(a + x) – (a – x)}

= Lim_x→0[2x{√(a + x) + √(a – x)}]/2x

= Lim_x→0{√(a + x) + √(a – x)}

Now put x = 0, we get

= √a + √a

= 2√a

问题3. Lim _x→0 {√(a ² + x ² )– a} / x ²

解决方案：

We have, Lim_x→0{√(a² + x²) – a}/x²

Find the limit of the given equation when x =>0. 

When we put x = 0, this expression takes the form of 0/0.

So, on rationalizing the given equation we get

= $Lim_{x→0}\frac{{\sqrt{(a^2 + x^2)} - a}}{x^2} \times \frac{\sqrt{(a^2 + x^2)} + a}{\sqrt{(a^2 + x^2)} + a}$

= Lim_x→0(a² + x² – a²)/[x²{√(a² + x²) + a}]

= Lim_x→0(x²)/[x²{√(a² + x²) + a}]

= Lim_x→0(1)/{√(a² + x²) + a}

Now put x = 0, we get

= 1/(a + a)

= 1/2a

问题4. Lim _x→0 {√(1 + x)–√(1 – x)} / 2x

解决方案：

We have, Lim_x→0{√(1 + x) – √(1 – x)}/2x

Find the limit of the given equation when x =>0. 

When we put x = 0, this expression takes the form of 0/0.

So, on rationalizing the given equation we get

= $Lim_{x→0}\frac{\sqrt{(1 + x)} - \sqrt{(1 - x)}}{2x} \times \frac{\sqrt{(1 + x)} + \sqrt{(1 - x)}}{\sqrt{(1 + x)} + \sqrt{(1 - x)}}$

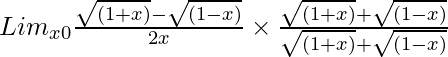
= Lim_x→0(1 + x – 1 + x)/[2x{√(1 + x) + √(1 – x)}]

= Lim_x→0(2x)/[2x{√(1 + x) + √(1 – x)}]

= Lim_x→0(1)/{√(1 + x) + √(1 – x)}

Now put x = 0, we get

= 1/(1 + 1)

= 1/2

问题5. Lim _x→2 {√(3 – x)– 1} /(2 – x)

解决方案：

We have, Lim_x→2{√(3 – x) – 1}/(2 – x)

Find the limit of the given equation

When we put x = 2, this expression takes the form of 0/0.

So, on rationalizing the given equation we get

= $Lim_{x→2}\frac{\sqrt{(3 - x)} - 1}{(2 - x)} \times \frac{\sqrt{(3 - x)} + 1}{\sqrt{(3 - x)} + 1}$

= Lim_x→2{(3 – x) – 1}/[(2 – x){√(3 – x) + 1}]

= Lim_x→2(2 – x)/[(2 – x){√(3 – x) + 1}]

= Lim_x→2(1)/{√(3 – x) + 1}

Now put x = 2, we get

= 1/{√(3 – 2) + 1}

= 1/(1 + 1)

= 1/2

问题6. Lim _x→3 (x – 3)/ {√(x – 2)–√(4 – x)}

解决方案：

We have, Lim_x→3(x – 3)/{√(x – 2) – √(4 – x)}

Find the limit of the given equation

When we put x = 3, this expression takes the form of 0/0.

So, on rationalizing the given equation we get

= $Lim_{x→3}\frac{(x - 3)}{\sqrt{(x - 2)} - \sqrt{(4 - x)}} \times \frac{\sqrt{(x - 2)} + \sqrt{(4 - x)}}{\sqrt{(x - 2)} + \sqrt{(4 - x)}}$

= Lim_x→3[(x – 3){√(x – 2) + √(4 – x)}]/{(x – 2) – (4 – x)}

= Lim_x→3[(x – 3){√(x – 2) + √(4 – x)}]/{2(x – 3)}

= Lim_x→3{√(x – 2) + √(4 – x)}/2

Now put x = 3, we get

= {√(3 – 2) + √(4 – 3)}/2

= (1 + 1)/2

= 1

问题7. Lim _x→0 (x)/ {√(1 + x)–√(1 – x)}

解决方案：

We have, Lim_x→0(x)/{√(1 + x) – √(1 – x)}

Find the limit of the given equation

When we put x = 0, this expression takes the form of 0/0.

So, on rationalizing the given equation we get

= $Lim_{x→0}\frac{x}{\sqrt{(1 + x)} - \sqrt{(1 - x)}} \times \frac{\sqrt{(1 + x)} + \sqrt{(1 - x)}}{\sqrt{(1 + x)} + \sqrt{(1 - x)}}$

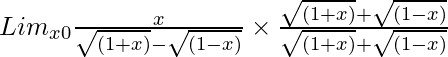
= Lim_x→0[x{√(1 + x) + √(1 – x)}]/{(1 + x) – (1 – x)}

= Lim_x→0[x{√(1 + x) + √(1 – x)}]/(2x)

= Lim_x→0{√(1 + x) + √(1 – x)}/(2)

Now put x = 0, we get

= (√1 + √1)/2

= 2/2

= 1

问题8. Lim _x→1 {√(5x – 4)–√x} /(x – 1)

解决方案：

We have, Lim_x→1{√(5x – 4) – √x}/(x – 1)

Find the limit of the given equation

When we put x = 1, this expression takes the form of 0/0.

So, on rationalizing the given equation we get

= $Lim_{x→1}\frac{\sqrt{(5x - 4)} - \sqrt{x}}{(x - 1)} \times \frac{\sqrt{(5x - 4)} + \sqrt{x}}{\sqrt{(5x - 4)} + \sqrt{x}}$

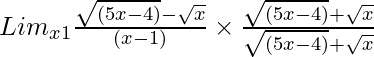
= Lim_x→1{5x – 4 – x}/[(x – 1){√(5x – 4) + √x}]

= Lim_x→1{4(x – 1)}/[(x – 1){√(5x – 4) + √x}]

= Lim_x→1(4)/{√(5x – 4) + √x}

Now put x = 1, we get

= 4/{√(5 – 4) + √1}

= 4/(1 + 1)

= 2

问题9. Lim _x→1 (x – 1)/ {√(x ² + 3)– 2}

解决方案：

We have, Lim_x→1(x – 1)/{√(x² + 3) – 2}

Find the limit of the given equation

When we put x = 1, this expression takes the form of 0/0.

So, on rationalizing the given equation we get

= $Lim_{x→1}\frac{(x - 1)}{\sqrt{(x^2 + 3)} - 2} \times \frac{\sqrt{(x^2 + 3)} + 2}{\sqrt{(x^2 + 3)} + 2}$

= Lim_x→1[(x – 1){√(x² + 3) + 2}]/{(x² + 3) – 4}

= Lim_x→1[(x – 1){√(x² + 3) + 2}]/(x² – 1)

= Lim_x→1[(x – 1){√(x² + 3) + 2}]/{(x – 1)(x + 1)}

= Lim_x→1{√(x² + 3) + 2}/{(x + 1)}

Now put x = 1, we get

= {√(1 + 3) + 2}/(1 + 1)

= (2 + 2)/2

= 2

问题10. Lim _x→3 {√(x + 3)–√6} /(x ² – 9)

解决方案：

We have, Lim_x→3{√(x + 3) – √6}/(x² – 9)

Find the limit of the given equation

When we put x = 3, this expression takes the form of 0/0.

So, on rationalizing the given equation we get

= $Lim_{x→3}\frac{\sqrt{(x + 3)} - \sqrt{6}}{(x^2 - 9)} \times \frac{\sqrt{(x + 3)} + \sqrt{6}}{\sqrt{(x + 3)} + \sqrt{6}}$

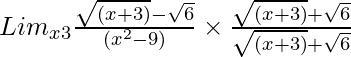
= Lim_x→3{(x + 3) – 6}/[(x² – 9){√( x+ 3) + √6}]

= Lim_x→3(x – 3)/[(x – 3)(x + 3){√(x + 3) + √6}]

= Lim_x→3(1)/[(x + 3){√(x + 3) + √6}]

Now put x = 3, we get

= $\frac{1}{(3+3)(\sqrt6+\sqrt6)}$

= $\frac{1}{(6)(2\sqrt6)}$

= 1/(12√6)

问题11. Lim _x→1 {√(5x – 4)–√x} /(x ² – 1)

解决方案：

We have, Lim_x→1{√(5x – 4) – √x}/(x² – 1)

Find the limit of the given equation

When we put x = 1, this expression takes the form of 0/0.

So, on rationalizing the given equation we get

= $Lim_{x→1}\frac{\sqrt{(5x - 4)} - \sqrt{x}}{(x^2 - 1)} \times \frac{\sqrt{(5x - 4)} + \sqrt{x}}{\sqrt{(5x - 4)} + \sqrt{x}}$

= Lim_x→1{5x – 4 – x}/[(x² – 1){√(5x – 4) + √x}]

= Lim_x→1{4(x – 1)}/[(x – 1)(x + 1){√(5x – 4) + √x}]

= Lim_x→1(4)/[{√(5x – 4) + √x}(x + 1)]

Now put x = 1, we get

= 4/[{√(5 – 4) + √1}(1 + 1)]

= 4/[(1 + 1)(1 + 1)]

= 4/4

= 1

问题12. Lim _x→0 {√(1 + x)– 1} / x

解决方案：

We have, Lim_x→0{√(1 + x) – 1}/x

Find the limit of the given equation

When we put x = 0, this expression takes the form of 0/0.

So, on rationalizing the given equation we get

= $Lim_{x→0}\frac{\sqrt{(1 + x)} - 1}{x} \times \frac{\sqrt{(1 + x)} + 1}{\sqrt{(1 + x)} + 1}$

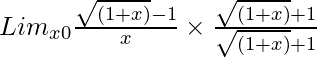
= Lim_x→0(1 + x – 1)/[x{√(1 + x) + 1}]

= Lim_x→0(x)/[x{√(1 + x) + 1}]

= Lim_x→0(1)/{√(1 + x) + 1}

Now put x = 0, we get

= 1/(1 + 1)

= 1/2

问题13. Lim _x→2 {√(x ² +1)–√5} /(x – 2)

解决方案：

We have, Lim_x→2{√(x² + 1) – √5}/(x – 2)

Find the limit of the given equation

When we put x = 2, this expression takes the form of 0/0.

So, on rationalizing the given equation we get

= $Lim_{x→2}\frac{\sqrt{(x^2 + 1)} - \sqrt{5}}{(x - 2)} \times \frac{\sqrt{(x^2 + 1)} + \sqrt{5}}{\sqrt{(x^2 + 1)} + \sqrt{5}}$

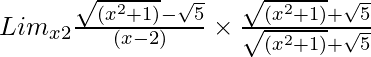
= Lim_x→2{x² + 1 – 5}/[(x – 2){√(x² + 1) + √5}]

= Lim_x→2{(x² – 4)}/[(x – 2){√(x² + 1) + √5}]

= Lim_x→2{(x – 2)(x + 2)}/[(x – 2){√(x² + 1) + √5}]

= Lim_x→2{(x + 2)}/{√(x² + 1) + √5}

Now put x = 2, we get

= 4/{√(5) + √5}

= 4/(2√5)

= 2/(√5)

问题14. Lim _x→2 (x – 2)/ {√x–√2}

解决方案：

We have, Lim_x→2(x – 2)/{√x – √2}

Find the limit of the given equation

When we put x = 2, this expression takes the form of 0/0.

So, on rationalizing the given equation we get

= $Lim_{x→2}\frac{(x - 2)}{√x - √2} \times \frac{√x + √2}{√x + √2}$

= Lim_x→2[(x – 2){√x + √2}]/{x – 2}

= Lim_x→2{√x + √2}

Now put x = 2, we get

= √2 + √2

= 2√2

问题15. Lim _x→7 {4 –√(9 + x)} / {1 –√(8 – x)}

解决方案：

We have, Lim_x→7{4 – √(9 + x)}/{1 – √(8 – x)}

Find the limit of the given equation

When we put x = 7, this expression takes the form of 0/0.

So, on rationalizing the given equation we get

=$\lim_{x\to7}\frac{(4-\sqrt{9+x})(4+\sqrt{9+x})(1+\sqrt{8-x})}{(1-\sqrt{8-x})(4+\sqrt{9+x})(1+\sqrt{8-x})}$

=$\lim_{x\to7}\frac{16-(9+x)}{1-(8-x)}×\frac{(1+\sqrt{8-x})}{(4+\sqrt{9+x})}$

=$\lim_{x\to7}\frac{(7-x)}{-1(7-x)}×\frac{(1+\sqrt{8-x})}{(4+\sqrt{9+x})}$

Now put x = 7, we get

= -{1 + √(8 – 7)}/{4 + √(9 + 7)}

= -2/(4 + 4)

= -1/4

问题16. Lim _{x→0 {} √(a + x)–√a} / [x {√(a ² + ax)}]

解决方案：

We have,

Lim_x→0{√(a + x) – √a}/[x{√(a² + ax)}]

Find the limit of the given equation

When we put x = 0, this expression takes the form of 0/0.

So, on rationalizing the given equation we get

= $Lim_{x→0}\frac{\sqrt{(a + x)} - \sqrt{a}}{x\sqrt{(a^2 + ax)}} \times \frac{\sqrt{(a + x)} + \sqrt{a}}{\sqrt{(a + x)} + \sqrt{a}}$

= Lim_x→0{(a + x) – a}/[x{√(a² + ax)}{√(a + x) + √a}]

= Lim_x→0(x)/[x{√(a² + ax)}{√(a + x) + √a}]

= Lim_x→0(1)/[{√(a² + ax)}{√(a + x) + √a}]

Now put x = 0, we get

= 1/{a.(√a + √a)}

= 1/(2a√a)

问题17. Lim _x→7 (x – 5)/ {√(6x – 5)–√(4x + 5)}

解决方案：

We have, Lim_x→7(x – 5)/{√(6x – 5) – √(4x + 5)}

Find the limit of the given equation

When we put x = 7, this expression takes the form of 0/0.

So, on rationalizing the given equation we get

= $Lim_{x→7}\frac{(x - 5)}{\sqrt{(6x - 5)} - \sqrt{(4x + 5)}} \times \frac{\sqrt{(6x - 5)} + \sqrt{(4x + 5)}}{\sqrt{(6x - 5)} + \sqrt{(4x + 5)}}$

= Lim_x→7[{√(6x – 5) + √(4x + 5)}(x – 5)]/{(6x-5) – (4x + 5)}

= Lim_x→7[{√(6x – 5) + √(4x + 5)}(x – 5)]/{2(x – 5)}

= Lim_x→7[{√(6x – 5) + √(4x + 5)}]/(2)

Now put x = 7, we get

= {√(6 × 7 – 5) + √(4 × 7 + 5)}/(2)

= (5 + 5)/2

= 5