第11类RD Sharma解决方案–第29章限制–练习29.9

问题1 $\lim_{x \to \pi}\frac{1+\cos x}{\tan ^2x}$

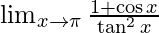
解决方案：

Given,$\lim_{x \to \pi}\frac{1+\cos x}{\tan ^2x}$

By Applying limits, we get,

⇒$\frac{1+\cos \pi}{\tan ^2\pi}$ = $\frac{0}{0}$   (Indeterminate form or 0/0 form)

So, we cannot just directly apply the limits as we got indeterminate form.

On substituting $\tan ^2x=\frac{sin^ 2x}{cos ^2x}$ we get,

⇒ $\lim_{x \to \pi}\frac{(1+\cos x)(\cos ^2x)}{\sin ^2x}$

We know, sin²x + cos²x = 1 

⇒ sin²x = 1 – cos²x  

⇒ $\lim_{x \to \pi}\frac{(1+\cos x)(\cos ^2x)}{1-\cos ^2x}$

By using a² − b² = (a + b)(a − b) we get,

⇒ $\lim_{x \to \pi}\frac{(1+\cos x)(\cos ^2x)}{(1+\cos x)(1-\cos x)}$

⇒ $\lim_{x \to \pi}\frac{\cos ^2x}{1-\cos x}$

Applying limits we get,

⇒ $\frac{\cos ^2\pi}{1-\cos \pi}$

⇒ $\frac{(-1)^2}{1-(-1)}=\frac{1}{2}$

Therefore, the value of $\lim_{x \to \pi}\frac{1+\cos x}{\tan ^2x} =\frac{1}{2}$

问题2。 $\lim_{x \to \frac{\pi}{4}}\frac{\cosec ^2x-2}{\cot x-1}$

解决方案：

Given,$\lim_{x \to \frac{\pi}{4}}\frac{\cosec ^2x-2}{\cot x-1}$

Applying the limits, we get,

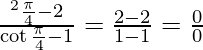⇒ $\frac{\cosec ^2\frac{\pi}{4}-2}{\cot \frac{\pi}{4}-1}=\frac{2-2}{1-1}=\frac{0}{0}$ (Indeterminate form)

So, we cannot just directly apply the limits as we got indeterminate form.

We know, cosec²x − cot²x = 1  

⇒ cosec²x = 1 + cot²x  

⇒ $\lim_{x \to \frac{\pi}{4}}\frac{1+\cot ^2x-2}{\cot x-1}$

⇒ $\lim_{x \to \frac{\pi}{4}}\frac{\cot ^2x-1}{\cot x-1}$

By using formula, a² − b² = (a + b)(a − b) we get,

⇒ $\lim_{x \to \frac{\pi}{4}}\frac{(\cot x-1)(\cot x+1)}{\cot x-1}$

⇒ $\lim_{x \to \frac{\pi}{4}}\cot x+1$

Applying the limits, we get, 

⇒ $\cot \frac{\pi}{4}+1 =2$

Therefore, the value of $\lim_{x \to \frac{\pi}{4}}\frac{\cosec ^2x-2}{\cot x-1} =2$

问题3。 $\lim_{x \to \frac{\pi}{6}}\frac{\cot ^2x-3}{\cosec x-2}$

解决方案：

Given,$\lim_{x \to \frac{\pi}{6}}\frac{\cot ^2x-3}{\cosec x-2}$

Applying the limits, we get,

⇒ $\frac{\cot ^2\frac{\pi}{6}-3}{\cosec \frac{\pi}{6}-2}=\frac{3-3}{2-2}=\frac{0}{0}$ (Indeterminate form)

We know, cosec²x − cot²x = 1 ⇒ cot²x = cosec²x – 1  

⇒ $\lim_{x \to \frac{\pi}{6}}\frac{\cosec ^2x-1-3}{\cosec x-2}$

⇒ $\lim_{x \to \frac{\pi}{6}}\frac{\cosec ^2x-4}{\cosec x-2}$

By using formula, a² − b² = (a + b)(a − b) we get,

 ⇒ $\lim_{x \to \frac{\pi}{6}}\frac{(\cosec x-2)(\cosec x+2)}{\cosec x-2}$

⇒ $\lim_{x \to \frac{\pi}{6}} (\cosec x+2)$

Applying the limits, we get,

⇒ $\cosec \frac{\pi}{6}+2=4$

Therefore, the value of $\lim_{x \to \frac{\pi}{6}}\frac{\cot ^2x-3}{\cosec x-2} =4$

问题4。 $\lim_{x \to \frac{\pi}{4}}\frac{2-\cosec ^2x}{1-\cot x}$

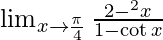
解决方案：

Given,$\lim_{x \to \frac{\pi}{4}}\frac{2-\cosec ^2x}{1-\cot x}$

Applying the limits we get,

⇒ $\frac{2-\cosec ^2\frac{\pi}{4}}{1-\cot \frac{\pi}{4}}=\frac{2-2}{1-1}=\frac{0}{0}$  (Indeterminate form)

So, we cannot just apply the limits.

We know, cosec²x − cot²x = 1 ⇒ cosec²x = 1 – cot²x    

⇒$\lim_{x \to \frac{\pi}{4}}\frac{2-(1+\cot ^2x)}{1-\cot x}$

⇒ $\lim_{x \to \frac{\pi}{4}}\frac{1-\cot ^2x}{1-\cot x}$

By using formula, a² − b² = (a + b)(a − b) we get,

⇒$\lim_{x \to \frac{\pi}{4}}\frac{(1-\cot x)(1+\cot x)}{1-\cot x}$

⇒ $\lim_{x \to \frac{\pi}{4}} (1+\cot x)$

Applying the limits we get,

⇒ $(1+\cot \frac{\pi}{4})=2$

Therefore, The value of $\lim_{x \to \frac{\pi}{4}}\frac{2-\cosec ^2x}{1-\cot x} =2$

问题5 $\lim_{x \to \pi}\frac{\sqrt{2+\cos x}-1}{(\pi -x)^2}$

解决方案：

Given,$\lim_{x \to \pi}\frac{\sqrt{2+\cos x}-1}{(\pi -x)^2}$

Applying the limits, we get,

⇒ $\frac{\sqrt{2+\cos \pi}-1}{(\pi -\pi)^2}=\frac{0}{0}$(Indeterminate form)

So, we cannot just apply the limits.

Rationalizing the numerator(multiplying and dividing with $\sqrt{2+\cos x}+1$)

⇒ $\lim_{x \to \pi}\frac{(\sqrt{2+\cos x}-1)(\sqrt{2+\cos x}+1)}{(\pi -x)^2(\sqrt{2+\cos x}+1)}$

⇒ $\lim_{x \to \pi}\frac{(2+\cos x-1)}{(\pi -x)^2(\sqrt{2+\cos x}+1)}$

Let x = π − h

If x → π, h → 0  

Substituting x = π − h we get,

⇒$\lim_{h \to 0}\frac{(1+\cos (\pi-h))}{(\pi -(\pi-h))^2(\sqrt{2+\cos (\pi-h)}+1)}$

We know that cos(π − x) = −cosx substituting we get, 

⇒ $\lim_{h \to 0}\frac{(1-\cos h)}{h^2(\sqrt{2-\cos h}+1)}$

By using cos2x = 1 − 2sin²x ⇒ cos h = 1 − 2sin²(h​/2)  

⇒$\lim_{h \to 0}\frac{2\sin^2(\frac{h}{2})}{(4\times\frac{h^2}{4})(\sqrt{2-\cos h}+1)}$

⇒ $\frac{1}{2} \lim_{h \to 0}[(\frac{\sin (\frac{h}{2})}{(\frac{h}{2})})^2\times(\frac{1}{\sqrt{2-\cos h}+1)})]$

We know that, $\lim_{x \to 0}\frac{\sin x}{x}=1$

Applying the limits, we get, 

⇒ $\frac{1}{2}\times1\times\frac{1}{\sqrt{2-1}+1}$

⇒ 1/2 x 1/2 = 1/4 

Therefore, the value of $\lim_{x \to \pi}\frac{\sqrt{2+\cos x}-1}{(\pi -x)^2} =\frac{1}{4}$

问题6。 $\lim_{x \to \frac{3\pi}{2}}\frac{1+\cosec ^3x}{\cot^ 2x}$

解决方案：

Given,$\lim_{x \to \frac{3\pi}{2}}\frac{1+\cosec ^3x}{\cot^ 2x}$

Applying the limits, we get,

⇒ $\frac{1+\cosec ^3(\frac{3\pi}{2})}{\cot^ 2(\frac{3\pi}{2})}=\frac{0}{0}$(Indeterminate form)

So, we cannot just directly apply the limits,

By using the formula, a³ + b³ = (a + b)(a² − ab + b²) we get,

⇒$\lim_{x \to \frac{3\pi}{2}}\frac{(1+\cosec x)(1-\cosec x+\cosec^ 2x)}{\cosec^ 2x-1}$

By using formula, a² − b² = (a + b)(a − b) 

⇒ $\lim_{x \to \frac{3\pi}{2}}\frac{(1+\cosec x)(1-\cosec x+\cosec^ 2x)}{(\cosec x-1)(\cosec x+1)}$

⇒ $\lim_{x \to \frac{3\pi}{2}}\frac{(1-\cosec x+\cosec^ 2x)}{\cosec x-1}$

Applying the limits, we get,

⇒ $\frac{(1-\cosec (\frac{3\pi}{2})+\cosec^ 2(\frac{3\pi}{2}))}{\cosec (\frac{3\pi}{2})-1} =\frac{3}{-2}$

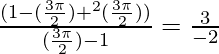
Therefore, the value of $\lim_{x \to \frac{3\pi}{2}}\frac{1+\cosec ^3x}{\cot^ 2x} =\frac{-3}{2}$