第11类RD Sharma解决方案–第29章限制–练习29.1

问题1.证明Lim _x→0 (x / | x |)不存在。

解决方案：

We have, Lim_x→0(x/|x|)

Now first we find left-hand limit:

= $\lim_{x\to0^-}\frac{x}{|x|}$

Let x = 0 – h, where h = 0

= $\lim_{h\to0^-}(\frac{(0 - h)}{|0 - h|})$

= $\lim_{h\to0^-}(\frac{(- h)}{h})$ 

= -1

Now we find right-hand limit:

= $\lim_{x\to0^+}\frac{x}{|x|}$

So, let x = 0 + h, where h = 0

= $\lim_{h\to0^+}(\frac{(0 + h)}{|0 + h|})$

= $\lim_{h\to0^+}(\frac{h}{h})$

= 1

Left-hand limit ≠ Right-hand limit

So, Lim_x→0(x/|x|) does not exist.

问题2.找到k，使Lim _x→0 f(x)，其中$f(x)= \begin{cases} 2x+3, \hspace{0.2cm}x\le2\\ x+k,\hspace{0.2cm}x>2 \end{cases}$

解决方案：

We have, 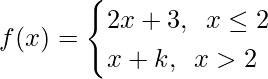$f(x)= \begin{cases} 2x+3, \hspace{0.2cm}x\le2\\ x+k,\hspace{0.2cm}x>2 \end{cases}$

Now first we find left-hand limit:

= $\lim_{x\to0^-}(2x+3)$

Let x = 2 – h, where h= 0.

=$\lim_{h\to0^-}(2(2-h)+3)$

= [2(2 – 0) + 3]

= 7

Now we find right-hand limit:

$=\lim_{x\to0^+}f(x)$

= $\lim_{x\to0^+}(x+k)$

Let x = 2 + h, where h = 0

= $\lim_{h\to0^+}((2+h)+k)$

= (2 + 0) + k

= (2 + k)

Here, Left-hand limit = Right-hand limit, so limit exists

So, (2 + k) = 7

k = 5

问题3.证明Lim _x→0 (1 / x)不存在。

解决方案：

We have to show that Lim_x→0(1/x) does not exists 

So for that 

First we find left-hand limit:

= $\lim_{x\to0^-}\frac{1}{x}$

Let x = 0 – h, where h = 0.

= $\lim_{h\to0^-}(\frac{1}{(0-h)})$

= $-\lim_{h\to0^-}(\frac{1}{h})$

= -∞

Now we find right-hand limit:

=$\lim_{x\to0^+}\frac{1}{x}$

Let x = 0 + h, where h = 0.

= $\lim_{h\to0^+}(\frac{1}{|(0+h)|})$

= $\lim_{h\to0^+}(\frac{1}{h})$

= ∞

Here, Left-hand limit ≠ Right-hand limit, so, Lim_x→0(1/x) does not exist.

问题4.令f(x)是一个由以下函数定义的函数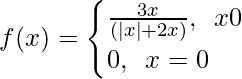$f(x)= \begin{cases} \frac{3x}{(|x|+2x)}, \hspace{0.2cm}x ≠ 0\\ 0,\hspace{0.2cm}x=0 \end{cases}$ 。证明lim _x→0 f(x)不存在。

解决方案：

We have, 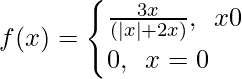$f(x)= \begin{cases} \frac{3x}{(|x|+2x)}, \hspace{0.2cm}x ≠ 0\\ 0,\hspace{0.2cm}x=0 \end{cases}$

According to the question we have to show that lim_x→0 f(x) does not exist.

So for that 

First we find left-hand limit:

=$\lim_{x\to0^-}\frac{3x}{|x|+2x}$

Let x = 0 – h, where h = 0

=$\lim_{h\to0^-}\frac{3(0-h)}{|0-h|+2(0-h)}$

=$\lim_{h\to0^-}(\frac{-3h}{h - 2h})$

=$\lim_{h\to0^-}(\frac{-3}{-1})$

= 3

Now we find right-hand limit:

=$\lim_{x\to0^+}\frac{3x}{|x|+2x}$

Let x = 0 + h, where h = 0.

=$\lim_{h\to0^+}\frac{3(0+h)}{|0+h|+2(0+h)}$

=$\lim_{h\to0^+}(\frac{3h}{3h})$

=$\lim_{h\to0^+}(\frac{3}{3})$

= 1

Here, Left-hand limit ≠ Right-hand limit, so, lim_x→0 f(x) does not exist.

问题5 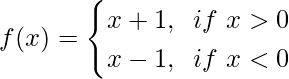$f(x)= \begin{cases} x+1, \hspace{0.2cm}if \ x >0\\ x-1,\hspace{0.2cm}if \ x<0 \end{cases}$ ，证明lim _x→0 f(x)不存在。

解决方案：

We have, 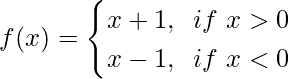$f(x)= \begin{cases} x+1, \hspace{0.2cm}if \ x >0\\ x-1,\hspace{0.2cm}if \ x<0 \end{cases}$

And we have to prove that lim_x→0f(x) does not exist.

So for that 

First we find left-hand limit:

=$\lim_{x\to0^-}(x-1)$

Let x = 0 – h, where h = 0.

=$\lim_{h\to0^-}((0-h)-1)$

=$\lim_{h\to0^-}(0-h-1)$

= -1

Now we find right-hand limit:

=$\lim_{x\to0^+}(x+1)$

Let x = 0 + h, where h = 0.

=$\lim_{h\to0^+}((0+h)+1)$

=$\lim_{h\to0^+}(0+h+1)$

= 1

Here, Left-hand limit ≠ Right-hand limit, so, lim_x→0f(x) does not exist.

问题6 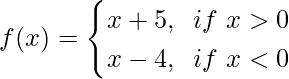$f(x)= \begin{cases} x+5, \hspace{0.2cm}if \ x >0\\ x-4,\hspace{0.2cm}if \ x<0 \end{cases}$ ，证明lim _x→0 f(x)不存在。

解决方案：

We have,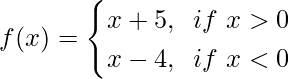$f(x)= \begin{cases} x+5, \hspace{0.2cm}if \ x >0\\ x-4,\hspace{0.2cm}if \ x<0 \end{cases}$

And we have to prove that lim_x→0f(x) does not exist.

So for that 

First we find left-hand limit:

=$\lim_{x\to0^-}(x-4)$

Let x = 0 – h, where h = 0.

=$\lim_{h\to0^-}((0-h)-4)$

=$\lim_{h\to0^-}(0-h-4)$

= -4

Now we find right-hand limit:

=$\lim_{x\to0^+}(x+5)$

Let x = 0 + h, where h = 0.

=$\lim_{h\to0^+}((0+h)+5)$

=$\lim_{h\to0^+}(0+h+5)$

= 5

Here, Left-hand limit ≠ Right-hand limit, so, lim_x→0f(x) does not exist.

问题7：找到lim _x→3 f(x)，其中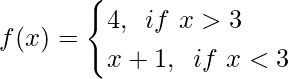$f(x)= \begin{cases} 4, \hspace{0.2cm}if \ x >3\\ x+1,\hspace{0.2cm}if \ x<3 \end{cases}$

解决方案：

We have, 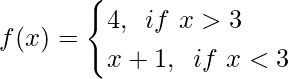$f(x)= \begin{cases} 4, \hspace{0.2cm}if \ x >3\\ x+1,\hspace{0.2cm}if \ x<3 \end{cases}$

And we have to find lim_x→3f(x)

So for that 

First we find left-hand limit:

=$\lim_{x\to3^-}(x+1)$

Let x = 3 – h, where h = 0.

= $\lim_{h\to0^-}((3-h)+1)$ 

= $\lim_{h\to0^-}(4-h)$ 

= 4

Now we find right-hand limit:

=$\lim_{x\to3^+}4$

Let x = 3 + h, where h = 0.

=$\lim_{h\to0^+}4$

= 4

Here, Left-hand limit = Right-hand limit, 

Hence, lim_x→3f(x) = 4

问题8(i)。如果$f(x)= \begin{cases} 2x+3, \hspace{0.2cm}if \ x\le0\\ 3(x+1),\hspace{0.2cm}if \ x>0 \end{cases}$ ，求出lim _x→0 f(x)。

解决方案：

We have, 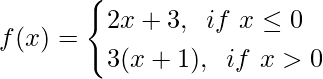$f(x)= \begin{cases} 2x+3, \hspace{0.2cm}if \ x\le0\\ 3(x+1),\hspace{0.2cm}if \ x>0 \end{cases}$

And we have to find lim_x→0f(x)

So for that 

First we find left-hand limit:

=$\lim_{x\to0^-}(2x+3)$

Let x = 0 – h, where h = 0.

= $\lim_{h\to0^-}(2(0-h)+3)$

= $\lim_{h\to0^-}(3-2h)$

= 3

Now we find right-hand limit:

=$\lim_{x\to0^+}3(x+1)$

Let x = 0 + h, where h = 0.

=$\lim_{h\to0^+}3((0+h)+1)$

=$\lim_{h\to0^+}(3+3h)$

= 3

Here, Left-hand limit = Right-hand limit, 

Hence, lim_x→0f(x) = 3

问题8(ii)。如果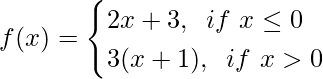$f(x)= \begin{cases} 2x+3, \hspace{0.2cm}if \ x\le0\\ 3(x+1),\hspace{0.2cm}if \ x>0 \end{cases}$ ，求出lim _x→1 f(x)。

解决方案：

We have, 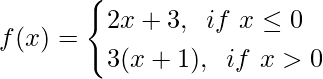$f(x)= \begin{cases} 2x+3, \hspace{0.2cm}if \ x\le0\\ 3(x+1),\hspace{0.2cm}if \ x>0 \end{cases}$

And we have to find lim_x→1f(x)

So for that 

First we find left-hand limit:

=$\lim_{x\to1^-}(2x+3)$

Let x = 1 – h, where h = 0.

= $\lim_{h\to0^-}(2(1-h)+3)$

= $\lim_{h\to0^-}(5-2h)$

= 5

Now we find right-hand limit:

=$\lim_{x\to1^+}3(x+1)$

Let x = 1 + h, where h = 0.

=$\lim_{h\to0^+}(3((1+h)+1))$

= $\lim_{h\to0^+}(6+3h)$

= 6

Here, Left-hand limit ≠ Right-hand limit, so lim_x→1f(x) does not exist.

问题9.求出lim _x→1 f(x)其中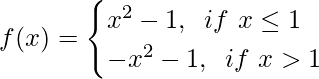$f(x)= \begin{cases} x^2-1, \hspace{0.2cm}if \ x\le1\\ -x^2-1,\hspace{0.2cm}if \ x>1 \end{cases}$

解决方案：

We have, 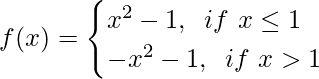$f(x)= \begin{cases} x^2-1, \hspace{0.2cm}if \ x\le1\\ -x^2-1,\hspace{0.2cm}if \ x>1 \end{cases}$

And we have to find lim_x→1f(x)

So for that 

First we find left-hand limit:

=$\lim_{x\to1^-}(x^2-1)$

Let x = 1 – h, where h = 0.

= $\lim_{h\to0^-}((1-h)^2-1)$

=$\lim_{h\to0^-}(1-2h-h^2-1)$

= 0

Now we find right-hand limit:

=$\lim_{x\to1^+}(-x^2-1)$

Let x = 1 + h, where h = 0.

= $\lim_{h\to0^+}(-(1+h)^2-1)$

= $\lim_{h\to0^+}(-1-2h-h^2-1)$

= -2

Here, Left-hand limit ≠ Right-hand limit, so, lim_x→1f(x) does not exist.

问题10.计算lim _x→0 f(x)，其中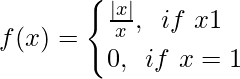$f(x)= \begin{cases} \frac{|x|}{x}, \hspace{0.2cm}if \ x≠1\\ 0,\hspace{0.2cm}if \ x=1 \end{cases}$

解决方案：

We have, 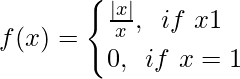$f(x)= \begin{cases} \frac{|x|}{x}, \hspace{0.2cm}if \ x≠1\\ 0,\hspace{0.2cm}if \ x=1 \end{cases}$

And we have to find lim_x→0f(x)

So for that 

First we find left-hand limit:

=$\lim_{x\to0^-}\frac{|x|}{x}$

Let x = 0 – h, where h = 0.

= $\lim_{h\to0^-}\frac{|0-h|}{0-h}$

= $\lim_{h\to0^-}(\frac{h}{-h})$

= -1

Now we find right-hand limit:

=$\lim_{x\to0^+}\frac{|x|}{x}$

Let x = 0 + h, where h = 0.

= $\lim_{h\to0^+}\frac{|0+h|}{0+h}$

= $\lim_{h\to0^+}(\frac{h}{h})$

= 1

Here, Left-hand limit ≠ Right-hand limit, so, lim_x→0f(x) does not exist.

问题11.设a ₁ ，a ₂ ，…..a _n为固定实数，使得f(x)=(x – a ₁ )(x – a ₂ )……..(xa _n )。 lim _x→a1 f(x)是什么?计算lim _x→a f(x)。

解决方案：

We have, f(x) = (x – a₁)(x – a₂)……..(x – a_n)

$\lim_{x→a_1}f(x)=\lim_{x→a_1}[(x-a_1)(x-a_2)........(x-a_n)]$

Now, put x = a₁

= (a₁ – a₁)(a₁ – a₂)……..(a₁ – a_n)

= 0

Now, lim_x→af(x) = lim_x→a[(x – a₁)(x – a₂)……..(x – a_n)]

Now, put x = a

= (a – a₁)(a – a₂)……..(a – a_n)

Hence, lim_x→af(x) = (a – a₁)(a – a₂)……..(a – a_n)

问题12。求出lim _{x→1 ⁺} [1 /(x – 1)]。

解决方案：

We have to find lim_x→1⁺[1/(x – 1)]

=$\lim_{x\to1^+}\frac{1}{x-1}$

Let x = 1 + h, where h = 0.

=$\lim_{h\to0^+}\frac{1}{(1+h)-1}$

=$\lim_{h\to0^+}\frac{1}{(h)}$

= ∞

Hence, lim_x→1⁺[1/(x – 1)] = ∞

问题13(i)。评估以下单边限制：lim _{x→2 ⁺} [(x – 3)/(x ² – 4)]

解决方案：

We have, $\lim_{x\to2^+}\frac{x-3}{x^2-4}$

Let x = 2 + h, where h = 0.

=$\lim_{h\to0^+}(\frac{(2+h)-3}{(2+h)^2-4})$ 

= $\lim_{h\to0^+}(\frac{h-1}{4+4h+h^2-4})$

= $\lim_{h\to0^+}(\frac{h-1}{4h+h^2})$

= -∞

问题13(ii)。评估以下单边限制：lim _{x→2 ^–} [(x – 3)/(x ² – 4)]

解决方案：

We have,$\lim_{x\to2^-}\frac{x-3}{x^2-4}$

Let x = 2 – h, where h = 0.

= $\lim_{h\to0^-}\frac{(2-h)-3}{(2-h)^2-4}$

= $\lim_{h\to0^-}\frac{(-h-1)}{4-4h+h^2-4}$

= $\lim_{h\to0^-}\frac{(-h-1)}{-4h+h^2}$

= ∞

问题13(iii)。评估以下单边限制：lim _{x→0 ⁺} [1 / 3x]

解决方案：

We have, lim_x→0⁺[1/3x]

Let x = 0 + h, where h = 0.

= Lim_h→0⁺[1/3(0+h)]

= Lim_h→0⁺[1/(3h)]

= ∞

问题13(iv)。评估以下单边限制：lim _{x→-8 ⁺} [2x /(x + 8)]

解决方案：

We have, lim_x→-8⁺[2x/(x + 8)]

Let x = -8 + h, where h = 0.

= lim_x→0⁺[2(-8 + h)/(-8 + h + 8)]

= Lim_h→0⁺[(2h – 16)/(h)]

= -∞

问题13(v)。评估以下单边限制：lim _{x→0 ⁺} [2 / x ^1/5 ]

解决方案：

We have, lim_x→0⁺[2/x^1/5]

Let x = 0 + h, where h = 0.

= Lim_h→0⁺[2/(0 + h)^1/5]

= ∞

问题13(vi)。评估以下单边限制：lim _{x→(π/ 2) ^–} [tanx]

解决方案：

We have, lim_{x→(π/2)^–}[tanx]

Let x = 0 – h, where h = 0.

= lim_h→0^–[tan(π/2 – h)]

= lim_x→0^–[cot h]

= ∞

问题13(vii)。评估以下单边限制：lim _{x→(-π/ 2) ⁺} [secx]

解决方案：

We have, lim_{x→(-π/2)⁺}[secx]

Let x = 0 + h, where h = 0.

= lim_h→0⁺[secx(-π/2 + h)]

= lim_h→0⁺[cosec h]

= ∞

问题13(viii)。评估以下单边限制：lim _{x→0 ^–} [(x ² – 3x + 2)/ x ³ – 2x ² ]

解决方案：

We have, lim_x→0-[x² – 3x + 2/x³ – 2x²]

= Lim_x→0-[(x – 1)(x – 2)/x²(x – 2)]

= Lim_x→0-[(x – 1)/x²]

Let x = 0 – h, where h = 0.

= Lim_h→0-[(0 – h – 1)/(0 – h)²]

= -∞

问题13(ix)。评估以下单边限制：lim _{x→-2 ⁺} [(x ² – 1)/(2x + 4)]

解决方案：

We have, lim_x→-2⁺[(x² – 1)/(2x + 4)]

Let x = -2 + h, where h = 0.

= Lim_h→-0⁺[(-2 + h)² – 1)/2(-2 + h) + 4]

= Lim_h→-0⁺[(-2 + h)² – 1)/(-4 + 4 + h)]

= (4 – 1)/0

= ∞

问题13(x)。评估以下单边限制：lim _x→0- [2 – cotx]

解决方案：

We have, lim_x→0-[2 – cotx]

Let x = 0 – h, where h = 0.

= Lim_h→0-[2 – cot(0 – h)]

= Lim_h→0-[2 + cot(h)]

= 2 + ∞

= ∞

问题13(xi)。评估以下单方面的限制。 lim _x→0- [1 + cosecx]

解决方案：

We have, lim_x→0-[1 + cosecx]

Let x = 0 – h, where h = 0.

= Lim_h→0-[1 + cosec(0 – h)]

= Lim_h→0-[1 – cosec(h)]

= 1 – ∞

= -∞

问题14.证明Lim _x→0 e ^{-1 / x}不存在。

解决方案：

Let, f(x) = Lim_x→0e^-1/x

So for that 

First we find left-hand limit:

= $\lim_{x\to0^-}e^{-\frac{1}{x}}$

Let x = 0 – h, where h = 0.

= $\lim_{h\to0^-}e^{-\frac{1}{0-h}}$

=$\lim_{h\to0^-}e^{\frac{1}{h}}$

= e^∞

= ∞

Now we find right-hand limit:

=$\lim_{x\to0^+}e^{-\frac{1}{x}}$

Let x = 0 + h, where h = 0.

= $\lim_{h\to0^+}e^{-\frac{1}{0 + h}}$

=$\lim_{h\to0^+}e^{-\frac{1}{h}}$

= e^-∞

= 0

Here, Left-hand limit ≠ Right-hand limit, so, Lim_x→0e^-1/x does not exist.

问题15(i)。寻找林_x→2 [x]

解决方案：

We have, Lim_x→2[x], where [] is Greatest Integer Function

So for that 

First we find left-hand limit:

=$\lim_{x\to0^-}[x]$

Let x = 2 – h, where h = 0.

= $\lim_{h\to0^-}[2 - h]$

= 1

Now we find right-hand limit:

= $\lim_{x\to0^+}[x]$

Let x = 2 + h, where h = 0.

= $\lim_{h\to0^+}[2+h]$

= 2

Here, Left-hand limit ≠ Right-hand limit, so, Lim_x→2[x] does not exist.

问题15(ii)。寻找林_x→5/2 [x]

解决方案：

We have, Lim_x→2[x], where [] is Greatest Integer Function

So for that 

First we find left-hand limit:

=$\lim_{x\to\frac{5}{2}^-}[x]$

Let x = 5/2 – h, where h = 0.

= $\lim_{h\to0^-}[\frac{5}{2} - h]$

= 2

Now we find right-hand limit:

=$\lim_{x\to\frac{5}{2}^+}[x]$

Let x = 5/2 + h, where h = 0.

= $\lim_{h\to0^+}[\frac{5}{2}+h]$

= 2

Here, Left-hand limit = Right-hand limit, so, Lim_x→5/2[x] = 2

问题15(iii)。寻找林_x→1 [x]

解决方案：

We have, Lim_x→1[x], where [] is Greatest Integer Function

So for that 

First we find left-hand limit:

=$\lim_{x\to1^-}[x]$

Let x = 1 – h, where h = 0.

= $\lim_{h\to0^-}[1-h]$

= 0

Now we find right-hand limit:

= $\lim_{x\to1^+}[x]$

Let x = 1 + h, where h = 0.

= $\lim_{h\to0^+}[1+h]$ 

= 1

Here, Left-hand limit = Right-hand limit, so, Lim_x→1[x] does not exist.

问题16：证明Lim _{x→a +} [x] = [a]。还证明Lim _x→1- [x] = 0。

解决方案：

We have, $\lim_{x\to a^+}[x]$

Let x = a + h, where h = 0.

= Lim_h→0-[(a + h)]

= a

Also, $\lim_{x\to1^-}[x]$

Let x = 1 – h, where h = 0.

= Lim_h→0[(1 – h)]

= 0

问题17。证明Lim _x→2 +(x / [x])≠Lim _x→2- (x / [x])。

解决方案：

We have to show Lim_x→2+(x/[x]) ≠ Lim_x→2-(x/[x])

So, R.H.L

We have, $\lim_{x\to2^-}\frac{x}{[x]}$, where [] is greatest Integer Function

Let x = 2 – h, where h = 0.

= Lim_h→0-[(2 – h)/|[2 – h]]

= 2/1

= 2

Now, L.H.L 

We have, $\lim_{x\to2^+}\frac{x}{[x]}$, where [] is greatest Integer Function

Let x = 2 + h, where h = 0.

= Lim_h→0+[(2 + h)/|[2 + h]]

= 2/2

= 1

Hence, Left-hand limit≠Right-hand limit

问题18：找到Lim _x→3 +(x / [x])。它等于Lim _x→3- (x / [x])

解决方案：

We have, $\lim_{x\to3^-}\frac{x}{[x]}$ Where [] is Greatest Integer Function

Let x = 3 – h, where h = 0.

= Lim_h→0-[(3 – h)/|[3 – h]]

= 3/2

Also, $\lim_{x\to3^+}\frac{x}{[x]}$

Let x = 3 + h, where h = 0.

= Lim_h→0+[(3 + h)/|[3 + h]]

= 3/3

= 1

Hence, Left-hand limit≠Right-hand limit

问题19.查找林_x→5/2 [x]

解决方案：

We have to find Lim_x→5/2[x], where [] is Greatest Integer Function

So for that 

First we find left-hand limit:

= $\lim_{x\to\frac{5}{2}^-}[x]$

Let x = 5/2 – h, where h = 0.

= Lim_h→0-[(5/2 – h)]

= 2

Now we find right-hand limit:

$=\lim_{x\to\frac{5}{2}^+}[x]$

Let x = 5/2 + h, where h = 0.

= Lim_h→0+[(5/2+h)]

= 2

Hence, Left-hand limit = Right-hand limit, so Lim_x→5/2[x]  = 2

问题20.评估Lim _x→2 f(x)，其中$f(x)= \begin{cases} x-[x], \hspace{0.2cm}x<2\\ 4 , \hspace{0.2cm}x=2\\ x+k,\hspace{0.2cm}x>2 \end{cases}$

解决方案：

We have, $f(x)= \begin{cases} x-[x], \hspace{0.2cm}x<2\\ 4 , \hspace{0.2cm}x=2\\ x+k,\hspace{0.2cm}x>2 \end{cases}$

We have to find Lim_x→2f(x)

So for that 

First we find left-hand limit:

= $\lim_{x\to0^-}(x-[x])$

Let x = 2 – h, where h = 0.

= Lim_h→0-{(2 – h) – [2 – h]}

= 2 – 1

= 1

Now we find right-hand limit:

=$\lim_{x\to2^+}(3x-5)$

Let x = 2 + h, where h = 0.

= Lim_h→0-[3(2 + h) – 5]

= 6 – 5

= 1

Hence, Left-hand limit = Right-hand limit, so, Lim_x→2f(x) = 1

问题21：证明Lim _x→0 sin(1 / x)不存在。

解决方案：

Let, f(x) = Lim_x→0sin(1/x)

First we find left-hand limit:

= $\lim_{x\to0^-}sin\frac{1}{x}$

Let x = 0 – h, where h = 0.

= Lim_h→0sin[1/(0 – h)]

= -Lim_h→0sin[1/(h)]

An oscillating number lies between -1 to +1.

So left hand limit does not exists.

Similarly, right-hand limit is also oscillating.

So, Lim_x→0sin(1/x) does not exist.

问题22.让$f(x)= \begin{cases} \frac{kcosx}{\pi-2x}, \hspace{0.2cm}where \ x \neq \frac{\pi}{2}\\ 3,\hspace{0.2cm}where \ x =\frac{\pi}{2} \end{cases}$如果lim _{x→ π/ 2} f(x)= f(π/ 2)，则求出k的值。

解决方案：

We have $f(x)= \begin{cases} \frac{kcosx}{\pi-2x}, \hspace{0.2cm}where \ x \neq \frac{\pi}{2}\\ 3,\hspace{0.2cm}where \ x =\frac{\pi}{2} \end{cases}$

First we find left-hand limit:

= $\lim_{x\to\frac{\pi}{2}^-}\frac{kcosx}{\pi-2x}$

Let x = π/2 – h, where h = 0.

=$\lim_{h\to0^-}\frac{kcos(\frac{\pi}{2}-h)}{\pi-2(\frac{\pi}{2}-h)}$

= k cos(π/2 – π/2)/π

= k/π

Now we find right-hand limit:

$\lim_{x\to\frac{\pi}{2}^+}\frac{kcosx}{\pi-2x}$

Let x = π/2 + h, where h = 0.

=$\lim_{h\to0^+}\frac{kcos(\frac{\pi}{2}+h)}{\pi-2(\frac{\pi}{2}+h)}$

= k cos(π/2 + π/2)/-π

= k/π

Hence, Left-hand limit = Right-hand limit, so

lim _x→π​/2 f(x) = f(π/2)

k/π = 3 

k = 3π