11类NCERT解决方案-第12章三维几何简介–第12章的其他练习

问题1：平行四边形ABCD的三个顶点是A(3，– 1，2，2)，B(1,2，– 4)和C(– 1，1，2)。找到第四个顶点的坐标。

解决方案：

ABCD is a parallelogram, with vertices A (3, -1, 2), B (1, 2, -4), C (-1, 1, 2) and D (x, y, z).

Using the property:

The diagonals of a parallelogram bisect each other, 

Midpoint of AC = Midpoint of BD = Point O

Now, by using Midpoint section formula

Coordinates of O for the line segment joining (x₁,y₁,z₁) and (x₂,y₂,z₂) = $(\frac{x_1+x_2}{2}, \frac{y_1+y_2}{2}, \frac{z_1+z_2}{2})$

So, Coordinates of O for the line segment joining AC = $(\frac{3+(-1)}{2}, \frac{-1+1}{2}, \frac{2+2}{2})$

= $(\frac{2}{2}, 0, \frac{4}{2})$

= (1, 0, 2) ……………………….(1)

and, Coordinates of O for the line segment joining BD = $(\frac{1+x}{2}, \frac{2+y}{2}, \frac{-4+z}{2})$ ………….(2)

Using the Eq(1) and Eq(2), we get

$\frac{1+x}{2}$ = 1

x = 1

$\frac{2+y}{2}$ = 0

y = -2

$\frac{z-4}{2}$ = 2

z = 8

Hence, the coordinates of the fourth vertex is D (1, -2, 8).

问题2：找到具有顶点A(0，0，6)，B(0,4，0)和(6，0，0)的三角形的中值长度。

解决方案：

The vertices of the triangle are A (0, 0, 6), B (0, 4, 0) and C (6, 0, 0).

So, let the medians be AD, BE and CF corresponding to the vertices A, B and C respectively.

D, E and F are the midpoints of the sides BC, AC and AB respectively.

Coordinates of mid-point for the line segment joining (x₁,y₁,z₁) and (x₂,y₂,z₂) = $(\frac{x_1+x_2}{2}, \frac{y_1+y_2}{2}, \frac{z_1+z_2}{2})$

So, Coordinates of D for the line segment joining BC = $(\frac{0+6}{2}, \frac{4+0}{2}, \frac{0+0}{2})$

Coordinates of D = (3, 2, 0)

and, Coordinates of E for the line segment joining AC = $(\frac{6+0}{2}, \frac{0+0}{2}, \frac{0+6}{2})$

Coordinates of E = (3, 0, 3)

and, Coordinates of F for the line segment joining AB = $(\frac{0+0}{2}, \frac{0+4}{2}, \frac{6+0}{2})$

Coordinates of F = (0, 2, 3)

By using the distance formula for two points, P(x₁,y₁,z₁) and Q(x₂,y₂,z₂)

PQ = $\mathbf{\sqrt{(x_1-x_2)^2+(y_1-y_2)^2+(z_1-z_2)^2}}$

So, AD = $\sqrt{(0-3)^2+(0-2)^2+(6-0)^2}$

AD =$\sqrt{9+4+36} = 7$

and, BE = $\sqrt{(0-3)^2+(4-0)^2+(0-3)^2}$

BE = $\sqrt{9+16+9} = \sqrt{34}$

and, CF = $\sqrt{(6-0)^2+(0-2)^2+(0-3)^2}$

CF = $\sqrt{36+4+9}$ = 7

Hence, the lengths of the medians are 7, √34 and 7.

问题3：如果原点是三角形PQR的质心，其顶点为P(2a，2，6)，Q(– 4，3b，–10)和R(8，14，2c)，则找到a，b和c。

解决方案：

The vertices of the triangle are P (2a, 2, 6), Q (-4, 3b, -10) and R (8, 14, 2c).

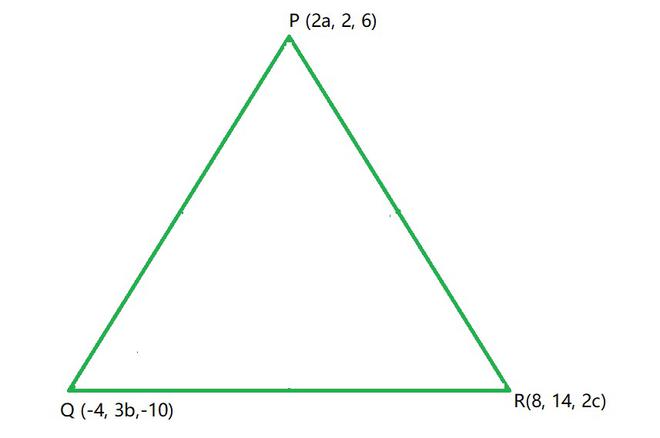
Coordinates of centroid(0, 0, 0) of the triangle having vertices (x₁,y₁,z₁), (x₂,y₂,z₂) and (x₃,y₃,z₃) = $(\frac{x_1+x_2+x_3}{3}, \frac{y_1+y_2+y_3}{3}, \frac{z_1+z_2+z_3}{3})$

(0, 0, 0) =$(\frac{2a-4+8}{3}, \frac{2+3b+14}{3}, \frac{6-10+2c}{3})$

(0, 0, 0) = $(\frac{2a+4}{3}, \frac{3b+16}{3}, \frac{2c-4}{3})$

So, $\frac{2a+4}{3}$ = 0

a = -2

and, $\frac{3b+16}{3}$ = 0

b = $\mathbf{\frac{-16}{3}}$

and, $\frac{2c-4}{3}$ = 0

c = 2

Hence, the values of a, b and c are a = -2, b = $\mathbf{\frac{-16}{3}}$ and c = 2

问题4：找到y轴上与点P(3，–2，5)相距5√2的点的坐标。

解决方案：

Point on y-axis = A (0, y, 0).

Distance between the points A (0, y, 0) and P (3, -2, 5) = 5√2.

Now, by using distance formula,

Distance of PQ = $\mathbf{\sqrt{(x_1-x_2)^2+(y_1-y_2)^2+(z_1-z_2)^2}}$

So, the distance between the points A (0, y, 0) and P (3, -2, 5) will be

Distance of AP = √[(3-0)² + (-2-y)² + (5-0)²]

= √[3² + (-2-y)² + 5²]

= √[(-2-y)² + 9 + 25]

5√2 = √[(-2-y)² + 34]

Squaring on both the sides, we get

(-2 -y)² + 34 = 25 × 2

(-2 -y)² = 50 – 34

4 + y² + (2 × -2 × -y) = 16

y² + 4y + 4 -16 = 0

y² + 4y – 12 = 0

y² + 6y – 2y – 12 = 0

y (y + 6) – 2 (y + 6) = 0

(y + 6) (y – 2) = 0

y = -6, y = 2

Hence, the points (0, 2, 0) and (0, -6, 0) are the required points on the y-axis.

问题5：具有x坐标4的点R位于连接点P(2，–3，4)和Q(8，0，10)的线段上。找到点R的坐标。

[提示：假设R将PQ除以k：1。点R的坐标由下式给出： $(\frac{8k+2}{k+1}, \frac{-3}{k+1}, \frac{10k+4}{k+1})$ ]

解决方案：

Let the coordinates of the required point be (4, y, z).

So now, let the point R (4, y, z) divides the line segment joining the points P (2, -3, 4) and Q (8, 0, 10) in the ratio k: 1.

Coordinates of the point which divides PQ in the ratio k : 1 = $(\frac{kx_2+x_1}{k+1}, \frac{ky_2+y_1}{k+1}, \frac{kz_2+z_1}{k+1})$

So, we have

$(\frac{8k+2}{k+1}, \frac{-3}{k+1}, \frac{10k+4}{k+1})$ = (4, y, z)

$\frac{8k+2}{k+1}$ = 4

8k + 2 = 4 (k + 1)

8k + 2 = 4k + 4

8k – 4k = 4 – 2

4k = 2

k = $\frac{2}{4}$

k = $\mathbf{\frac{1}{2}}$

Now, substituting the value we get,

y = $\frac{-3}{\frac{1}{2}+1} = \frac{-3}{\frac{3}{2}}$ = -2

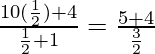z = $\frac{10(\frac{1}{2})+4}{\frac{1}{2}+1} = \frac{5+4}{\frac{3}{2}}$ = 6

Hence, the coordinates of the required point are (4, -2, 6).

问题6：如果A和B分别是点(3、4、5)和(–1、3，–7)，则找到点集合P的方程，使得PA ² + PB ² = k ² ，其中k是一个常数。

解决方案：

The points A (3, 4, 5) and B (-1, 3, -7)

Let the point be P (x, y, z).

Now by using distance formula,

Distance of point (x₁, y₁, z₁) and (x₂, y₂, z₂) = $\mathbf{\sqrt{(x_1-x_2)^2+(y_1-y_2)^2+(z_1-z_2)^2}}$

So, the distance between the points A (3, 4, 5) and P (x,y,z)) will be

Distance of PA = √[(3-x)² + (4-y)² + (5-z)²]

Distance of PB = √[(-1-x)² + (3-y)² + (-7-z)²]

As, PA² + PB² = k²

[(3 – x)² + (4 – y)² + (5 – z)²] + [(-1 – x)² + (3 – y)² + (-7 – z)²] = k²

[(9 + x² – 6x) + (16 + y² – 8y) + (25 + z² – 10z)] + [(1 + x² + 2x) + (9 + y² – 6y) + (49 + z² + 14z)] = k²

9 + x² – 6x + 16 + y² – 8y + 25 + z² – 10z + 1 + x² + 2x + 9 + y² – 6y + 49 + z² + 14z = k²

2x² + 2y² + 2z² – 4x – 14y + 4z + 109 = k²

2x² + 2y² + 2z² – 4x – 14y + 4z = k² – 109

2 (x² + y² + z² – 2x – 7y + 2z) = k² – 109

(x² + y² + z² – 2x – 7y + 2z) = $\frac{(k^2 - 109)}{2}$

Hence, the required equation is (x² + y² + z² – 2x – 7y + 2z) = $\mathbf{\frac{(k^2 - 109)}{2}}$