11类RD Sharma解决方案–第23章直线-练习23.6 |套装1

问题1.找到直线的等式：

(1)从轴上切除截距3和2

解决方案

Given that, a = 3, b = 2

Now we find the equation of line cutoff intercepts from the axes,

According to the formula of the equation of the line 

x/a + y/b = 1 

we get x/3 + y/2 = 1

By taking LCM,

2x + 3y = 6

Hence, the equation of line cut off intercepts 3 and 2 from the axes is 2x + 3y = 6

(2)从轴上切除截距-5和6

解决方案

Given that,

a = -5, b = 6

According to the formula of the equation of the line 

x/a + y/b = 1 

We get x/-5 + y/6 = 1     ….(1)

Take LCM of eq(1)

6x – 5y = -30

Hence, the equation of line cut off intercepts 3 and 2 from the axes is 6x – 5y = -30

问题2。找到通过(1，-2)并在轴上截去相等截距的直线方程。

解决方案 ：

In the question given that,

A line passing through (1, -2)

Let us considered that, the equation of the line cutting 

equal intercepts at coordinates of length ‘m’ is

According to the formula of the equation of the line 

x/a + y/b = 1 

We get

x/m + y/m = 1

x + y = m

The straight line x + y = m passes through (1, -2)

So, the given point satisfy the equation

1 – 2 = m

m = -1

Hence, the equation of the line is x+ y = -1

问题3.找到通过点(5，6)并在轴上有截距的直线上的方程

(1)数量级相等且均为正

解决方案：

We have given that,

a = b

Now find the equation of line cutoff intercepts from the axes:

According to the formula of the equation of the line 

x/a + y/b = 1 

We get

x + y = a

It is given that the straight line which is passes through the point (5, 6)

So, the given point satisfy the equation

5 + 6 = a

a = 11

Hence, the equation of the line is x + y = 11

(2)大小相等但符号相反

解决方案：

We have given that,

b = -a

Now we find the equation of line cutoff intercepts from the axes:-

According to the formula of the equation of the line 

x/a + y/b = 1 

We get

x/a + y/-a = 1

x – y = a

It is given that the straight line which is passes through the point (5, 6)

So, the given point satisfy the equation

5 – 6 = a

a = -1

Hence, the equation of the line is x – y = -1

问题4.对于a和b的值是多少，由轴ax + + + 8 = 0在坐标轴上截取的截距的长度相等，但符号与由2x – 3y + 6 = 0截取的截距相反。在轴上。

解决方案：

According to the question

It is given that intercepts cut off on the coordinate axes by the line 

ax + by +8 = 0  …(1)

So the slope of the equations is 

slope(m1) = –a/b

Also, they are equal in length, but opposite in signs to those cut off by the line 

2x – 3y +6 = 0  …(2)

So the slope of the equations is 

slope(m2) = 2/3

So, on solving we get

-a/b = 2/3

a = -2b/3   …..(3)

Now, the length of the perpendicular from the origin.

So, by using the formula, we get

d = |ax + by + c/√a² + b²|

d₁ = |a(0) + b(0) + 8/√a² + b²|

= 8 × 3/√13b²

d₂ = |2(0) – 3(0) + 6/√2² + 3²| 

= 6/√13

As we know that d₁ = d₂,

So, 8 × 3/√13b² = 6/√13

b = 4

Now put the value of b in eq(3), we get

a = -2b/3

 a = -8/3

Hence, the value of a and b is -8/3 and 4

问题5。找到直线上的等式，该等式在轴上截取相等的正截距，其乘积为25。

解决方案：

According to the question

a = b   …..(1)

ab = 25  …..(2)

We have to find the equation of the line which cutoff equal positive intercepts on the axes

So, from eq(1) and (2), we get

a² = 25

a = 5 

According to the formula of the equation of the line 

x/a + y/b = 1 

We get

x/5 + y/5 = 1

Now we’re taking LCM, we get

x + y = 5

Hence, the equation of line is x + y = 5

问题6。找到通过点(-4，3)的直线方程，然后在轴之间截取的直线部分被该点在内部以5：3的比例除。

解决方案：

As we know that the equation of the line is 

x/a + y/b = 1     …..(1)

And it cuts the axis at point A(a, 0) and B(0, b)

So, AB intercept between the axis is 5 : 3

h = 3 × a + 5 × 0/8 

k = 3 × 0 + 5 × 0/8

P = (3a/8, 5b/8)

It is given that the straight line which is passes through the point (-4, 3)

So, 3a/8 = -4 and 5b/8 = 3

a = -32/3 and b = 24/5

Now put the value of a and b in eq(1), we get

-3x/32 + 5y/24 = 1

Hence, the equation of line is 9x – 20y + 96 = 0

问题7.一条直线穿过该点将线之间的线截取成两等分。证明线的等式为x /2α+ y /2β= 1。

解决方案：

As we know that the equation of the line is 

x/a + y/b = 1     …..(1)

And line intercept by the axes are(a, 0) and (0, b),

If the line segment bisect at the point (α, β) then,

a + 0/2 = α

0 + b/2 = β

a = 2α, b = 2β

Now put the value of a and b in eq(1), we get

x/2α + y/β = 1

问题8.找到通过点(3，4)的线方程，使得线在轴之间截取的部分可被点以比例2：3整除。

解决方案：

As we know that the equation of the line is 

x/a + y/b = 1     …..(1)

And let us assume point Q(3, 4) divides the line that point A(a, 0) and B(0, b)

in ratio 2 : 3

So, 2(0) + 3(a)/2 + 3 = 3

2(a) + 3(0)/2 + 3 = 4

a = 5, b = 10

Now put the value of a and b in eq(1), we get

x/5 + y/10 =1

Hence, the equation of line is 2x + y = 10

问题9.点R(h，k)将轴之间的线段按比例1：2划分。找到线方程。

解决方案：

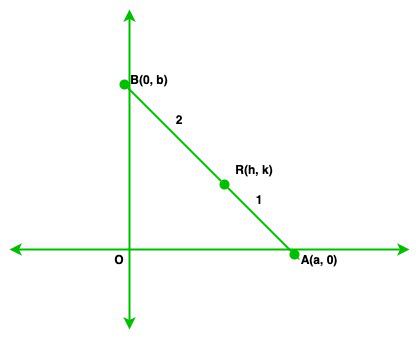
It is given that the point R(h, k) divides a line segment between the axes in the ratio 1 : 2

By using the section formula we get

h = 2 × a + 1 × 0/1 + 2

and,

k = 2 × 0 + 1 × b/1 + 2

So, 

h = 2a/3 and k = b/3

Hence, the value of a and b is 

a = 3h/2

b = 3k

Thus, the corresponding points of A (3h/2, 0) and B (0, 3k)

y – 3k/3k – 0 = x – 0/0 – 3h/2

-3hy + 9hk = 6kx

Hence, the equation of the line is 2kx + hy = 3kh

问题10。找到通过点(-3，8)并在和为7的坐标轴上截断正截距的直线方程。

解决方案：

As we know that the equation of the line is 

x/a + y/b = 1     …..(1)

Then a + b = 7

x/a + y/7 – 1 = 1          …..(2)

It is given that the line passes through point(-3, 8)

= -3/a + 8/7 – a = 1

=-21 + 3a + 8a = 7a – a²

= a² + 4a – 21 = 0

a = 3 or -7

But a > 0 so a not = -7

Now put the value of a and b in eq(1), we get

x/3 + y/4 = 1

Hence, the equation of the line is 4x + 3y = 12