第12类NCERT解决方案-数学第二部分–第9章微分方程-练习-9.2

在第1到第6个问题中，验证给定的函数(显式)是相应微分方程的解：

问题1. y = e ^x + 1：y” – y’= 0

解决方案：

Given: y = e^x + 1

On differentiating we get

y’ = e^x          -(1)

Again differentiating we get

y” = e^x           -(2)

Now substitute the values from equation(1) and (2), in the differential equation 

y” – y’ = e^x – e^x = 0       

Hence verified

问题2。y = x ² + 2x + C：y’– 2x – 2 = 0

解决方案：

Given: y = x² + 2x + C

On differentiating we get

y’ = 2x + 2      

y’ – 2x – 2 = 0   

Hence verified

问题3. y = cosx + c：y’+ sin x = 0

解决方案：

Given: y = cos x + c

On differentiating we get

y’ = -sin x        -(1)

Now substitute the values from equation(1), in the differential equation 

y’ + sin x = 0

-sin x + sin x = 0

0 = 0

Hence verified

问题4。 $y=\sqrt{1+x^{2}}$ ： $y'=\frac{xy}{1+x^{2}}$

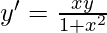
解决方案：

Given: $y=\sqrt{1+x^{2}}$ 

$y'=\frac{1}{2\sqrt{1+x^2}}.2x=\frac{x}{\sqrt{1+x^2}}=\frac{x.\sqrt{1+x^2}}{1+x^2}=\frac{xy}{1+x^2}$

问题5. y = Ax：xy’= y(x≠0)

解决方案：

Given: y = Ax

y/A = x          -(1)

On differentiating we get

y’ = A          -(2)

Now substitute the values from equation(1) and (2), in the differential equation 

xy’ = y

$\frac{y}{A}A$ = y

y = y

Hence verified

问题6：y = x sin x：xy’= y + x 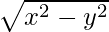$\sqrt{x^2-y^2}$ (x≠0且x> y或x <-y)

解决方案：

Given: y = x.sin x   

On differentiating we get

y’ = x cos x + sin x           -(1)

Now substitute the values from equation(1), in the differential equation 

Taking LHS

xy’ = x(x cos x + sin x)

= x² cos x + x sin x

= x²√1 – sin²x + y

= y + x²$\sqrt{1 - (\frac{y}{x})^2}$

= y + x²$\frac{\sqrt{x^2 - y^2}}{x}$

= y + x$\frac{\sqrt{x^2 - y^2}}{x}$

LHS = RHS

Hence verified

问题7. xy =对数y + C：y’= 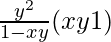$\frac{y^{2}}{1-xy}(xy≠1)$

解决方案：

Given: xy = logy + C          -(1)

On differentiating we get

xy’ + y = $\frac{1}{y}$> y’

xyy’ + y² = y’

xyy’ – y’ = -y²

y'(xy – 1) = -y²

y’ = -y²/ (xy – 1)          -(2)

Now substitute the values from equation(1) and (2), in the differential equation 

y’ = $\frac{y^{2}}{1-xy}$

$\frac{-y^2}{-(1 - xy)} = \frac{y^{2}}{1-xy}$

$\frac{y^2}{1 - xy} = \frac{y^{2}}{1-xy}$

Hence verified

问题8. y – cos y = x：(y sin y + cos y + x)y’= y

解决方案：

Given: y – cos y = x         -(1)

On differentiating we get

y’ – sin y.y’ = 1

y'(1 + sin y) = 1

$y' = \frac{1}{(1 + sin y)}$          -(2)

Now substitute the values from equation(1) and (2), in the differential equation 

(y sin y + cos y + x)y’ = y

$(y sin y + cos y + y - cosy)\frac{1}{1+siny} = y$

$(y sin y + y)\frac{1}{1+siny} = y$

$y(sin y + 1)\frac{1}{1+siny} = y$

y = y

Hence verified

问题9. x + y = tan ^-1 y：y ² y’+ y ² +1 = 0

解决方案：

Given: x + y = tan^-1y

On differentiating we get

1 + y’ = $\frac{1}{1+y^2}y'$

$y'[\frac{1}{1+y^2}-1]=1$

$y'[\frac{1-1+y^2}{1+y^2}]=1$

$y'[\frac{-y^2}{1+y^2}]=1$

$y'=\frac{1}{\frac{-y^2}{1+y^2}}$

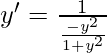
$y'=\frac{-(1+y^2)}{y^2}$          -(1)

Now substitute the values from equation(1), in the differential equation 

y²y’ + y² + 1 = 0

$y^2[\frac{-(1+y^2)}{y^2}] + y^2 + 1 = 0$

$-1-y^2 + y^2 + 1 = 0$

0 = 0

Hence verified

问题10。 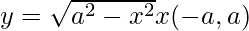$y=\sqrt{a^2-x^2}x∈(-a,a)$ ： $x+y\frac{dy}{dx}=0(y≠0)$

解决方案：

Given: $y=\sqrt{a^2-x^2}$

We can also write as 

y² = a² – x²

Now on differentiating we get

2yy’ = -2x

y’ = -2x/2y

y’ = -x/y         -(1)

Now substitute the values from equation(1), in the differential equation 

x + y.$\frac{dy}{dx}=0$

x + y (-x/y) = 0

x – x = 0

0 = 0

Hence verified

问题11。四阶微分方程的一般解中的任意常数的个数是

(A)0(B)2(C)3(D)4

解决方案：

(D) is correct answer because the number of constants in the general solution of a differential equation of order n is equal to its order.

问题12。在三阶微分方程的特定解中的任意常数的数量为：

(A)3(B)2(C)1(D)0

解决方案：

(D) is the correct answer because there are no arbitrary constants in a particular solution.