第12类NCERT解决方案-数学第I部分–第4章行列式–练习4.3

问题1.在以下每个给定的点上找到具有顶点的三角形区域：

(i)(1、0)，(6、0)，(4、3)

(ii)(2、7)，(1、1)，(10、8)

(iii)(–2，–3)，(3，2)，(–1，–8)

解决方案：

(i) (1, 0), (6, 0), (4, 3) 

$Area\ of\ triangle=\frac{1}{2}\begin{vmatrix}1 & 0 & 1\\6&0&1\\4&3&1\end{vmatrix}\\=\frac{1}{2}[1(0-3)-0(6-4)+1(18-0)]\\=\frac{15}{2}square\ units$

(ii) (2, 7), (1, 1), (10, 8) 

$Area\ of\ triangle=\frac{1}{2}\begin{vmatrix}2 & 7 & 1\\1&1&1\\10&8&1\end{vmatrix}\\=\frac{1}{2}[2(1-8)-7(1-10)+1(8-10)]\\=\frac{47}{2}square\ units$

(iii) (–2, –3), (3, 2), (–1, –8) 

$Area\ of\ triangle=\frac{1}{2}\begin{vmatrix}-2 & -3 & 1\\3&2&1\\-1&-8&1\end{vmatrix}\\=\frac{1}{2}[-2(10)+3(4)-22]\\=15\ square\ units$

问题2。证明点A(a，b + c)，B(b，c + a)，C(c，a + b)是共线的。

解决方案：

If the area of the triangle is equal to zero then the points are collinear. 

For example:- Area of triangle = 0 

$Area\ of\ triangle=\frac{1}{2}\begin{vmatrix}a&b+c&1\\b&c+a&1\\c&a+b&1\end{vmatrix}\\ =\frac{1}{2}[a(c+a-a-b)-(b+c)(b-c)+1\{b(a+b)-c(c+a)\}]\\=\frac{1}{2}(ac-ab-b^2+c^2+ab+b^2-c^2-ac)\\0\\Hence,\ the\ points\ are\ collinear\ as\ the\ area\ of\ triangle\ is\ equal\ to\ zero$

问题3.如果三角形的面积是4平方单位，并且顶点是

(i)(k，0)，(4，0)，(0，2)
(ii)(-2，0)，(0，4)，(0，k)

解决方案：

(i) (k, 0), (4, 0), (0, 2) 

Given: Area of triangle = ±4 sq. units 

i.e. $\frac{1}{2}\begin{vmatrix}x_1&y_1&1\\x_2&y_2&1\\x_3&y_3&1\end{vmatrix}=4\\\frac{1}{2}[k(0-2)-0+1(8-0)]=4\\\frac{1}{2}(-2k+4)=4\\-k+4=4\\Here, -k+4=\pm4\\if,\\-k+4=4\ and-k+4=-4\\k=0\ and\ k=8$

(ii) (-2, 0), (0, 4), (0, k)

$\frac{1}{2}\begin{vmatrix}x_1&y_1&1\\x_2&y_2&1\\x_3&y_3&1\end{vmatrix}=4\\\frac{1}{2}\begin{vmatrix}-2&0&1\\0&4&1\\0&k&1\end{vmatrix}\\\frac{1}{2}(-8+2k)=4\\-k+4=4\\Here, -k+4=\pm4\\if,\\-k+4=4\ and-k+4=-4\\k=0\ and\ k=8$

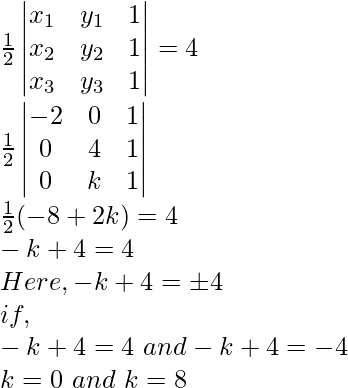
问题4。(i)使用行列式找到线连接(1，2)和(3，6)的方程。
(ii)使用行列式找到线连接(3，1)和(9，3)的方程式。

解决方案：

(i) Find equation of line joining (1, 2) and (3, 6) using determinants. 

Let us assume, 

A(x, y) be any vertex of a triangle 

All points are on one line (collinear) if the area of triangle is zero. 

$\frac{1}{2}\begin{vmatrix}1&2&1\\3&6&1\\x&y&1\end{vmatrix}=0\\\frac{1}{2}[x(2-6)-y(1-3)+1(6-6)]=0\\-4x+2y=0\\y=2x\\Which\ is\ equation\ of\ a\ line.$

(ii) Find equation of line joining (3, 1) and (9, 3) using determinants. 

Let us assume, 

A(x, y) be any vertex of a triangle 

All points are on one line (collinear) if the area of triangle is zero. 

$\frac{1}{2}\begin{vmatrix}x&y&1\\3&1&1\\9&3&1\end{vmatrix}\\\frac{1}{2}[x(1-3)-y(3-9)+1(9-9)]=0\\-2x+6y=0\\x-3y=0\\It\ is\ a\ equation\ of\ line.$

问题5.如果三角形的面积是35平方单位，且顶点分别为(2，– 6)，(5，4)和(k，4)。那么k是
(A)12(B)–2(C)–12，–2(D)12，–2

解决方案：

(D) is the correct option. 

As: 

$\frac{1}{2}\begin{vmatrix}x_1&y_1&1\\x_2&y_2&1\\x_3&y_3&1\end{vmatrix}=35\\\frac{1}{2}[2(4-4)-(-6)(5-k)+1(20-4k)=35\\By\ solving\ we\ will\ get\\25-5k=\pm35\\25-5k=35\ and\ 25-5k=-35\\k=-2\ and\ k=12.$