第12类RD Sharma解–第22章微分方程–练习22.7 |套装1

解决以下微分方程：

问题1.(x – 1)(dy / dx)= 2xy

解决方案：

We have,

(x – 1)(dy/dx) = 2xy     

dy/y = [2x/(x – 1)]dx

On integrating both sides,

∫(dy/y) = ∫[2x + (x – 1)]dx

log(y) = ∫[2 + 2/(x – 1)]dx

log(y) = 2x + 2log(x – 1) + c (Where ‘c’ is integration constant)

问题2.(x ² +1)dy = xydx

解决方案：

We have,

(x² + 1)dy = xydx     

(dy/y) = [x/(x² + 1)]dx

On integrating both sides

∫(dy/y) = ∫[x/(x² + 1)]dx

log(y) = (1/2)∫[2x/(x² + 1)]dx

log(y) = (1/2)log(x² + 1) + c (Where ‘c’ is integration constant)

问题3.(dy / dx)=(e ^x + 1)y

解决方案：

We have,

(dy/dx) = (e^x + 1)y        

(dy/y) = (e^x + 1)dx

On integrating both sides

∫(dy/y) = ∫(e^x + 1)dx

log(y) = (e^x + x) + c (Where ‘c’ is integration constant)

问题4.(x – 1)(dy / dx)= 2x ³ y

解决方案：

We have,

 (x – 1)(dy/dx) = 2x³y     

(dy/y) = [2x³/(x – 1)]dx

On integrating both sides

∫(dy/y) = ∫[2x³/(x – 1)]dx

∫(dy/y) = 2∫[x² + x + 1 + 1/(x – 1)]dx

log(y) = (2/3)(x³) + x² + 2x + 2log(x – 1) + c (Where ‘c’ is integration constant)

问题5. xy(y + 1)dy =(x ² +1)dx

解决方案：

We have,

xy(y + 1)dy = (x² + 1)dx  

y(y + 1)dy = [(x² + 1)/x]dx

(y² + y)dy = xdx + (dx/x)

On integrating both sides,

∫(y² + y)dy = ∫xdx + (dx/x)

(y³/3) + (y²/2) = (x²/2) + log(x) + c (Where ‘c’ is integration constant)

问题6. 5(dy / dx)= e ^x y ⁴

解决方案：

We have,

5(dy/dx) = e^xy⁴          

5(dy/y⁴) = e^x  

On integrating both sides,

5∫(dy/y⁴) = ∫e^x 

-(5/3)(1/y³) = e^x + c (Where ‘c’ is integration constant)

问题7. xcosydy =(xe ^x logx + e ^x )dx

解决方案：

We have,

xcosydy = (xe^xlogx + e^x)dx       

cosydy = e^x(logx + 1/x)dx

On integrating both sides,

∫cosydy = ∫e^x(logx + 1/x)dx

Since, ∫[f(x) + f'(x)]e^xdx] = e^xf(x)

siny = e^xlogx + c (Where ‘c’ is integration constant)

问题8.(dy / dx)= e ^{x + y} + x ² e ^y

解决方案：

We have,

(dy/dx) = e^x+y + x²e^y             

(dy/dx) = e^xe^y + x²e^y 

dy = e^y(e^x + x²)dx

e^-ydy = (e^x + x²)dx

On integrating both sides,

∫e^-ydy = ∫(e^x + x²)dx

-e^-y = e^x + (x³/3) + c (Where ‘c’ is integration constant)

问题9. x(dy / dx)+ y = y ²

解决方案：

We have,

x(dy/dx) + y = y²      

x(dy/dx) = y² – y

[1/(y² – y)]dy = dx/x

On integrating both sides,

∫[1/(y² – y)]dy = ∫dx/x

∫[1/(y – 1) – 1/y]dy = ∫(dx/x)

log(y-1) – log(y) = logx + logc

log[(y – 1)/y] = log[xc]

(y – 1)/y = xc

(y-1) = yxc (Where ‘c’ is integration constant)

问题10.(e ^y + 1)cosxdx + e ^y sinxdy = 0

解决方案：

We have,

(e^y + 1)cosxdx + e^ysinxdy = 0          

(cosx/sinx)dx = -[e^y/(e^y + 1)]dy

On integrating both sides,

∫(cosx/sinx)dx = -∫[e^y/(e^y + 1)]dy

log(sinx) = -log(e^y + 1) + log(c)

log(sinx) + log(e^y + 1) = log(c)

log[sinx(e^y + 1)] = log(c)

sinx(e^y + 1) = c (Where ‘c’ is integration constant)

问题11. xcos ² ydx = ycos ² xdy

解决方案：

We have,

xcos²ydx = ycos²xdy      

(x/cos²x)dx = (y/cos²y)dy

xsec²xdx = ysec²ydy

On integrating both sides,

∫xsec²xdx = ∫ysec²ydy

$x∫sec^2x - ∫[\frac{d(x)}{dx(x)}∫sec^2xdx]dx = y∫sec^2x-∫[\frac{d(y)}{dy}(y)∫sec^2y]dy$

xtanx – ∫tanxdx = ytany – ∫tanydy

xtanx – log(secx) = ytany – log(secy) + c (Where ‘c’ is integration constant)

问题12. xydy =(y – 1)(x + 1)dx

解决方案：

We have,

xydy = (y – 1)(x + 1)dx          

[y/(y – 1)]dy = [(x + 1)/x]dx

On integrating both sides,

∫[y/(y – 1)]dy = ∫[(x + 1)/x]dx

∫[1 + 1/(y – 1)]dy = ∫[(x + 1)/x]dx

y + log(y – 1) = x + log(x) + c

y – x = log(x) – log(y – 1) + c (Where ‘c’ is integration constant)

问题13. x(dy / dx)+ coty = 0

解决方案：

We have,

x(dy/dx) + coty = 0          

x(dy/dx) = -coty

dy/coty = -(dx/x)

tanydy = -(dx/x)

On integrating both sides,

∫tanydy = -∫(dx/x)

log(secy) = -log(x) + log(c)

log(secy) + log(x) = log(c)

log(xsecy) = log(c)

x/cosy = c

x = c * cosy (Where ‘c’ is integration constant)

问题14.(dy / dx)=(xe ^x logx + e ^x )/(xcosy)

解决方案：

We have,

(dy/dx) = (xe^xlogx + e^x)/(xcosy)         

xcosydy = (xe^xlogx + e^x)dx

cosydy = e^x(logx + 1/x)dx

On integrating both sides,

∫cosydy = ∫e^x(logx + 1/x)dx

Since, ∫[f(x) + f'(x)]e^xdx] = e^xf(x)

siny = e^xlogx + c (Where ‘c’ is integration constant)

问题15(dy / dx)= e ^{x + y} + x ³ e ^y

解决方案：

We have,

(dy/dx) = e^x+y + x³e^y   

(dy/dx) = e^xe^y + x³e^y

dy = e^y(e^x + x³)dx

e^-ydy = (e^x + x³)dx

On integrating both sides,

∫e^-ydy = ∫(e^x + x³)dx

-e^-y = e^x + (x⁴/4) + c

e^-y + e^x + (x⁴/4) = c  (Where ‘c’ is integration constant)

问题16.y√(1 + x ² )+x√(1 + y ² )(dy / dx)= 0

解决方案：

We have,

y√(1 + x²) + √(1 + y²)(dy/dx) = 0        

y√(1 + x²)dx = -x√(1 + y²)dy

$\frac{\sqrt{1+y^2}}{y}dy=-\frac{\sqrt{1+x^2}}{x}dx$

$\frac{y\sqrt{1+y^2}}{y^2}dy=-\frac{x\sqrt{1+x^2}}{x^2}dx$

On integrating both sides,

$∫\frac{y\sqrt{1+y^2}}{y^2}dy=-∫\frac{x\sqrt{1+x^2}}{x^2}dx$

Let, 1 + y² = z²  

On differentiating both sides

2ydy = 2zdz

ydy = zdz

= $∫\frac{y\sqrt{1+y^2}}{y^2}dy$

= $∫\frac{zdz*z}{z^2-1}$

= ∫[z²/(z² – 1)]dz

= ∫[1 + 1/(z² – 1)]dz

= z + (1/2)log[(z – 1)/(z + 1)]

On putting the value of z in above equation

= $\sqrt{1+y^2}+\frac{1}{2}log[\frac{\sqrt{1+y^2}-1}{\sqrt{1+y^2}+1}]$

Similarly,

$∫\frac{x\sqrt{1+x^2}}{x^2}dx$ = $\sqrt{1+x^2}+\frac{1}{2}log[\frac{\sqrt{1+x^2}-1}{\sqrt{1+x^2}+1}]$

$\sqrt{1+y^2}+\frac{1}{2}log[\frac{\sqrt{1+y^2}-1}{\sqrt{1+y^2}+1}]=-\sqrt{1+x^2}-\frac{1}{2}log[\frac{\sqrt{1+x^2}-1}{\sqrt{1+x^2}+1}]+c$

$\sqrt{1+y^2}+\sqrt{1+x^2}+\frac{1}{2}log[\frac{\sqrt{1+y^2}-1}{\sqrt{1+y^2}+1}]+\frac{1}{2}log[\frac{\sqrt{1+x^2}-1}{\sqrt{1+x^2}+1}]=c$(Where ‘c’ is integration constant)

问题17.√(1 + x ² )(dy)+√(1 + y ² )dx = 0

解决方案：

We have,

√(1 + x²)(dy) + √(1 + y²)dx = 0                  

$\frac{dy}{\sqrt{(1+y^2)}}=\frac{-dx}{\sqrt{(1+x^2)}}$

On integrating both sides,
log[y + √(1 + y²)] = -log[x + √(1 + x²)] + logc

log[y + √(1 + y²)] + log[x + √(1 + x²)] = logc

log([y + √(1 + y²)][x + √(1 + x²)]) = logc

[y + √(1 + y²)][x + √(1 + x²)] = c  (Where ‘c’ is integration constant)

问题18。 $\sqrt{(1 + x^2 + y^2 + x^2y^2)} + xy(\frac{dy}{dx}) = 0$

解决方案：

We have,

$\sqrt{(1 + x^2 + y^2 + x^2y^2)} + xy(\frac{dy}{dx}) = 0$

$\sqrt{[(1 + x^2) + y^2(1+x^2)]}+xy(\frac{dy}{dx})=0$

$\sqrt{[(1+x^2)(1+y^2)]}+xy(\frac{dy}{dx})=0$

$\frac{y}{\sqrt{1+y^2}}dy=-\frac{\sqrt{1+x^2}}{x}dx$

$\frac{y}{\sqrt{1+y^2}}dy=-\frac{x\sqrt{1+x^2}}{x^2}dx$

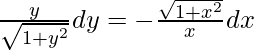
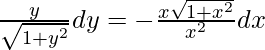
On integrating both sides,

$∫\frac{y}{\sqrt{1+y^2}}dy=-∫\frac{x\sqrt{1+x^2}}{x^2}dx$

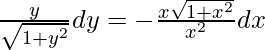
Let, 1 + x² = z²  

On differentiating both sides

2xdx = 2zdz

xdx = zdz

= $-∫\frac{x\sqrt{1+x^2}}{x^2}dy$

= $-∫\frac{zdz*z}{z^2-1}$

= -∫[z²/(z² – 1)]dz

= -∫[1 + 1/(z² – 1)]dz

= -z – (1/2)log[(z – 1)/(z + 1)]

On putting the value of z in above equation

$=-\sqrt{1+x^2}-\frac{1}{2}log[\frac{\sqrt{1+x^2}-1}{\sqrt{1+x^2}+1}]$

Let, 1 + y² = v²  

On differentiating both sides

2ydy = 2vdv

ydy = vdv

= ∫(vdv/v)

= v

On putting the value of v in above equation

= √(1 + y²)

= $\sqrt{1+y^2}=-\sqrt{1+x^2}-\frac{1}{2}log[\frac{\sqrt{1+x^2}-1}{\sqrt{1+x^2}+1}]+c$

= $\sqrt{1+y^2}+\sqrt{1+x^2}+\frac{1}{2}log[\frac{\sqrt{1+x^2}-1}{\sqrt{1+x^2}+1}]=c$ (Where ‘c’ is integration constant)

问题19。 $(\frac{dy}{dx}) = \frac{e^x(sin^2x + sin2x)}{y(2logy+1)}$

解决方案：

We have,

$(\frac{dy}{dx}) = \frac{e^x(sin^2x + sin2x)}{y(2logy+1)}$

y(2logy + 1)dy = e^x(sin²x + sin2x)dx

On integrating both sides,

∫y(2logy + 1)dy = ∫e^x(sin²x + sin2x)dx

$2logy*∫ydy-∫[2\frac{d}{dx}(logy)∫ydy]dy+\frac{y^2}{2}=e^xsin^2x+c$

Since, ∫e^x(sin²x + sin2x)dx = e^xsin²x 

Using property ∫[f(x) + f'(x)]e^x = e^xf(x)

y²log(y) – ∫ydy + y²/2 = e^xsin²x + c

y²log(y) – y²/2 + y²/2 = e^xsin²x + c

y²log(y) = e^xsin²x + c  (Where ‘c’ is integration constant)

问题20.(dy / dx)= x(2logx +1)/(窦性+黏性)

解决方案：

We have,

(dy/dx) = x(2logx + 1)/(siny + ycosy)        

(siny + ycosy)dy = x(2logx + 1)dx

On integrating both sides,

∫(siny + ycosy)dy = ∫x(2logx + 1)dx

∫sinydy + y∫cosydy – ∫{(dy/dy)∫cosydy}dy = 2logx∫xdx – 2∫{$\frac{d(logx)}{dx}$∫xdx} + ∫xdx

-cosy + ysiny – ∫sinydy = x²logx – ∫xdx + (x²/2) + c

-cosy + ysiny + cosy = x²logx – (x²/2) + (x²/2) + c

ysiny = x²logx + c (Where ‘c’ is integration constant)