第12类NCERT解决方案–数学第I部分–第4章行列式–练习4.6 |套装2

第4章行列式–练习4.6 |套装1

问题11. 2x + y + z = 1

x – 2y – z = 3/2

3y – 5z = 9

解决方案：

Matrix form of the given equation is AX = B

i.e.$\begin{bmatrix}2 & 1 & 1\\1 & -2 & -1\\0 & 3 & -5\end{bmatrix}\begin{bmatrix}x\\y\\z\end{bmatrix}=\begin{bmatrix}1\\3/2\\9\end{bmatrix}$

∴ |A| =$\begin{vmatrix}2 & 1 & 1\\1 & -2 & -1\\0 & 3 & -5\end{vmatrix}=2(10+3)-1(-5-0)+1(3-0)=26+5+3≠0$

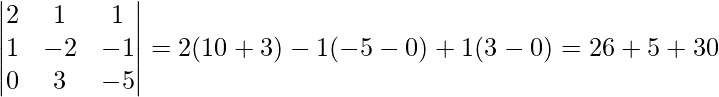
∴ Solution is unique.

Now, X = A^-1B = $\frac{1}{|A|}$(adj.A)B

$\begin{bmatrix}x\\y\\z\end{bmatrix}=\frac{1}{34}\begin{bmatrix}13 & 8 & 1\\5 & -10 & 3\\3 & -6 & -5\end{bmatrix}\begin{bmatrix}1\\3/2\\ 9\end{bmatrix} =\frac{1}{34}\begin{bmatrix}13+12+9\\5-15+27\\3-9-45\end{bmatrix}=\frac{1}{34}\begin{bmatrix}34\\17\\-51\end{bmatrix}=\begin{bmatrix}1\\1/2\\-3/2\end{bmatrix}$

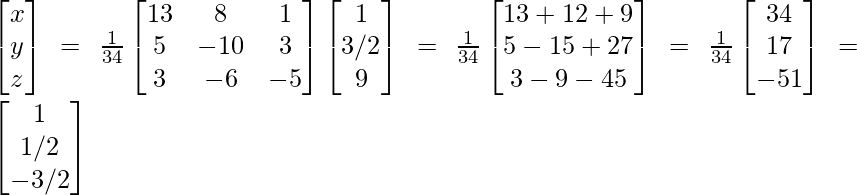
Therefore, x=1, y=1/2, z=3/2

问题12. x – y + z = 4

2x + y – 3z = 0

x + y + z = 2

解决方案：

Matrix form of the given equation is AX = B

i.e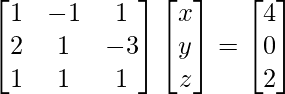$\begin{bma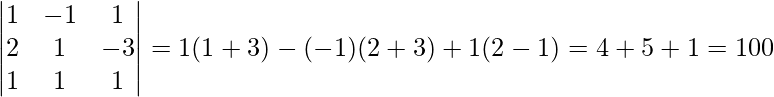trix}1 & -1 & 1\\2 & 1 & -3\\1 & 1 & 1\end{bmatrix}\begin{bmatrix}x\\y\\z\end{bmatrix}=\begin{bmatrix}4\\0\\2\end{bmatrix}$

∴ |A| =$\begin{vmatrix}1 & -1 & 1\\2 & 1 & -3\\1 & 1 & 1\end{vmatrix}=1(1+3)-(-1)(2+3)+1(2-1)=4+5+1=10≠0$

∴ Solution is unique.

Now, X = A^-1B =$\frac{1}{|A|}$ (adj.A)B

$\begin{bmatrix}x\\y\\z\end{bmatrix}=\frac{1}{10}\begin{bmatrix}4 & 2 & 2\\-5 & 0 & 5\\1 & -2 & 3\end{bmatrix}\begin{bmatrix}4\\0\\ 2\end{bmatrix} =\frac{1}{10}\begin{bmatrix}16+0+4\\-20+0+10\\4-0+6\end{bmatrix}=\frac{1}{10}\begin{bmatrix}20\\-10\\10\end{bmatrix}=\begin{bmatrix}2\\-1\\ 1\end{bmatrix}$

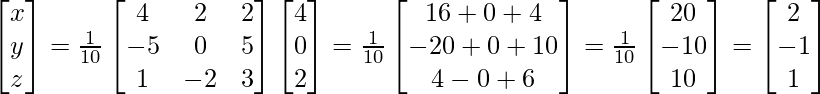
Therefore, x = 2, y = -1, z = 1

问题13. 2x + 3y +3 z = 5

x – 2y + z = – 4

3x – y – 2z = 3

解决方案：

Matrix form of given equation is AX = B

i.e.$\begin{bmatrix}2 & 3 & 3\\1 & -2 & -1\\3 & -1 & -2\end{bmatrix}\begin{bmatrix}x\\y\\z\end{bmatrix}=\begin{bmatrix}5\\-4\\3\end{bmatrix}$

∴ |A| =$\begin{vmatrix}2 & 3 & 3\\1 & -2 & -1\\3 & -1 & -2\end{vmatrix}=2(4+1)-3(-2-3)+3(-1+6)=10+15+15≠0$

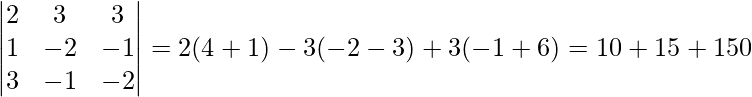
∴ Solution is unique.

Now, X = A^-1B = $\frac{1}{|A|}$(adj.A)B

$\begin{bmatrix}x\\y\\z\end{bmatrix}=\frac{1}{40}\begin{bmatrix}5 & 3 & 9\\5 & -13 & 1\\5 & 11 & -7\end{bmatrix}\begin{bmatrix}5\\-4\\ 3\end{bmatrix} =\frac{1}{10}\begin{bmatrix}25-12+27\\25+52+3\\25-44-21\end{bmatrix}=\frac{1}{10}\begin{bmatrix}40\\80\\-40\end{bmatrix}=\begin{bmatrix}1\\2\\ -1\end{bmatrix}$

Therefore, x = 1, y = 2, z = -1

问题14. x – y + 2z = 7

3x + 4y – 5z = – 5

2x – y + 3z = 12

解决方案：

Matrix form of given equation is AX = B

i.e.$\begin{bmatrix}1 & -1 & 2\\3 & 4 & -5\\2 & -1 & 3\end{bmatrix}\begin{bmatrix}x\\y\\z\end{bmatrix}=\begin{bmatrix}7\\-5\\12\end{bmatrix}$

∴ |A| =$\begin{vmatrix}1 & -1 & 2\\3 & 4 & -5\\2 & -1 & 3\end{vmatrix}=1(12-5)-(-1)(9+10)+2(-3-8)=7+9-22=4≠0$

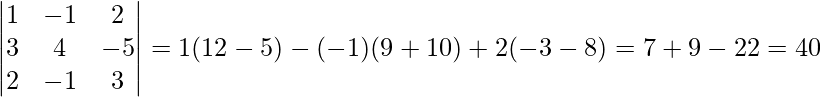
∴ Solution is unique.

Now, X = A^-1B =$\frac{1}{|A|}$ (adj.A)B

$\begin{bmatrix}x\\y\\z\end{bmatrix}=\frac{1}{4}\begin{bmatrix}7 & 1 & -3\\-19 & -1 & 11\\-11 & -1 & 7\end{bmatrix}\begin{bmatrix}7\\-5\\ 12\end{bmatrix} =\frac{1}{4}\begin{bmatrix}49-5-36\\-133+5+132\\-77+5+84\end{bmatrix}=\frac{1}{4}\begin{bmatrix}8\\4\\12\end{bmatrix}=\begin{bmatrix}2\\1\\ 3\end{bmatrix}$

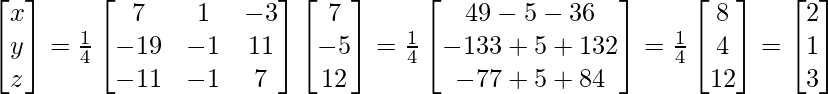
Therefore, x = 2, y = 1, z = 3

问题15：如果A = 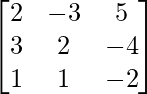$\begin{bmatrix}2 & -3 & 5\\3 & 2 & -4\\1 & 1 & -2\end{bmatrix}$ ，找到A–1。使用A–1求解方程组

2x – 3y + 5z = 11

3x + 2y – 4z = – 5

x + y – 2z = – 3

解决方案：

Given: A=$\begin{bmatrix}2 & -3 & 5\\3 & 2 & -4\\1 & 1 & -2\end{bmatrix}$

Now, |A|= $\begin{vmatrix}2 & -3 & 5\\3 & 2 & -4\\1 & 1 & -2\end{vmatrix}$

∴ |A|= $2(-4+4)-(-3)(-6+4)+5(3-2)=0-6+5= -1≠0$

Means, A^-1 exists.

And A^-1 =$\frac{1}{|A|}$(adj.A)……(1)

Now,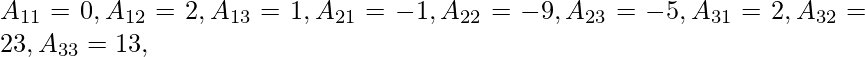$A_{11}=0,A _{12}=2,A _{13}=1, A _{21}=-1,A _{22}=-9,A _{23}=-5, A _{31}=2,A _{32}=23,A _{33}=13,$

∴ adj. A =$\begin{bmatrix}0 & -1 & 2\\2 & -9 & 23\\1 & -5 & 13\end{bmatrix}$

From eq. (1),

A^-1=$\frac{1}{-1}\begin{bmatrix}0 & -1 & 2\\2 & -9 & 23\\1 & -5 & 13\end{bmatrix}=\begin{bmatrix}0 & 1 & -2\\-2 & 9 & -23\\-1 & 5 & -13\end{bmatrix}$

Now, Matrix form of given equation is AX = B

i.e.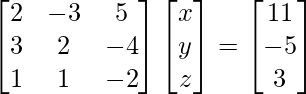$\begin{bmatrix}2 & -3 & 5\\3 & 2 & -4\\1 & 1 & -2\end{bmatrix}\begin{bmatrix}x\\y\\z\end{bmatrix}=\begin{bmatrix}11\\-5\\3\end{bmatrix}$

∵ Solution is unique.

∴ X=A^-1B

⇒$\begin{bmatrix}x\\y\\z\end{bmatrix}=\begin{bmatrix}0 & 1 & -2\\-2 & 9 & -23\\-1 & 5 & -13\end{bmatrix}\begin{bmatrix}11\\-5\\3\end{bmatrix} =\begin{bmatrix}0-5+6\\-22-45+69\\-11-25+39\end{bmatrix} =\begin{bmatrix}1\\2\\3\end{bmatrix}$

Therefore, x = 1, y = 2, z = 3

问题16. 4公斤洋葱，3公斤小麦和2公斤大米的价格为60卢比。 2公斤洋葱，4公斤小麦和6公斤大米的价格为90卢比。 6公斤洋葱，2公斤小麦和3公斤大米的价格为70卢比。通过矩阵法查找每公斤的每件商品成本。

解决方案：

Let Rs x, Rs y, Rs z per kg be the prices of onion, wheat and rice respectively.

A.T.Q.

4x+3y+2z=60

2x+4y+6z=90

6x+2y+3z=70

Matrix form of equation is AX = B

where, A=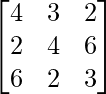$\begin{bmatrix}4 & 3 & 2\\2 & 4 & 6\\6 & 2 & 3\end{bmatrix}$,B=$\begin{bmatrix}60\\90\\70\end{bmatrix}$and X=$\begin{bmatrix}x\\y\\z\end{bmatrix}$

=> $\begin{bmatrix}4 & 3 & 2\\2 & 4 & 6\\6 & 2 & 3\end{bmatrix}\begin{bmatrix}x\\y\\z\end{bmatrix}=\begin{bmatrix}60\\90\\70\end{bmatrix}$

Now, |A|=$\begin{vmatrix}4 & 3 & 2\\2 & 4 & 6\\6 & 2 & 3\end{vmatrix}=4(12-12)-3(6-36)+2(4-24)=0+90-40=50 ≠0$

∴ Solution is unique

Now, X=A^-1B=$\frac{1}{|A|}$(adj. A)B……(1)

Now, $A_{11}=0,A _{12}=30,A _{13}=-20, A _{21}=-5,A _{22}=0,A _{23}=10, A _{31}=10,A _{32}=-20,A _{33}=10,$

∴ (adj.A)= $\begin{bmatrix}0 & -5 & 10\\30 & 0 & -20\\-20 & 10 & 10\end{bmatrix}$

From eqⁿ.(1)

$\begin{bmatrix}x\\y\\z\end{bmatrix}=\frac{1}{50}\begin{bmatrix}0 & -5 & 10\\30 & 0 & -20\\-20 & 10 & 10\end{bmatrix}\begin{bmatrix}60\\90\\70\end{bmatrix} =\frac{1}{50}\begin{bmatrix}-450+700\\1800-1400\\-1200+900+700\end{bmatrix}=\frac{1}{50}\begin{bmatrix}250\\400\\400\end{bmatrix} =\begin{bmatrix}5\\8\\8\end{bmatrix}$

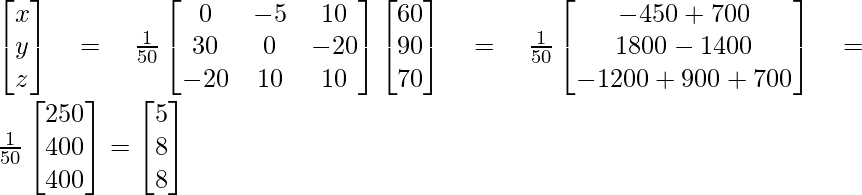
Therefore, x = 5, y = 8, z = 8

Hence, the cost of onion, wheat and rice are Rs. 5, Rs 8 and Rs 8 per kg.