第9类RD Sharma解决方案–第六章多项式的因式分解-练习6.3

在下面的每一个中使用余数定理，找到将f(x)除以g(x)时的提醒并通过实际除法进行验证：(1-8)

问题1. f(x)= x ³ + 4x ² -3x + 10，g(x)= x + 4

解决方案：

Given:f(x)=x³+4x²-3x+10, g(x)=x+4

from, the remainder theorem when f(x) is divided by g(x) =x-(-4) the remainder will be equal to f(-4).

Let, g(x)=0

⇒ x+4=0

⇒ x = -4

Substitute the value of x in f(x)

f(-4)=(-4)³+4(-4)²-3(-4)+10

= -64+(4*16)+12+10

= -64 +64 +12+10

= 22

Therefore, the remainder is 22.

问题2. f(x)= 4x ⁴ -3x ^{3 -2x 2} + x-7，g(x)= x-1

解决方案：

Given:f(x)= 4x⁴-3x³-2x²+x-7, g(x)=x-1

from, the remainder theorem when f(x) is divided by g(x) = x-(1) the remainder will be equal to f(1)

Let, g(x)=0

⇒ x-1=0

⇒ x=1

Substitute the value of x in f(x)

f(1)= 4(1)⁴-3(1)³-2(1)²+1-7

= 4-3-2+1-7

= 5-12

= -7

Therefore, the reminder is 7.

问题3. f(x)= 2x ⁴ -6x ³ + 2x ² -x + 2，g(x)= x + 2

解决方案：

Given: f(x)=2x⁴-6x³+2x²-x+2, g(x)=x+2

from, the remainder theorem when f(x) is divided by g(x) = x-(-2) the remainder will be equal to f(-2)

Let, g(x)=0

⇒ x+2=0

⇒ x=-2

Substitute the value of x in f(x)

f(-2)=2(-2)⁴-6(-2)³+2(-2)²-(-2)+2

= (2*16)-(6*(-8))+(2*4)+2+2

= 32+48+8+2+2

= 92

Therefore, the reminder is 92.

问题4. f(x)= 4x ³ -12x ² + 14x-3，g(x)= 2x-1

解决方案：

Given:f(x)=4x³-12x²+14x-3, g(x)=2x-1

from, the remainder theorem when f(x) is divided by g(x) = 2(x-1/2) the remainder will be equal to f(1\2)

Let, g(x)=0

⇒ 2x-1=0

⇒ x=-1/2

Substitute the value of x in f(x)

$f(\frac12)=4(\frac12)^3-12(\frac12)^2+14(\frac12)-3$

=$4(\frac18)-12(\frac14)+4(\frac12)-3$

=$\frac12 -3 +7-3$

=$\frac12+1$

=$\frac32$

Therefore, the reminder is$\frac32$

问题5. f(x)= x ³ -6x ² + 2x-4，g(x)= 1-2x

解决方案：

Given:f(x)=x³-6x²+2x-4, g(x)=1-2x

from, the remainder theorem when f(x) is divided by g(x) = -2(x-1/2) the remainder will be equal to f(1\2)

Let, g(x)=0

⇒ 1-2x=0

⇒ x=1/2

substitute the value of x in f(x)

$f(\frac12)=(\frac12)^3-6(\frac12)^2+2(\frac12)-4$

=$\frac18-8(\frac14)+2(\frac12)-4$

=$\frac18-(\frac12)+1-4$

=$\frac18-\frac12-3$

Taking L.C.M

=$\frac{1-4+8-32}{8}$

=$\frac{1-36}8$

=$\frac{-35}{8}$

Therefore, the remainder is$\frac{-35}{8}$

问题6. f(x)= x ⁴ -3x ² +4，g(x)= x-2

解决方案：

Given:f(x)=x⁴-3x²+4, g(x)=x-2

from, the remainder theorem when f(x) is divided by g(x) = x-(2) the remainder will be equal to f(2)

Let, g(x)=0

⇒ x-2=0

⇒ x=2

Substitute the value of x in f(x)

f(2)=2⁴-3(2)²+4

= 16-3(4) + 4

= 16 – 12 + 4

= 20 – 12

= 8

Therefore, the remainder is 8

问题7. f(x)= 9x ³ -3x ² + x-5，g(x)= 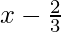$x-\frac23$

解决方案：

Given:f(x)=9x³-3x²+x-5, g(x)=$x-\frac23$

from, the remainder theorem when f(x) is divided by g(x) = x-($\frac23$) the remainder will be equal to f($\frac23$)

Let, g(x)=0

⇒ x-2/3=0

⇒ x=2/3

substitute the value of x in f(x)

$f(\frac23)=9(\frac23)^3-3(\frac23)^2+(\frac23)-5$

=$9(\frac{8}{27})-3(\frac49)+\frac23-5$

=$\frac83-\frac43+\frac23-5$

=$\frac{10-19}{3}$

= -3

Therefore, the remainder is -3

问题8. f(x)= 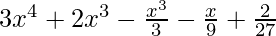$3x^4+2x^3-\frac{x^3}{3}-\frac{x}{9}+\frac{2}{27}$ ，g(x)= $x+\frac23$

解决方案：

Given:$f(x)=3x^4+2x^3-\frac{x^3}{3}-\frac{x}{9}+\frac{2}{27}$,$g(x)=x+\frac23$

from, the remainder theorem when f(x) is divided by g(x) = x-(-\frac23) the remainder will be equal to f($-\frac23$)

substitute the value of x in f(x)

$f(-\frac23)=3(-\frac23)^4+2(-\frac23)^3- \frac{(-\frac23)^3}{3}-\frac{-\frac{2}{3}}{9}+ \frac{2}{27}$

=$\frac{16}{27} -\frac{16}{27}-\frac{4}{27}+\frac{2}{27}+\frac{2}{27}$

=$\frac{4}{27}-\frac{4}{27}$

= 0

Therefore, the remainder is 0

问题9.如果多项式^{2x 3} + ax 2 + 3x-5和x ^{3 + x 2} -4x + a在除以x-2时留下相同的提示，请找到a的值。

解决方案：

Given:f(x)=2x³+ax²+3x-5,p(x)=x³+x²-4x+a

The remainder are f(2) and p(2) when f(x) and p(x) are divided by x-2

We know that,

f(2) = p(2) (given in problem)

we need to calculate f(2) and p(2)

for, f(2)

substitute (x=2) in f(x)

f(2)=2(2)³+a(2)²+3(2)-5

= 16+4a+1

= 4a+17 ———- 1

for, p(2)

Substitute (x=2) in p(x)

p(2)=2³+2²-4(2)+a

= 8+4-8+a

= 4+a ———– 2

Since, f(2) = p(2)

Equate eq1 and eq2

⇒ 4a+17 = 4+a

⇒ 3a = -13

⇒ a = -13/3

The value of a = -13/3

问题10.如果将多项式³ + 3x ² -3和2x ³ -5x + a除以(x-4)，则将提醒分别设为R1和R2。在以下每种情况下，找到a的值，如果

1. R1 = R2

2. R1 + R2 = 0

3. 2R1-R2 = 0

解决方案：

The polynomials are f(x)=ax³+3x²-3,p(x)=2x³-5x+a

let,

R1 is the reminder when f(x) is divided by x-4

⇒ R1=f(4)

⇒ R1=a(4)³ + 3(4)² -3

= 64a + 48 – 3

= 64a + 45 —————– 1

Now, let

R2 is the reminder when p(x) is divided by x-4

⇒ R2=p(4)

⇒ R2=2(4)³-5(4)+a

= 128-20+a

= 108 +a ——————— 2

1. Given, R1 = R2

⇒ 64a + 45 = 108 +a

⇒ 63a=63

⇒ a =1

2. Given, R1+R2 =0

⇒ 64a + 45 + 108 +a = 0

⇒ 65a + 153 = 0

⇒ a = -153/65

3. Given, 2R1-R2 =0

⇒2( 64a + 45)- (108 +a) =0

⇒ 128a + 90 – 108 -a =0

⇒ 127a – 18 =0

⇒ a =$\frac{18}{127}$

问题11。如果将多项式^{ax 3} + 3x ² -13和2x ³ -5x + a除以(x-2)，则保持相同的提示，请找到a的值。

解决方案：

Given:f(x)=ax³+3x²-13,p(x)=2x³-5x+a

Equate x-2 to zero

⇒ x=2

Substitute the value of x in f(x) and p(x)

f(2)=a(2)³+3(2)²-13

= 8a+12-13

= 8a-1 ————– 1

p(2)=2(2)³-5(2)+a

= 16-10+a

= 6 + a ————- 2

f(2) = p(2)

⇒ 8a-1 = 6+a

⇒ 7a = 7

⇒ a =1

The value of a is 1

问题12。当f(x)=(x) ³ +3(x) ² +3(x)+1除以时，找到提示

1. x + 1

2. x – 1/2

3. x

4. x +π

5. 5 + 2倍

解决方案：

Given:f(x)=x³+3x²+3x+1

by reminder theorem

1. x+1 = 0

x=-1

Substitute the value of x in f(x)

f(-1)=(-1)³+3(-1)²+3(-1)+1

= -1+3-3+1

=0

2. x-1/2 =0

x = 1/2

Substitute the value of x in f(x)

$f(\frac12)=(\frac12)^3+3(\frac12)^2+3(\frac12)+1$

=$\frac18+3(\frac12)^2+3(\frac12)+1$

=$\frac{1+6+12+8}{8}$

=$\frac{27}8$

3. x = 0

Substitute the value of x in f(x)

f(0)=(0)³+3(0)²+3(0)+1

= 0 + 0+0+1

= 1

4. x+π =0

x = -π

Substitute the value of x in f(x)

f(-π)=(-π)³+3(-π)²+3(-π)+1

=-π³+3π²-3π +1

5. 5+2x =0

x = -5/2

Substitute the value of x in f(x)

$f(-\frac52 )=(-\frac52 )^3+3(-\frac52 )^2+3(-\frac52 )+1$

=$\frac{-125}{8}+3\frac{25}{4}+3\frac{-5}{2}+1$

Taking L.C.M

=$\frac{-125+150-50+8}{8}$

=$\frac{-27}{8}$