9类RD Sharma解决方案–第六章多项式的因式分解-练习6.4 |套装2

问题13：如果x – 3是k的因数，则求出k的值k ² x ³ – kx ² + 3kx – k

解决方案：

Let, f(x) = k²x³ – kx² + 3kx – k

According to factor theorem 

If x – 3 is the factor of f(x) then f(3) = 0

⇒ x – 3 = 0

⇒ x = 3

On substituting the value of x in f(x), we get

f(3) = k²(3)³ – k(3)²+ 3k(3) – k

= 27k² – 9k + 9k – k

= 27k² – k

= k( 27k – 1)

Equate f(3) to zero, to find k 

⇒ f(3) = 0

 ⇒ k(27k – 1) = 0

 ⇒ k = 0 and 27k – 1 = 0 

 ⇒ k = 0 and k = 1/27

When k = 0 and 1/27, (x – 3) will be the factor of f(x) 

^{问题14：如果x 2} – 4是f(x)= ax ⁴ + 2x ³ – 3x ² + bx – 4的因数，则求出a和b的值

解决方案：

Given: f(x) = ax⁴ + 2x³ – 3x² + bx – 4, g(x) = x² – 4

We need to find the factors of g(x)

⇒ x² – 4 = 0

⇒ x² = 4

⇒ x = √4

⇒ x = ±2

(x – 2) and (x + 2) are the factors 

According to factor theorem 

If (x – 2) and (x + 2) are the factors of f(x) 

the result of f(2) and f(-2) should be zero 

Let, x – 2 = 0 

⇒ x = 2

On substituting the value of x in f(x), we get

f(2) = a(2)⁴ + 2(2)³ – 3(2)² + b(2) – 4

= 16a  + 2(8) – 3(4) + 2b – 4

= 16a + 2b + 16 – 12 – 4

= 16a + 2b

Equate the value of f(2) to zero 

⇒ 16a + 2b = 0 

⇒ 2(8a + b) = 0

⇒ 8a + b = 0         -(1)

Let, x + 2 = 0 

x = -2

On substituting the value of x in f(x), we get

f(-2) = a(-2)⁴ + 2(-2)³– 3(-2)² + b(-2) – 4

= 16a + 2(-8) – 3(4) – 2b – 4

= 16a – 16 – 12 – 2b – 4

= 16a – 2b – 32 

Equate the value of f(-2) to zero

⇒ 16a – 2b – 32 = 0 

⇒ 16a – 2b – 32 = 0 

⇒ 8a – b  = 16         -(2)

On solving equation (1) and (2) 

8a + b = 0

8a – b = 16

16a = 16

a = 1

On substituting the value of a in eq (1), we get

8(1) + b = 0

b = -8

The values are a = 1 and b = -8

问题15.查找$\alpha, \beta$如果(x + 1)和(x + 2)是以下因素$x^3+3x^2-2\alpha x+\beta$

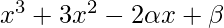
解决方案：

Given: $f(x)=x^3+3x^2-2\alpha x+\beta$ and factors are (x + 1) and (x + 2)

According to factor theorem, 

If they are the factors of f(x) then results of f(-2) and f(-1) should be zero.

Let,

⇒ x + 1 = 0

⇒ x = -1

On substituting the value of x in f(x), we get

$f(x)=x^3+3x^2-2\alpha x+\beta$

=$(-1)^3+3(-1)^2-2\alpha (-1)+\beta$

=$-1+3+2\alpha +\beta$

= 2\alpha +\beta +2         -(1)

Let,

⇒ x + 2 = 0

⇒ x = -2

On substituting the value of x in f(x), we get

$f(x)=x^3+3x^2-2\alpha x+\beta$

$=(-2)^3+3(-2)^2-2\alpha (-2)+\beta$ 

=$-8+12+4\alpha +\beta$

$=4\alpha +\beta +4$       -(2)

On solving eq(1) and (2) 

⇒ $2\alpha +\beta+2 -(4\alpha +\beta +4)=0$

⇒$-2\alpha -2 =0$

⇒$2\alpha=-2$

⇒ $\alpha =-1$

On substituting $\alpha =-1$in equation(1)

⇒$2(-1)+\beta=-2$

⇒$\beta=-2+2$

⇒$\beta=0$

The values are $\alpha =-1$ and $\beta=0$

问题16：找到p和q的值，以便x ⁴ + px ³ + 2x ² – 3x + q被x ² – 1整除

解决方案：

Given: f(x) = x⁴ + px³ + 2x² – 3x + q, g(x) = x² – 1

First, we need to find the factors of x² – 1 

⇒ x² – 1 = 0

⇒ x² = 1

⇒  x = ±1

⇒ (x + 1) and (x – 1)

According to factor theorem

If x = 1, -1 are the factors of f(x) then f(1) = 0 and f(-1) = 0

Let, us take x + 1 = 0

x = -1

On substituting the value of x in f(x), we get

f(-1) = (-1)⁴ + p(-1)³ + 2(-1)² – 3(-1) + q

= 1 – p + 2 + 3 + q

= -p + q + 6         -(1)

Let us take, x – 1 = 0 

x = 1

On substituting the value of x in f(x), we get

f(1) = (1)⁴ + p(1)³ + 2(1)² – 3(1) + q

= 1 + p + 2 – 3 + q

= p + q         -(2)

On solving eq(1) and (2), we get 

-p + q = -6

p + q = 0

2q = -6

q = -3

On substituting q value in eq(2), we get

p + q = 0

p – 3 = 0

p = 3

The value of p = 3 and q = -3

问题17。找到a和b的值，使得(x + 1)和(x – 1)是x ⁴ + ax ³ – 3x ² + 2x + b的因数

解决方案：

Given: f(x) = x⁴ + ax³ – 3x² + 2x + b

The factors are (x + 1) and (x – 1)

According to factor theorem

If x = 1, -1 are the factors of f(x) then f(1) = 0 and f(-1) = 0

Let, us take x + 1

⇒ x + 1 = 0

 ⇒ x = -1

On substituting the value of x in f(x), we get

f(-1) = (-1)⁴ + a(-1)³ – 3(-1)² + 2(-1) + b

= 1 – a – 3 – 2 + b

= -a + b – 4         -(1)

Let, us take x – 1

 ⇒ x – 1 = 0

⇒ x = 1

On substituting the value of x in f(x), we get

f(1) = (1)⁴ + a(1)³ – 3(1)² + 2(1) + b

= 1 + a – 3 + 2 + b

= a + b         -(2)

On solving equation (1) and (2) 

-a + b = 4

a + b = 0

2b = 4

b = 2

On substituting value of b in eq (2), we get

a + 2 = 0

a = -2

The values are a = -2 and b = 2

问题18.如果x ³ + ax ² – bx + 10可被x ³ – 3x + 2整除，则求出a和b的值

解决方案：

Given: f(x) = x³ + ax² – bx + 10, g(x) = x³ – 3x + 2   

First we need to find the factors of g(x)

g(x) = x³ – 3x + 2

=x³ – 2x – x + 2

= x(x – 2) – 1(x – 2)

= (x – 1)(x – 2) are the factors

Let us take (x – 1)

⇒ x – 1 = 0

⇒ x = 1

On substituting the value of x in f(x), we get

f(1) = 1³ + a(1)² – b(1) + 10 

= 1 + a – b + 10

= a – b +11         -(1)

Let us take (x – 2)

⇒ x – 2 = 0

⇒ x = 2

On substituting the value of x in f(x), we get

f(2) = 2³ + a(2)² – b(2) + 10 

= 8 + 4a – 2b + 10

= 4a – 2b + 18

Equating f(2) to zero 

⇒  4a – 2b +18 = 0 

⇒ 2a – b + 9 = 0         -(2)

On solving eq(1) and (2), we get 

a – b = -11

2a – b = -9

a = 2

On substituting the value of a in equation (1), we get 

⇒ 2 – b = – 11

⇒ -b = -11 – 2

⇒ b = 13

The value is a = 2 and b = 13

问题19.如果(x +1)和(x – 1)都是ax ³ + x ² – 2x + b的因数，则求出a和b的值

解决方案：

Given: f(x) = ax³ + x² – 2x + b, (x + 1) and (x – 1) are the factors 

According to factor theorem, 

If x = -1 & 1 are factors of f(x) then f(1) = 0 and f(-1) = 0

Let, x – 1 = 0

⇒ x = 1

On substituting the value of x in f(x), we get

f(1) = a(1)³ + (1)² – 2(1) + b

= a +1 – 2 + b

= a + b – 1         -(1)

Let, x + 1 = 0

⇒ x = -1

On substituting the value of x in f(x), we get

f(-1) = a(-1)³ + (-1)² – 2(-1) + b

= -a + 1 + 2 + b

= -a + b + 3         -(2)

On solving equation (1) and (2), we get 

⇒ a + b = 1

⇒ -a + b = -3

⇒ 2b = -2

⇒ b = -1

On substituting the b in eq (1) 

⇒ a – 1 = 1 

⇒ a = 2

The values are a = 2 and b = -1

问题20.必须将什么加到x ³ – 3x ² – 12x + 19上，以便结果可以被x ² + x – 6整除

解决方案：

Given: p(x) = x³ – 3x² – 12x + 19, g(x) = x² + x – 6

According to division algorithm when p(x) is divided by g(x), 

the remainder will be the linear expression in x 

Let, r(x) = ax + b is added to p(x)

⇒ f(x) = p(x) + r(x)

= f(x) = x³ – 3x² – 12x + 19 + ax + b

We know that, g(x) = x² + x – 6

First, we find the factors of g(x)

⇒ g(x) = x²  + x – 6

= x² + 3x – 2x – 6

= x(x + 3) – 2(x + 3)

= (x – 2)(x + 3)

According to factor theorem

If (x – 2) & (x + 3) are factors of f(x) then f(-3) = 0 and f(2) = 0

Let, x + 3 = 0

⇒ x = -3

On substituting the value of x in f(x), we get

f(-3) = (-3)³ – 3(-3)² – 12(-3) + 19 + a(-3) + b

= -27 – 27 – 3a + 24 + 19 + b

= -3a + b +1         -(1)

Let, x – 2 = 0

⇒ x = 2 

On substituting the value of x in f(x), we get

f(2) = (2)³ – 3(2)² – 12(2) + 19 + a(2) + b

= 8 – 12 + 2a – 24 + b

= 2a + b – 9         -(2)

On solving eq(1) and eq (2), we get

⇒ -3a + b = -1

⇒ 2a + b = 9

⇒ -5a = -10

⇒ a = 2

On substituting the value of a in eq(1) 

⇒ -3(2) + b = -1

⇒ -6 + b = -1

⇒ b = 5

Therefore, r(x) = ax + b

= 2x + 5

Hence, x³ – 3x² – 12x + 19 is divisible by x² + x – 6 when it is added by 2x + 5.

问题21.必须将什么加到x ³ – 6x ² – 15x + 80上，以便结果可以被x ² + x – 12整除

解决方案：

Let p(x) = x³ – 6x² – 15x + 80, q(x) = x² + x – 12

According to algorithm, when p(x) is divided by q(x) the remainder is a linear expression in x. 

So, let r(x) = ax + b is subtracted from p(x), so that p(x) – q(x) is divisible by q(x)

Let, f(x) = p(x) – q(x) 

q(x) = x² + x – 12

= x² + 4x – 3x – 12

= x(x + 4) – 3(x + 4)

=(x – 3)(x + 4)

Clearly, (x – 3) and (x + 4) are factors of q(x)

So, f(x) is divisible by q(x) if (x – 3) and (x + 4) are factors of q(x)

According to factor theorem

f(-4) = 0 and f(3) = 0

⇒ f(3) = 3³ – 6(3)² – 3(a + 15) + 80 – b = 0

= 27 – 54 – 3a – 45 + 80 – b

= -3a – b + 8         -(1)

Similarly, 

f(-4) = 0

 f(-4) = (-4)³ – 6(-4)² – 4(a + 15) + 80 – b 

⇒ -64 – 96 – 4a + 60 + 80 – b = 0 

⇒ 4a – b – 20 = 0

On subtracting eq (1) and eq (2), we get 

4a – b – 20 = 0         -(2) 

⇒ 7a – 28 = 0

⇒ a = 28/7

⇒ a = 4 

On Putting a = 4 in eq (1), we get 

⇒ -3(4) – b = -8

⇒ -b – 12 = -8

⇒ -b = -8 + 12

⇒ b = -4

On substituting a and b values in r(x)

⇒ r(x) = ax + b

⇒ 4x – 4

Hence, p(x) is divisible by q(x), if r(x) = 4x – 4 is subtracted from it.

问题22.必须加3x ³ + x ² – 22x + 9的值，以便结果可以被3x ² + 7x – 6整除

解决方案：

Let, p(x) = 3x³+ x²– 22x + 9 and q(x) = 3x² + 7x – 6

According to divisible theorem, when p(x) is divided by q(x), the reminder is linear equation in x.

Let, r(x) = ax + b is added to p(x), so that p(x) + r(x) is divisible by q(x)

f(x) = p(x) + r(x)

⇒ f(x) = 3x³ + x² – 22x + 9(ax + b)

= 3x³ + x²+ x(a – 22) + b + 9

 We know that,

q(x) = 3x² + 7x – 6

= 3x² + 9x – 2x – 6

= 3x(x + 3) – 2(x + 3)

= (3x – 2)(x + 3)

So, f(x) is divisible by q(x) if (3x – 2) and (x + 3) are the factors of f(x)

From factor theorem 

f(2/3) = 0 and f(-3) = 0

Let, 3x – 2 = 0

3x = 2

x = 2/3

$f(\frac23)=3(\frac23)^3+(\frac23)^2+\frac23(a-22)+b+9$

= $3(\frac8{27})+\frac49+\frac23a-\frac{44}3+b+9$

= $\frac{12}9+\frac23a-\frac{44}3+b+9$

= $\frac{12+6a-132+9b+81}{9}$

Equate to zero 

⇒ $\frac{12+6a-132+9b+81}{9}=0$

⇒ 6a + 9b – 39 = 0

⇒ 2a + 3b – 13 = 0         -(1)

Similarly, 

Let, x + 3 = 0

⇒ x = -3

f(-3) = 3(-3)³ + (-3)² – 3(a – 22) + b + 9

= -81 + 9 – 3a + 66 + b + 9

= -3a + b + 3

Equate to zero

⇒ -3a + b + 3 = 0 

Multiply the given equation by 3 

⇒ -9a + 3b + 9 = 0         -(2)

On Subtracting eq (1) from eq(2) 

⇒ -9a + 3b + 9 – 2a – 3b + 13 = 0

⇒-11a + 22 = 0

⇒-11a = -22

⇒ a = 2

On substituting value of a in eq (1) 

⇒ -3(2) + b = -3

⇒ -6 + b = -3

⇒ b = 3

Put the values in r(x) 

r(x) = ax + b

= 2x + 3

Hence, p(x) is divisible by q(x), if r(x) = 2x + 3 is added to it. 

问题23.如果x – 2是以下两个多项式中每一个的因数，则分别找到a的值：

(i)x ³ – 2ax ² + ax – 1

(ii)x ⁵ – 3x ⁴ –斧³ + 3ax ² + 2ax + 4

解决方案：

(i) Let f(x) = x³ – 2ax² + ax – 1

According to factor theorem

If (x-2) is a factor of f(x) then f(2) = 0

Let, x – 2 = 0

⇒ x = 2

On substituting the value of x in f(x), we get

f(2) = 2³ – 2a(2)² + a(2) – 1  

= 8 – 8a + 2a – 1

= -6a + 7

Equate f(2) to zero 

 ⇒ -6a + 7 = 0

⇒ -6a = -7

⇒ a = 7/6

So, (x – 2) is the factor of f(x)

(ii) Let f(x) = x⁵ – 3x⁴ – ax³ + 3ax² + 2ax + 4

According to factor theorem

If (x – 2) is a factor of f(x) then f(2) = 0

Let, x – 2 = 0

⇒ x = 2 

On substituting the value of x in f(x), we get

f(2) = 2⁵ – 3(2)⁴ – a(2)³ + 3a(2)² + 2a(2) + 4

= 32 – 48 – 8a + 12 + 4a + 4

= 8a -12

Equate f(2) to zero

⇒ 8a – 12 = 0

⇒ a = 12/8

⇒ a = 3/2

So, (x – 2) is a factor of f(x) 

问题24.在以下两个多项式的每一个中，如果(x – a)是一个因数，则找到a的值：

(i)x ⁶ –斧⁵ + x ⁴ –斧³ + 3x – a + 2

(ii)x ⁵ – a ² x ³ + 2x + a + 1

解决方案：

(i) Let, f(x) = x⁶ – ax⁵ + x⁴ – ax³ + 3x – a + 2

Here, x – a = 0

⇒ x = a

On substituting the value of x in f(x), we get

f(a) = a⁶ – a(a)⁵ + (a)⁴ – a(a)³ + 3(a) – a + 2

= a⁶ – a⁶ + a⁴ – a⁴ + 3a – a + 2  

= 2a+2

Equate to zero

⇒ 2a + 2 = 0

⇒ 2(a + 1) = 0

⇒ a = -1

So, (x – a) is a factor of f(x)

(ii) Let, f(x) = x⁵ – a²x³ + 2x + a + 1

Here, x – a = 0

⇒ x = a

On substituting the value of x in f(x), we get

f(a) = a⁵ – a²(a)³ + 2(a) + a + 1

= a⁵ – a⁵ + 2a + a + 1  

= 3a + 1

Equate to zero

⇒ 3a + 1 = 0

⇒ 3a = -1

⇒ a = -1/3

So, (x – a) is a factor of f(x)

问题25.在以下两个多项式的每一个中，如果(x + a)是一个因数，则找到a的值：

(i)x ³ + ax ² – 2x + a +4

(ii)x ⁴ – a ² x ² + 3x – a

解决方案：

(i) Let, f(x) = x³ + ax² – 2x + a + 4

Here, x + a = 0

⇒ x = – a

On substituting the value of x in f(x), we get

f(-a) = (-a)³+ a(-a)²– 2(-a) + a + 4

= 3a + 4

Equate to zero

⇒ 3a + 4 = 0

⇒ 3a = -4

⇒ a = -4/3

So, (x + a) is a factor of f(x) 

(ii) Let, f(x) = x⁴– a²x² + 3x – a

Here, x + a = 0

⇒ x = -a

On substituting the value of x in f(x), we get

f(-a) = (-a)⁴ – a²(-a)² + 3(-a) – a

= a⁴ – a⁴ – 3a – a

= -4a

Equate to zero

⇒ -4a = 0

⇒ a = 0

So, (x + a) is a factor of f(x)