第9章平行四边形和三角形的区域–练习9.3第1套
问题11。在图中,ABCDE是五边形。穿过B且与AC平行的线与在F处产生的DC相交。
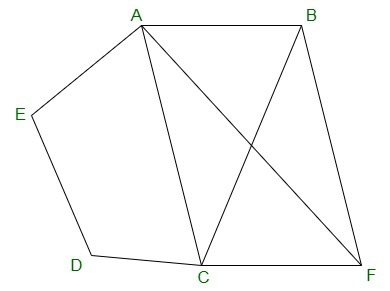
显示
(i)ar(ΔACB)= ar(ΔACF)
(ii)ar(AEDF)= ar(ABCDE)
解决方案:
(i) ΔACB and ΔACF lie on the same base AC and between the same parallels AC and BF.
Therefore,
ar(ΔACB) = ar(ΔACF)
(ii) ar(ΔACB) = ar(ΔACF)
=> ar(ΔACB)+ar(ΔACDE) = ar(ΔACF)+ar(ΔACDE)
=> ar(ABCDE) = ar(AEDF)
问题12:一个村民伊特瓦里(Itwaari)拥有一块四边形的土地。该村的Gram Panchayat决定从一个角落接管他的部分土地,以建造一个保健中心。伊塔瓦里同意上述提议,但条件是应给他相等数量的土地,以代替他毗邻其土地的土地,从而形成一个三角形的土地。解释该建议将如何实施。
解决方案:

Let us assume ABCD be the plot of the land of the shape of a quadrilateral.
Construction:
Join the diagonal BD.
Draw AE parallel to BD.
Join BE, that intersected AD at O.

We will get,
ΔBCE is the shape of Itwari’s original field
ΔAOB is the area for construction of the Health Centre.
ΔDEO is the land joined to the plot.
To prove:
ar(ΔDEO) = ar(ΔAOB)
Proof:
ΔDEB and ΔDAB lie on the same base BD, in-between the same parallels BD and AE.
Therefore,
Ar(ΔDEB) = ar(ΔDAB)
=> ar(ΔDEB) – ar(ΔDOB) = ar(ΔDAB) – ar(ΔDOB)
=> ar(ΔDEO) = ar(ΔAOB)
问题13. ABCD是AB ||的梯形。直流电平行于AC的线在X处的AB与Y处的BC相交。证明ar(ΔADX)= ar(ΔACY)。
[提示:加入CX。]
解决方案:

Given:
ABCD is a trapezium with,
AB || DC.
XY || AC
To Construct,
Join CX
Prove:
ar(ADX) = ar(ACY)
Proof:
ar(ΔADX) = ar(ΔAXC) -(equation 1)(Since they are on the same base AX and
in-between the same parallels AB and CD)
also,
ar(ΔAXC)=ar(ΔACY) -(equation-2)(Since they are on the same base AC and
in-between the same parallels XY and AC)
From equation (1) and (2),
ar(ΔADX) = ar(ΔACY)
问题14.在图AP中|| BQ || CR。证明ar(ΔAQC)= ar(ΔPBR)。
解决方案:

Given:
AP || BQ || CR
Prove:
ar(AQC) = ar(PBR)
Proof:
ar(ΔAQB) = ar(ΔPBQ) -(equation 1)(Since they are on the same base BQ and
between the same parallels AP and BQ)
also,
ar(ΔBQC) = ar(ΔBQR) (equation 2)(Since they are on the same base BQ and
between the same parallels BQ and CR)
Adding equations (1) and (2),
ar(ΔAQB)+ar(ΔBQC) = ar(ΔPBQ)+ar(ΔBQR)
=> ar(ΔAQC) = ar(ΔPBR)
问题15:四边形ABCD的对角AC和BD在O处相交,使得ar(△AOD)= ar(△BOC)。证明ABCD是梯形。
解决方案:

Given:
ar(ΔAOD) = ar(ΔBOC)
Prove:
ABCD is a trapezium.
Proof:
ar(ΔAOD) = ar(ΔBOC)
=> ar(ΔAOD) + ar(ΔAOB) = ar(ΔBOC)+ar(ΔAOB)
=> ar(ΔADB) = ar(ΔACB)
Areas of ΔADB and ΔACB are equal.
Therefore,
They should be lying between the same parallel lines.
Therefore,
AB ∥ CD
Hence, ABCD is a trapezium.
问题16。在图中,ar(DRC)= ar(DPC)和ar(BDP)= ar(ARC)。证明四边形ABCD和DCPR都是梯形。

解决方案:
Given:
ar(ΔDRC) = ar(ΔDPC)
ar(ΔBDP) = ar(ΔARC)
Prove:
ABCD and DCPR are trapeziums.
Proof:
ar(ΔBDP) = ar(ΔARC)
⇒ ar(ΔBDP) – ar(ΔDPC) = ar(ΔDRC)
⇒ ar(ΔBDC) = ar(ΔADC)
ar(ΔBDC) = ar(ΔADC).
Therefore,
ar(ΔBDC) and ar(ΔADC) are lying in-between the same parallel lines.
Hence, AB ∥ CD
ABCD is a trapezium.
Similarly,
ar(ΔDRC) = ar(ΔDPC).
Therefore,
ar(ΔDRC) and ar(ΔDPC) are lying in-between the same parallel lines.
Hence, DC ∥ PR
Thus, DCPR is a trapezium.