切线和法线方程

导数用于求函数相对于变量的变化率。要找到函数相对于变量的变化率，需要将函数相对于该变量进行微分。函数y = f(x)相对于 x 的变化率由 dy/dx 或 f'(x) 定义。例如 y = x ² + x 那么，dy/dx = f'(x) = d(x ² + x)/dx = 2x + 1。

当两个变量（让 x = f(t) 和 y = g(t)）相对于另一个变量 t 变化时，变化率由链式法则计算。 y 相对于 x 的变化率为：dy/dx = (dy/dt) × (dt/dx)。例如，x = t 和 y = 2t ，则 dy/dx 计算为：dx/dt = 1 或 dt/dx = 1 和 dy/dt = 2。因此，dy/dx = (dy/dt) × ( dt/dx) = 2 × 1 = 2。因此，y 相对于 x 的变化率计算为 y 相对于 t 的变化率和 t 相对于 x 的变化率。

衍生品的应用

导数主要用于数学中，以找出任何变量的值相对于另一个变量的变化。在现实生活中，也会看到和讨论衍生品。比如速度这个词被提到很多，但很少有人知道速度也是一个导数，速度是距离相对于时间的变化。让我们看看衍生品的一些重要应用，

• 求函数相对于变量的变化率。
• 找到切线和法线曲线。
• 求函数的最大值和最小值。
• 查找函数是增加还是减少以及函数增加或减少的区间。
• 求函数的凹度（函数是上凹还是下凹）。
• 从位移计算速度，从速度计算加速度（速度是位移相对于时间的变化，加速度是速度相对于时间的变化）。
• 找出某些数量的近似值（例如平方根、一个数字的立方根）。

切线和法线

在给定点与曲线相切是在给定点接触曲线的直线（不与曲线相交，仅在给定点接触曲线）。而法线是垂直于切线的直线。

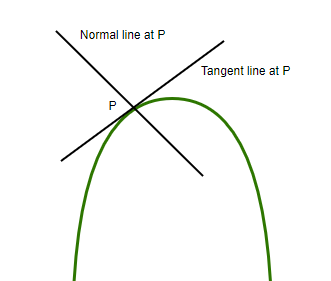
因此，切线斜率和法线斜率的乘积为-1 。令切线和法线的表示斜率为m _T 和m _N 分别。然后，

m_T × m_N = -1.

If tangent makes angle Θ with x-axis then slope of tangent = m_T = tan Θ.

Since, m_T × m_N = -1 So, tan Θ × m_N = -1. 

Hence, the slope of normal is -1/tan Θ or -cot Θ.

正切方程和法线方程

方程为y = f(x)在点a的曲线的切线斜率为f'(a) （ f(x)在点a 的导数）。因此，点斜率形式的切线方程为

(y – f(a))/(x – a) = f'(a), 

并使用方程m _T × m _N = -1 ，

法线方程为：

(y – f(a))/(x – a) = -1/f'(a).

示例问题

问题1：y = x ²是一个曲线方程，求切线方程，法线在点(1, 1)。

解决方案：

First differentiate y = x², y’ = 2x .

Slope of tangent at point (1, 1) is 2 × 1 = 2 and slope of normal is -1/2 .

Hence, equation of tangent at point (1, 1) is: (y – 1)/(x – 1) = 2 => y = 2x – 1

And equation of normal at point (1, 1) is: (y – 1)/(x – 1) = -1/2 = 2y = -x + 3 .

问题2：y = x ⁴ + x ²是一个曲线方程，求点(1, 2)的切线方程和法线方程

解决方案：

First differentiate y = x⁴ + x² , we get y’ = 4x³ + 2x .

Slope of tangent at point (1, 2) is 4 × (1)³ + 2 × 1 = 6 and slope of normal is -1/6.

Hence, equation of tangent at point (1, 2) is: (y – 2)/(x – 1) = 6 => y = 6x – 4

And equation of normal at point (1, 2) is: (y – 2)/(x – 1) = -1/6 => 6y = -x + 13.

问题3：y = x 是曲线方程，求(1, 1) 点的切线方程和法线方程。

解决方案：

First differentiate y = x , we get y’ = 1 .

The slope of the tangent at point (1, 1) is 1, and the slope of normal is -1.

Hence, equation of tangent at point (1, 1) is: (y – 1)/(x – 1) = 1 => y = x

And equation of normal at point (1, 1) is: (y – 1)/(x – 1) = -1 => 2y = -x + 2.

问题 4：x = t 和 y = t 是曲线方程，求点 t = 2 处的切线方程和法线方程。

解决方案：

First differentiate x = t, we get dx/dt = 1 or dt/dx = 1.

And differentiate y = t, dy/dt = 1.

Hence, dy/dx = (dy/dt) × (dt/dx) = 1 × 1 = 1.

The slope of the tangent at point t = 2 is 1 and slope of normal is -1.

Hence, equation of tangent at point at point t = 2 is: (y – 2)/(x – 2) = 1 => y = x

And equation of normal at point at point t = 2 is (y – 2)/(x – 2) = -1 => y = -x + 4.

问题 5：x = t 和 y = t ²是曲线方程，求 t = 1 处的切线方程和法线方程。

解决方案：

First, differentiate x = t, dx/dt = 1 or dt/dx = 1.

And differentiate y = t², dy/dt = 2t = 2 × 1 = 2.

Hence, dy/dx = (dy/dt) × (dt/dx) = 2 × 1 = 2.

The slope of the tangent at point t = 1 is 2 and the slope of normal is -1/2.

Hence, equation of tangent at point at point t = 1 is (y – 1)/(x – 1) = 2 => y = 2x – 1.

And equation of normal at point at point t = 1 is (y – 1)/(x – 1) = -1/2 => 2y = -x + 3.