10类RD Sharma解决方案–第16章表面积和体积–练习16.3 |套装2

问题11.桶的形式为圆锥台形，其圆锥台的高度为30厘米，其下端和上端半径分别为10厘米和20厘米。找到铲斗的容量和表面积。另外，找到可以完全装满容器的牛奶的成本，为每升25卢比。

解决方案：

Let R and r be the radii of the top and base of the bucket respectively,

Let h be its height.

Then, we have R = 20 cm, r = 10 cm, h = 30 cm

Capacity of the bucket = Volume of the frustum of the cone

= 1/3 π(R² + r² + R r )h

= 1/3 π(20² + 10² + 20 x 10 ) x 30

= 3.14 x 10 (400 + 100 + 200)

= 21980 cm³ = 21.98 litres

Now,

Surface area of the bucket = CSA of the bucket + Surface area of the bottom

= π l (R + r) + πr²

We know that,

l = √h² + (R – r)²

= √[30² + (20 – 10)²] = √(900 + 100)

= √1000 = 31.62 cm

So,

The Surface area of the bucket = (3.14) x 31.62 x (20 + 10) + (3.14) x 10²

= 2978.60 + 314

= 3292.60 cm²

Next, given that the cost of 1 litre milk = Rs 25

Thus, the cost of 21.98 litres of milk = Rs (25 x 21.98) = Rs 549.50

问题12：圆锥体的实心平截头体的圆形末端的半径分别为33 cm和27 cm，其倾斜高度为10 cm。查找其总表面积。

解决方案：

Given:

Radii of top circular ends (r₁) = 20 cm

Radii of bottom circular end of bucket (r₂) = 12 cm

Let height of bucket be ‘h’.

Volume of frustum cone = 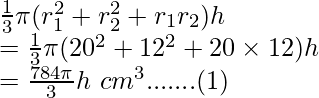$\frac{1}{3}\pi(r_1^2+r_2^2+r_1r_2)h\\ =\frac{1}{3}\pi(20^2+12^2+20\times12)h\\ =\frac{784\pi}{3}h\ cm^3.......(1)$

Given capacity/ volume of bucket = 12308.8 cm³      —–(2)

Equating (1) & (2)

$\Rightarrow\frac{784\pi}{3}h=12308.8\\ \Rightarrow h=\frac{12308.8\times3}{784\times\pi}$

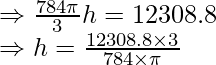
⇒ h = 15 cm

Therefore, 

Height of bucket (h) = 15 cm

Let ‘l’ be slant height of bucket

⇒  l² = (r₁ – r₂)² + h²

⇒ l = $\sqrt{(r_1-r_2)^2+h^2}$

⇒ l = $\sqrt{(20-12)^2+15^2}=\sqrt{64+225}$

⇒ l = 17 cm

Therefore, 

Length of bucket/ slant height of bucket (l) = 17 cm

Curved surface area of bucket = π(r₁ + r₂)l + πr₂²

= π(20 + 12)17 + π(12)²

= π(32)17 + π(12)²

= π(9248 + 144) = 2160.32 cm²

Therefore,

Curved surface area = 2160.32 cm²

问题13.用铝片制成的水桶高20厘米，上下两端的半径分别为25厘米和10厘米。找到使铲斗如果铝片收费每100cm ² 70 Rs的成本是多少?

解决方案：

Given:

Height of bucket (h) = 20 cm

Upper radius of bucket (r₁) = 25 cm

Lower radius of bucket (r₂) = 10 cm

Let ‘l’ be slant height of bucket

$l=\sqrt{(r_1-r_2)^2+h^2}\\ l=\sqrt{(25-10)^2+20^2}=\sqrt{225+400}\\ l=25\ cm$

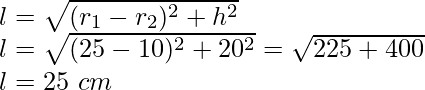
Therefore,

Slant height of bucket (l) = 25 cm

Curved surface area of bucket = π(r₁ + r₂)l + πr₂²

= π(25 + 10)25 + π(10)²

= π(35)25 + π(100) = 975π

Curved surface area = 3061.5 cm²

Cost of making bucket per 100 cm² = Rs 70

Cost of making bucket per 3061.5 cm² = $\frac{3061.5}{100}\times70$

= Rs 2143.05

Therefore,

Total cost for 3061.5 cm² = Rs 2143.05

问题14：圆锥体的实心平截头体的圆形末端的半径分别为33 cm和27 cm，其倾斜高度为10 cm。找到其总表面积?

解决方案：

Given:

Slant height of frustum cone = 10 cm

Radii of circular ends of frustum cone are 33 and 27

r₁ = 33 cm; r₂ = 27 cm

Total surface area of a solid frustum of cone = π(r₁ + r₂) × l + πr₁² + πr₂²

= π(33 + 27) × 10 + π(33)² + π(27)²

= π(60) × 10 + π(33)² + π(27)²

= π(600 + 1089 + 729)

= 2418π cm²

= 7599.42 cm²

Therefore, 

Total surface area of frustum cone = 7599.42 cm²

问题15.用金属板制成的水桶是圆锥形的视锥台，高度为16 cm，其上下两端的直径分别为16 cm和40 cm。找出铲斗的体积，如果所用金属板的成本为每100 cm ² Rs 20，则还要找出铲斗的成本?

解决方案：

Given:

Height of frustum cone = 16 cm

Diameter of lower end of bucket (d₁) = 16 cm

Lower end radius (r₁) = 16/2 = 8 cm

Upper end radius (r₂) = 40/2 = 20 cm

Let ‘l’ be slant height of frustum of cone

$l=\sqrt{(r_1-r_2)^2+h^2}\\ l=\sqrt{(20-8)^2+16}\\ l=\sqrt{144+256}$

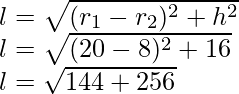
l = 20 cm

Therefore,

Slant height of frustum cone (l) = 20 cm

Volume of frustum cone =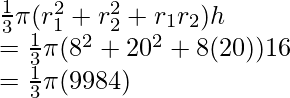 
$\frac{1}{3}\pi(r_1^2+r_2^2+r_1r_2)h\\ =\frac{1}{3}\pi(8^2+20^2+8(20))16\\ =\frac{1}{3}\pi(9984)$

Volume = 10449.92 cm³

Curved surface area of frustum cone = π(r₁ + r₂)l + πr₂²

= π(20 + 8)20 + π(8)²

= π(560 + 64) = 624π cm²

Cost of metal sheet per 100 cm² = Rs 20

Cost of metal sheet for 624π cm² = $\frac{624\pi}{100}\times20$

= Rs 391.90

Therefore,

Total cost of bucket = Rs 391.9

问题16：固体呈圆锥台形。两个圆形端的直径分别为60厘米和36厘米，高度为9厘米。查找其整个表面的面积和体积?

解决方案：

Given:

Height of a frustum cone = 9 cm

Lower end radius (r₁) = 60/2 cm = 30 cm

Upper end radius (r₂) = 36/2 cm = 18 cm

Let slant height of frustum cone be l

$l=\sqrt{(r_1-r_2)^2+h^2}\\ l=\sqrt{(8-30)^2+9^2}\\ l=\sqrt{144+81}\\ l=15\ cm$

Volume of frustum cone =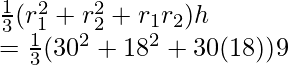 
$\frac{1}{3}π(r_1^2+r_2^2+r_1r_2)h\\ =\frac{1}{3}π(30^2+18^2+30(18))9$

= 5292π cm³

Volume = 5292π cm³

Total surface area of frustum cone = π(r₁ + r₂) × l + πr₁² + πr₂² 

= π(30 + 18)15 + π(30)² + π(18)²

= π(48(15) + (30)² + (18)²)

= π(720 + 900 + 324)

= 1944π cm²

Therfore,

Total surface area = 1944π cm²

问题17：牛奶容器是用圆锥形截头圆锥形的金属板制成的，其容积为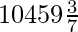$10459\frac{3}{7}$厘米³它的上下圆形端的半径分别为8厘米和20厘米。找出用于制造容器的金属板的成本，成本为每厘米² 1.40卢比吗?

解决方案：

Given: 

Lower end radius of bucket (r₁) = 8 cm

Upper end radius of bucket (r₂) = 20 cm

Let height of bucket be ‘h’

V₁ = $\frac{1}{3}π(8^2+20^2+8(20))h\ cm^3\ \ \ ----(1)$

Volume of milk container = $10459\frac{3}{7}\ cm^3$

V₂ = $\frac{73216}{7}\ cm^3\ \ \ \ -----(2)$ 

Equating (1) and (2)

V₁ = V₂

⇒ $\frac{1}{3}(8^2+20^2+8(20))h=\frac{73216}{7}$

⇒ h = $\frac{10459.42}{653.45}$

⇒ h = 16 cm

Therefore,

Height of frustum cone(h) = 16 cm

Let slant height of frustum cone be ‘l’

l = 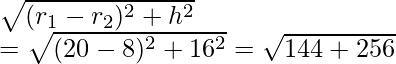$\sqrt{(r_1-r_2)^2+h^2}\\ =\sqrt{(20-8)^2+16^2}=\sqrt{144+256}$

l = 20 cm

Therefore,

Slant height of frustum cone (l) = 20 cm

Total surface area of frustum cone = π(r₁ + r₂)l + πr₁² + πr₂²

= π(20 + 8)20 + π(20)² + π(8)²

= π(560 + 400 + 64)

= π(960 + 64) = 1024π = 3216.99 cm²

Total surface area = 3216.99 cm²