10类RD Sharma解决方案–第16章表面积和体积–练习16.3 |套装1

问题1.铲斗的顶部和底部直径分别为40厘米和20厘米。如果水桶的深度为12厘米，请查找水桶的体积。另外，找到用于制造铲斗的锡板的成本，价格为1.20 Rs / dm ² 。

解决方案：

Given,

Diameter of the top of the bucket = 40 cm

Therefore,

Radius (r₁) = 40/2 = 20 cm

Also,

Diameter of bottom part of the bucket = 20 cm

Therefore,

Radius (r₂) = 30/2 = 10cm

Depth of the bucket (h) = 12 cm

Now, 

Volume of the bucket = 1/3 π(r₂² + r₁² + r₁ r₂)h

= π/3(20² + 10² + 20 × 10)12

= 8800 cm³

Now,

Let us assume L to be the slant height of the bucket. 

$L = \sqrt{(r_2 - r_1)^2 + h^2} \\ = \sqrt{(20-10)^2 + 12^2} \\ = 15.62 cm$

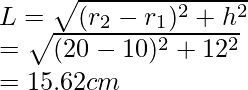
Also,

Total surface area of bucket = 

$\pi(r_1 + r_2) * L + \pi * r_2^2 \\ = \pi (20+10) * 15.62 + \pi 10^2 \\ = \frac{1320\sqrt61 + 2200}{7} \\ = 17.87 dm^2$

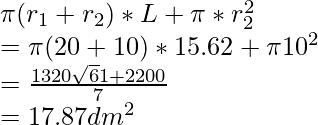
Since,

The cost of tin sheet used for making bucket per dm² = Rs 1.20

Therefore, the total cost for making bucket 17.87dm² = 1.20 × 17.87 = Rs 21.40

问题2.圆锥台的截头圆锥体的底部直径为20厘米，顶部直径为12厘米，高度为3厘米。找到其整个表面的面积和体积。

解决方案：

Given,

We know,

Base diameter of the cone (d₁) = 20 cm

Therefore,

Radius (r₁) = 20/2 cm = 10 cm

Top diameter of Cone (d₂) = 12 cm

Therefore,

Radius (r₂) = 12/2 cm = 6 cm

Also,

Height of the cone (h) = 3 cm

Volume of the frustum of a right circular cone = 1/3 π(r₂² + r₁² + r₁ r₂ )h

Substituting the values,

= π/3(10² + 6² + 10 × 6)3

= 616 cm³

Let us assume ‘L’ to be the slant height of the cone, then we know that

Now, 

L = √(r₁ – r2₁)² + h²

L = √(10 – 6)² + 3²

L = √(16 + 9)

Solving, we get,

L = 5cm

Therefore,

The slant height of cone (L) = 5 cm

Thus,

Total surface area of the frustum = π(r₁ + r₂) x L + π r₁² + π r₂²

Substituting the values,

= π(10 + 6) × 5 + π × 10² + π × 6²

= π(80 + 100 + 36)

= π(216)

= 678.85 cm²

问题3.圆锥台的斜面高度为4厘米，其圆锥形端的周长为18厘米和6厘米。找到平截头体的曲面。

解决方案：

Given,

Slant height of frustum of the cone (l) = 4 cm

Let us assume the ratio of the top and bottom circles be r₁ and r₂ respectively.

And, we know, 

Perimeters of the circular ends are 18 cm and 6 cm respectively.

Substituting the values,

⟹ 2πr₁ = 18 cm and 2πr₂ = 6 cm

Solving, we get,

⟹ πr₁= 9 cm and πr₂ = 3 cm

Since, we know,

Curved surface area of frustum of a cone = π(r₁ + r₂)l

= (πr₁+πr₂)l 

= (9 + 3) × 4 

= (12) × 4 = 48 cm²

Therefore, the curved surface area = 48 cm²

问题4.右圆锥台的平截头体末端的周长是44厘米和33厘米。如果视锥的高度为16厘米，请找到其体积，倾斜表面和整个表面。

解决方案：

Given values,

Perimeter of the upper end of the circular cone= 44 cm

Now,

2 π r₁ = 44

2(22/7) r₁ = 44

Solving, we get,

r₁ = 7 cm

Also,

Perimeter of the lower end of the circular cone= 33 cm

2 π r₂ = 33

2(22/7) r₂ = 33

Solving,

r₂ = 21/4 cm

Now,

Let us assume the slant height of the frustum of a right circular cone to be L

Given,

L = 16.1 cm

$L = \sqrt{(r_1 - r_2)^2 + h^2} \\ = \sqrt{(7-5.25)^2 + 16^2}$

We know, the curved surface area of the frustum cone = π(r₁ + r₂)l

Substituting values,

= π(7 + 5.25)16.1

Curved surface area of the frustum cone = 619.65 cm³

And, The volume of the frustum cone = 1/3 π(r₂² + r₁² + r₁ r₂)h

= 1/3 π(72 + 5.252 + (7) (5.25)) x 16

= 1898.56 cm³

Now, the total surface area of the frustum cone

= π(r₁ + r₂) x L + π r₁² + π r₂²

= π(7 + 5.25) × 16.1 + π7² + π5.25²

= π(7 + 5.25) × 16.1 + π(7² + 5.25²) = 860.27 cm²

Therefore, the total surface area of the frustum cone is 860.27 cm²

问题5.如果一个圆锥形桶的圆形末端(高45厘米)的半径为28厘米和7厘米，请找出桶的容量。

解决方案：

Given,

The height of the conical bucket = 45 cm

Also,

The radii of the two ends of the conical bucket are 28 cm and 7 cm respectively.

Now, x = 28 cm and y = 7 cm

Now, Volume of the conical bucket = 1/3 π(x² + y² + xy )h

Substituting the values, we get,

= 1/3 π(28² + 7² + 28 × 7)45 = 15435π

Therefore, the volume of the bucket is 48510 cm³.

问题6.锥体的高度为20厘米。一个小的圆锥体从顶部被平行于底部的平面切掉。如果其体积为原始圆锥体积的1/125，请确定在底部上方的哪个高度进行切割。

解决方案：

Let the radius of the small cone and big cone be R and r cm respectively.

Given, height of the big cone = 20 cm

Let us assume the height of section made to be h cm

Now, 

Calculating the height of small cone = (20 – h) cm

Considering △OAB and △OCD

∠AOB = ∠COD [common]

And, since both of the angles are 90^o

∠OAB = ∠OCD 

Now, by AA similarity

Then, OAB ~ △OCD

So, by C.P.S.T we have

OA/ OC = AB/ CD

Substituting values,

(20 – h)/ 20 = r/ R …… (i)

And,

Volume of small cone = 1/125 x volume of big cone, that is,

1/3 π r²(20 – h) = 1/125 × 1/3 πR² x 20

=>r_²/ R² = 1/125 × 20/ (20 – h) [From (i)]

=>(20 – h)²/ 20² = 1/125 × 20/20 – h

=>(20 – h)³ = 20³/ 125

=>20 – h = 20/5

=>20 – h = 4

Now,

h = 20 – 4 = 16 cm

Therefore, the section was made at a height of 16 cm above the base.

问题7.如果一个高度为24 cm的桶的圆形端部的半径分别为5和15 cm，请找到桶的表面积。

解决方案：

We know,

Height of the bucket (h) = 24 cm

Radii of the circular ends of the bucket x and y are 5 cm and 15 cm respectively.

Let us assume L to be the slant height of the bucket

$L = \sqrt{(r_1 - r_2)^2 + h^2} \\ = \sqrt{(5 - 15)^2 + 24^2} \\ = \sqrt{100+576} \\ = 26 cm$

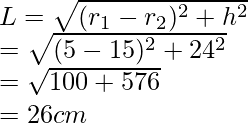
Now,

Curved surface area of the bucket is given by= π(x+ y)l + πx²

Substituting the values, we get,

= π(5 + 15)26 + π5² = π(520 + 25) = 545π cm²

问题8.右圆锥台的平截头体的圆底半径为12 cm和3 cm，高度为12 cm。找到平截头体的总表面积和体积。

解决方案：

Given,

Height of frustum cone = 12 cm

Now,

The radius of the lower end (x) of the frustum of cone= 12 cm

The radius of the upper end (y) of the frustum of cone= 3 cm

Let us assume L to be the slant height of the frustum cone

Now,

$L = \sqrt{(r_1 - r_2)^2 + h^2} \\ = \sqrt{81+144} \\ = 15 cm$

L = 15 cm

Now, 

The total surface area of frustum of a cone = π (x + y) x L + π x² + π y²

Substituting values,

= π (12 + 3)15 + π12² + π3²

Solving, we get,

= 378 π cm²

Also,

Volume of frustum cone = 1/3 π(y² + x² + x y )h

= 1/3 π(122 + 3² + 1² × 3) × 12

 = 756π cm³

问题9.帐篷由一个圆锥台的平截头台组成，圆锥台上盖有一个圆锥体。如果平截头端的半径为13 m和7 m，平截头的高度为8 m，圆锥形帽的倾斜高度为12 m，请找到帐篷所需的帆布。

解决方案：

Given,

Radii of the frustum cone are 13 cm and 7 cm respectively.

Height of the frustum cone, h = 8 m

Now, 

The radius of the lower end (x) of the frustum of cone= 13 cm

The radius of the upper end (y) of the frustum of cone= 7 cm

Let us assume L to be the slant height of the frustum cone

Now, 

$L = \sqrt{(r_1 - r_2)^2 + h^2} \\ = \sqrt{(13 - 7)^2 + 8^2} \\ = \sqrt{36+64} \\ = 10 m$

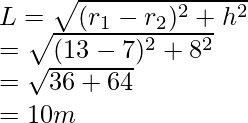
Curved surface area of the frustum (s₁) = π(x + y) × L 

=  π(13 + 7) × 10 

= 200 π m²

Also,

Slant height of conical cap = 12 m

And,

Base radius of upper cap cone = 7 m

Now,

The curved surface area of upper cap cone (s₂) = π *r *l 

= π × 7 × 12 

= 264 m²

Therefore, the total canvas required for tent (S) 

= Curved surface area of the frustum + Curved surface area of upper cap cone 

= s₁ + s₂

S = 200π + 264 

= 892.57 m² which is the canvas required for the tent 

问题10.高度为16厘米的牛奶容器是用圆锥台截头圆锥形的金属板制成的，其下端和上端的半径分别为8厘米和20厘米。以卢比的价格找到牛奶的成本。每升可容纳44升的容器。

解决方案：

Given,

The radius of the lower end (x) of the frustum of cone= 8 cm

The radius of the upper end (y) of the frustum of cone= 20 cm

Height, h = 16 cm

Now,

The capacity of the container = Volume of frustum of the cone

which is equivalent to, 

= 1/3 π(y² + x² + xy )h

Substituting the values,

= 1/3 π(20² + 8² + (20) (8) ) x 16

= 10459.42 cm³ = 10.46 litres

Cost of 1 litre of milk = Rs 44

Cost of 10.46 litres of milk = Rs (44 x 10.46) = Rs 460.24

Therefore, 

The cost of 21.98 litres of milk = Rs (25 x 21.98) = Rs 549.50