10类NCERT解决方案-第9章三角学的某些应用–练习9.1 |套装2

第9章三角学的某些应用–练习9.1 |套装1

问题11：电视塔垂直竖立在运河两岸。从与塔对面的另一排上的一点开始，塔顶的仰角为60°。从连接此点到塔架脚的线上的该点之外的另一个点20 m，塔架顶部的仰角为30°(见图)。找到塔的高度和运河的宽度。

解决方案：

In fig: AB = tower = ?

CB = canal = ?

In rt. ∆ABC,

tan60° = $\frac{AB}{BC}$

h/x = √3

h = √3 x          -(1)

In rt. ∆ABD

$\frac{AB}{BD}$ = tan 30°

$\frac{h}{x + 20}$ = 1/√3

h = (x + 20)/√3          -(2)

From (1) and (2)

√3/1 = (x + 20)/√3  

3x = x + 20

3x – x = 20

2x = 20

X = 20/2

X = 10

Width of the canal is 10m

Putting value of x in equation 1

h = √3 x

= 1.732(10)

= 17.32

Height of the tower 17.32m.

问题12.从一栋7 m高的建筑物的顶部开始，电缆塔顶部的仰角为60°，而其脚下垂的角度为45°。确定塔的高度。

解决方案：

In fig: ED = building = 7m

AC = cable tower = ?

In rt ∆EDC,

$\frac{ED}{DC}$ = tan45°

7/x = 1/1

DC = 7

Now, EB = DC = 7m

In rt. ∆ABE,

$\frac{AB}{BC}$ = tan60°

AB/7 = √3/1

Height of tower = AC = AB + BC

7√3 + 7

= 7(√3 + 1)

= 7(1.732 + 1)

= 7(2.732)

Height of cable tower = 19.125m

问题13.从海拔75 m的一座灯塔的顶部观察，两艘船的俯角分别为30°和45°。如果一艘船正好位于灯塔同一侧的另一艘船后面，请找出两艘船之间的距离。

解决方案：

In fig:

AB = lighthouse = 75m

D and C are two ships

DC = ?

In rt. ∆ABD,

$\frac{AB}{BD}$ = tan30°

75/BD = 1/√3

BD = 75√3

In rt. ∆ABC

$\frac{AB}{BC}$ = tan45°

75/BC = 1/1

BC = 75

DC = BD – BC

= 75√3 – 75

75(√3 – 1)

75(1.372 – 1)

34.900

Hence, distance between two sheep is 34.900

问题14.一个身高1.2 m的女孩在离地面88.2 m的高度上发现了随风在水平线上移动的气球。距地面88.2 m的高度。在任何瞬间，气球从女孩眼中的仰角均为60°。一段时间后，仰角减小到30°(见图)。找出间隔内气球行驶的距离。

解决方案：

In fig: AB = AC – BC

= 88.2 – 1.2

= 81m

In rt. ∆ABE

$\frac{AB}{EB}$ = 87/EB = tan30°

87/EB = 1/√3

EB = 87√3

In rt. ∆FDE

$\frac{FD}{ED}$ = tan60°

√3 ED = 87  

ED = 87/√3

DB = DB – ED

87√3 – 87/√3

87(√3 – 1/√3)

= 87(3 – 1/√3)

= 87(2/√3) = 174/√3 * √3/√3

= 174 * √3/3 = 58√3

58 * 1.732 = 100.456m

Distance traveled by balloon is 100.456m

问题15：一条笔直的高速公路通向塔脚。站在塔顶的一个人以30°的俯角观察汽车，它以均匀的速度接近塔底。六秒钟后，发现汽车的下倾角为60°。找到从这一点到汽车到达塔底所需的时间。

解决方案：

In fig: AB is tower

In rt. ∆ABD  

$\frac{AB}{DB}$ = tan30°

$\frac{AB}{DB}$ = 1/√3

DB = √3 AB          -(1)

In rt. ∆ABC  

$\frac{AB}{BC}$ = tan60°

BC = AB/√3          -(2)

DC = DB – BC

= √3 AB – AB/√3  

AB(3 – 1/√3)

CD = 2AB/√3

$Speed = \frac{Distance}{Time}$

S1 = S2

\frac{D1}{T1} = \frac{D2}{T2}

$\frac{DC}{6} = \frac{CB}{t}$

2/√3AB/6 = AB/√3/t

2t = 6

t = 6/2

t = 3sec

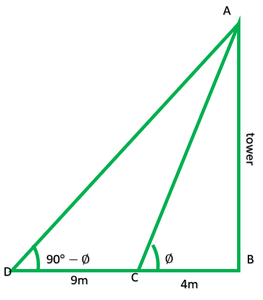
问题16.从距塔架底部4 m和9 m处且与塔架在同一直线上的两个点开始的塔顶顶部仰角是互补的。证明塔的高度为6 m。

解决方案：

In fig: AB is tower  

To prove: AB = 6m

Given: BC = 4m          DB = 9m

In ∆ABC

$\frac{AB}{BC}$ = tanθ

AB/4 = tanθ         -(1)

In ∆ABD

$\frac{AB}{BD}$ = tan (90°-θ)

AB/9 = 1/ tanθ

9/AB = tanθ         -(2)

From (1) and (2)

AB/4 = 9/AB

AB² = 36

AB = √36

AB = √(6 * 6)  

AB = 6m

Height of the tower is 6m.