10类RD Sharma解决方案–第4章三角形–练习4.5 |套装1

问题1.在图中，ΔACB〜ΔAPQ。如果BC = 8厘米，PQ = 4厘米，BA = 6.5厘米，AP = 2.8厘米，请找到CA和AQ。

解决方案：

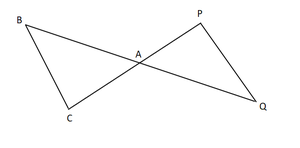
Given,

ΔACB ∼ ΔAPQ

BC = 8 cm, PQ = 4 cm, BA = 6.5 cm and AP = 2.8 cm

 To find: CA and AQ

We know that,

ΔACB ∼ ΔAPQ             [given]

$\therefore$BA/ AQ = CA/ AP = BC/ PQ       [sides are proportional of Similar Triangles]

Now,

6.5/ AQ = 8/ 4

AQ = (6.5 x 4)/ 8

AQ = 3.25 cm

Similarly, 

CA/ AP = BC/ PQ

CA/ 2.8 = 8/ 4

CA = 2.8 × 2

CA = 5.6 cm

Hence, CA = 5.6 cm and AQ = 3.25 cm.

问题2.在图AB AB QR中，找到PB的长度。

解决方案：

Given,

ΔPQR, AB ∥ QR

AB = 3 cm, QR = 9 cm and PR = 6 cm

 To find: length of PB

In ΔPAB and ΔPQR

We have,

∠P = ∠P            [Common angle ]

∠PAB = ∠PQR       [Corresponding angles]

∠PBA = ∠PRQ        [Corresponding angles]

$\therefore$ ΔPAB ∼ ΔPQR          [By AAA similarity criteria]

$\therefore$AB/ QR = PB/ PR     [sides are proportional of Similar Triangles]

⇒ 3/ 9 = PB/6

PB = 6/3

Hence the length of PB = 2 cm

问题3。给出XY∥BC。求出XY的长度。

解决方案：

Given,

XY∥BC

AX = 1 cm, XB = 3 cm and BC = 6 cm

To find: length of XY

In ΔAXY and ΔABC

We have,

∠A = ∠A             [Common angle]

∠AXY = ∠ABC    [Corresponding angles]

∠AYX = ∠ACB  [Corresponding angles]

$\therefore$ ΔAXY ∼ ΔABC    [By AAA similarity criteria]

$\therefore$XY/ BC = AX/ AB   [Corresponding Parts of Similar Triangles are propositional]

Now,

(AB = AX + XB = 1 + 3 = 4)

XY/6 = 1/4

XY/1 = 6/4

Hence, length of XY = 1.5 cm

问题4.在一个具有边a和b和斜边c的直角三角形中，在斜边上绘制的高度为x。证明ab = cx。

解决方案：

Let us consider  ΔABC to be a right angle triangle with sides a,b and c as  hypotenuse. let BD be the altitude drawn on the hypotenuse AC.

To prove: ab = cx

Now,

In ΔABC and ΔADB

∠BAC = ∠DAB    [Common]

∠ACB = ∠ABC = 90$\degree$    [right angled triangle]

$\therefore$ ΔABC ∼ ΔADB              [By AA similarity criteria]

So,

AC/ AB = BC/ EB      [Corresponding Parts of Similar Triangles are propositional]

c/ a = b/ x

⇒ xc = ab

$\therefore$ ab = cx

Hence, proved.

问题5。在图中，∠ABC= 90°，而BD⊥AC。如果BD = 8厘米，AD = 4厘米，找到CD。

解决方案：

Given,

ABC is a right-angled triangle and BD⊥AC.

 BD = 8 cm, and AD = 4 cm

To find: CD.

Now in triangle ΔABD and ΔCBD,

∠BDC=∠BDA        [each 90$\degree$]

∠ABD=∠CBD      [BD⊥AC]

$\therefore$ΔDBA∼ΔDCB  [By AA similarity]

BD/ CD = AD/ BD

BD² = AD x DC

(8)² = 4 x DC

DC = 64/4 = 16 cm

Hence, the length of side CD = 16 cm

问题6.在图中，∠ABC= 90 ^o ，BD⊥AC。如果AB = 5.7厘米，BD = 3.8厘米，CD = 5.4厘米，求BC。

解决方案：

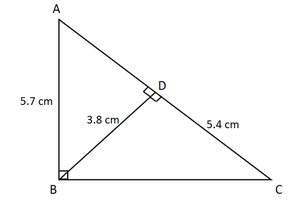
Given:

BD ⊥ AC

AB = 5.7 cm, BD = 3.8 cm and CD = 5.4 cm

∠ABC = 90^o

Required to find: BC

let ∠BCD=x

Now In ΔADB and ΔCDB,

∠ADB = ∠CDB = 90^o [right angled triangle] 

∠ABD = ∠CBD       [∠ABD = ∠CBD = 90^o – x]

$\therefore$ ΔABC ∼ ΔBDC [By AA similarity]

so,

AB/ BD = BC/ CD     [Corresponding Parts of Similar Triangles are propositional]

$\therefore$5.7/ 3.8 = BC/ 5.4

BC = (5.7 × 5.4)/ 3.8 = 8.1

Hence, length of side BC = 8.1 cm

问题7。给定DE∥BC，使AE =(1/4)AC。如果AB = 6厘米，找到AD。

解决方案：

Given:

DE∥BC

AE = (1/4)AC

AB = 6 cm.

To find: AD.

Now In ΔADE and ΔABC,

∠A = ∠A        [Common angle]

∠ADE = ∠ABC    [Corresponding angles]

$\therefore$ΔADE ∼ ΔABC   [By AA similarity criteria]

so,

$\therefore$AD/AB = AE/ AC   [Corresponding Parts of Similar Triangles are propositional]

AD/6 = 1/4

4 x AD = 6

AD = 6/4

$\therefore$AD=1.5 cm

Hence length of AD = 1.5 cm

问题8。给定，如果AB AB BC，DC⊥BC和DE⊥AC，则证明ΔCED〜ΔABC

解决方案：

Given:

AB ⊥ BC,

DC ⊥ BC,

DE ⊥ AC

To prove: ΔCED∼ΔABC

Now In ΔABC and ΔCED,

∠B = ∠E = 90^o   [given]

∠BAC = ∠ECD     [alternate angles]

$\therefore$ ΔCED∼ΔABC  [AA similarity]

Hence Proved

问题9.梯形ABCD与AB Di DC的对角AC和BD在O点处相交。使用两个三角形的相似性准则，得出OA / OC = OB / OD

解决方案：

Given: OC is the point of intersection of AC and BD in the trapezium ABCD, with AB ∥ DC.

To prove: OA/ OC = OB/ OD

Now In ΔAOB and ΔCOD,

∠AOB = ∠COD [Vertically Opposite Angles]

∠OAB = ∠OCD    [Alternate angles]

$\therefore$ ΔAOB ∼ ΔCOD

so,

 $\therefore$OA/ OC = OB/ OD   [Corresponding sides are proportional]

Hence Proved

问题10.如果ΔABC和ΔAMP是两个直角三角形，分别与B和M成直角，则∠MAP=∠BAC。证明

(i)ΔABC〜ΔAMP

(ii)CA / PA = BC / MP

解决方案：

(i)  Given:

Δ ABC and Δ AMP are the two right triangles.

Now In ΔABC and ΔAMP,

∠AMP = ∠B = 90^o

∠MAP = ∠BAC   [Vertically Opposite Angles]

$\therefore$ ΔABC∼ΔAMP     [AA similarity]

(ii) Since, ΔABC∼ΔAMP

CA/ PA = BC/ MP  [Corresponding sides are proportional]

Hence, proved.

问题11. 10厘米长的垂直棍棒会投射8厘米长的阴影。同时，一座塔投下了30 m长的阴影。确定塔的高度。

解决方案：

Given:

Length of stick = 10cm

Length of the stick’s shadow = 8cm

Length of the tower’s shadow = 30m = 3000cm

To find: the height of the tower = PQ.

Now In ΔABC and ΔPQR,

∠ABC = ∠PQR = 90^o  [each 90^o]

∠ACB = ∠PRQ   [angle are made at the same time]

$\therefore$ ΔABC ∼ ΔPQR    [By AA similarity]

So, 

AB/BC = PQ/QR    [by Corresponding sides are proportional]

10/8 = PQ/ 3000

PQ = (3000×10)/ 8

PQ = 30000/8

PQ = 3750/100

Hence the length of PQ = 37.5 m

问题12。在图中，∠A=∠CED，证明ΔCAB〜ΔCED。另外，找到x的值。

解决方案：

Given:

∠A = ∠CED

To prove: ΔCAB ∼ ΔCED

In ΔCAB and ΔCED

∠C = ∠C  [Common]

∠A = ∠CED [Given]

$\therefore$ΔCAB ∼ ΔCED [By AA similarity]

so,

CA/ CE = AB/ ED  [by Corresponding sides are proportional]

15/10 = 9/x

x = (9 × 10)/ 15

Hence, value for x = 6 cm