第10类RD Sharma解决方案–第7章统计–练习7.4 |套装1

问题1.以下是飞机发动机15个零件的小时寿命。求中位数：

715、724、725、710、729、745、694、699、696、712、734、728、716、705、719。

解决方案：

On arranging the observations in ascending order, we have

694, 696, 699, 705, 710, 712, 715, 716, 719, 721, 725, 728, 729, 734, 745

Number of terms in the observation sequence is odd, i.e., N = 15

Now,

Median = (N + 1)/2^th term

= (15 + 1)/2^th term

= 8^th term

Therefore, 716, which is the 8^th term is the median of the data.

问题2：以下是某城市某班学生的身高分布：

Height (in cm):	160 – 162	163 – 165	166 – 168	169 – 171	172 – 174
No of students:	15	118	142	127	18

找到中值高度。

解决方案：

We have N = 420,

So, N/2 = 420/ 2 = 210

Now, The cumulative frequency just greater than N/2 is 275 

Therefore, 165.5 – 168.5 is the median class s.t

L = 165.5, f = 142, F = 133 and h = (168.5 – 165.5) = 3

$Median = L + \frac{\frac{N}{2} - F}{f} * h \\ = 165.5 + \frac{210 - 133}{142} * 3 \\ = 165.5 + \frac{231}{142}$

= 165.5 + 1.63

= 167.13

问题3.以下是100名学生的智商分布。找到中位数智商

Class interval (exclusive)	Class interval  (inclusive)	Class interval frequency	Cumulative frequency
160 – 162	159.5 – 162.5	15	15
163 – 165	162.5 – 165.5	118	133(F)
166 – 168	165.5 – 168.5	142(f)	275
169 – 171	168.5 – 171.5	127	402
172 – 174	171.5 – 174.5	18	420
		N =420

解决方案：

N = 100,

Therefore, N/2 = 100/ 2 = 50

The cumulative frequency just greater than N/ 2 is 67 then the median class is (94.5 – 104.5) s.t,

L = 94.5, F = 33, h = (104.5 – 94.5) = 10

$Median = L + \frac{\frac{N}{2} - F}{f} * h \\ = 94.5 + \frac{50 - 34}{33} * 10$

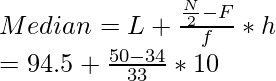
= 94.5 + 4.85

= 99.35

问题4.从以下数据计算中位数：

I.Q:	55 – 64	65 – 74	75 – 84	85 – 94	95 – 104	105 – 114	115 – 124	125 – 134	135 – 144
No of students:	1	2	9	22	33	22	8	2	1

解决方案：

N = 140,

And, N/2 = 140/ 2 = 70

The cumulative frequency just greater than N/ 2 is 98 then median class is 55 – 65 s.t,

L = 55, f = 40, F = 58, h = 65 – 55 = 10

$Median = L + \frac{\frac{N}{2} - F}{f} * h \\ = 55 + \frac{70 - 58}{40} * 10 \\ = 58$

问题5.从以下数据计算中位数：

Class interval (exclusive)	Class interval  (inclusive)	Class interval frequency	Cumulative frequency
55 – 64	54.5 – 64.5	1	1
65 – 74	64.5 – 74.5	2	3
75 – 84	74.5 – 84.5	9	12
85 – 94	84.5 – 94.5	22	34(F)
95 – 104	94.5 – 104.5	33(f)	67
105 – 114	104.5 – 114.5	22	89
115 – 124	114.5 – 124.5	8	97
125 – 134	124.5 – 134.5	2	98
135 – 144	134.5 – 144.5	1	100
		N = 100

解决方案：

N = 250,

And, N/2 = 250/ 2 = 125

The cumulative frequency just greater than N/ 2 is 127 then median class is 50 – 60 s.t,

 L = 50, f = 31, F = 96, h = 60 -50 = 10

$Median = L + \frac{\frac{N}{2} - F}{f} * h \\ = 50 + \frac{125 - 96}{31} * 10 \\ = 59.35$

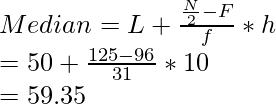
问题6.计算以下分布的丢失频率，假设分布的中位数为24。

Rent (in Rs):	15 – 25	25-35	35-45	45-55	55-65	65-75	75-85	85-95
No of houses:	8	10	15	25	40	20	15	7

解决方案：

Let us assume the unknown frequency to be x.

Class interval	Frequency	Cumulative frequency
0 – 10	5	5
10-20	25	30 (F)
20-30	x(f)	30 + x
30-40	18	48 + x
40-50	7	55 + x
	N=170

Given: Median = 24

Therefore,

Median class = 20 – 30; L = 20, h = 30 -20 = 10, f = x, F = 30

$Median = L + \frac{\frac{N}{2} - F}{f} * h \\24 = 20 + \frac{\frac{55+x}{2} - 30}{x} * 10 \\4 = \frac{\frac{55+x}{2} - 30}{x} * 10 \\ 4x = (\frac{55+x}{2} - 30) * 10$

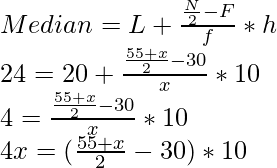
4x = 275 + 5x – 300

4x – 5x = – 25

– x = – 25

x = 25

Therefore, x = 25

问题7.下表列出了已婚妇女按结婚年龄划分的频率分布。

Class interval	Frequency	Cumulative frequency
15 – 25	8	8
25 – 35	10	18
35 – 45	15	33
45 – 55	25	58 (F)
55 – 65	40(f)	98
65 – 75	20	118
75 – 85	15	133
85 – 95	7	140
	N = 140

计算中位数并解释结果。

解决方案：

Class interval (exclusive)	Class interval (inclusive)	Frequency	Cumulative frequency
15 – 19	14.5 – 19.5	53	53(F)
20 – 24	19.5 – 24.5	140(f)	193
25 – 29	24.5 – 29.5	98	291
30 – 34	29.5 – 34.5	32	323
35 – 39	34.5 – 39.5	12	335
40 – 44	39.5 – 44.5	9	344
45 – 49	44.5 – 49.5	5	349
50 – 54	49.5 – 54.5	3	352
55 – 54	54.5 – 59.5	3	355
60 and above	59.5 and above	2	357
		N =357

N = 357,

And, N/2 = 357/ 2 = 178.5

The cumulative frequency just greater than N/2 is 193, 

Therefore, median class is 19.5 – 24.5 s.t

l = 19.5, f = 140, F = 53, h = 25.5 – 19.5 = 5

$Median = L + \frac{\frac{N}{2} - F}{f} * h \\ = 19.5 + \frac{178.5 - 53}{140} * 5$

Median = 23.98, that implies that nearly half of the women are married between the ages of 15 and 25.

问题8.下表列出了400盏霓虹灯的使用寿命：

Marks below:	0-10	10 – 20	20 – 30	30-40	40-50	50-60	60-70	70-80
No of students:	15	35	60	84	96	127	198	250

找到中位数寿命。

解决方案：

Life time	Number of lamps fi	Cumulative frequency (cf)
1500 – 2000	14	14
2000 – 2500	56	70
2500 – 3000	60	130(F)
3000 – 3500	86	216
3500 – 4000	74	290
4000 – 4500	62	352
4500 – 5000	48	400
	N = 400

Now

N = 400 

And the cumulative frequency just greater than n/2 (= 200) is 216, which belongs to the class interval 3000 – 3500 

Median class = 3000 – 3500. Therefore,

(l)  = 3000 and,(f) of median class = 86, (cf) of class preceding median class = 130 and (h) = 500

We have,

$Median = l+ \frac{\frac{n}{2} - cf}{f} * h \\ = 3000 + \frac{200 - 130}{86} * 500$

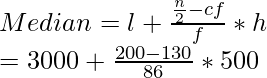
= 3000 + (35000/86)

= 3406.98 hrs, which is the median time of lamps. 

问题9.以下分布给出了每班30名学生的体重。查找学生的体重中位数：

Marks below	No. of students	Class interval	Frequency	Cumulative frequency
10	15	0-10	15	15
20	35	10-20	20	35
30	60	20-30	25	60
40	84	30-40	24	84
50	96	40-50	12	96(F)
60	127	50-60	31(f)	127
70	198	60-70	71	198
80	250	70-80	52	250
			N = 250

解决方案：

Weight (in kg)	Number of students fi	Cumulative frequency (cf)
40 – 45	2	2
45-50	3	5
50-55	8	13
55-60	6	19
60-65	6	25
65-70	3	28
70-75	2	30

The cf value just greater than n/ 2 (i.e. 30/ 2 = 15) is 19, belongs to class interval 55 – 60.

Therefore,

Median class = 55 – 60

where,

(l) of median class = 55, (f) of median class = 6, (cf) = 13 and (h) = 5

We have,

$Median = l+ \frac{\frac{n}{2} - cf}{f} * h \\ = 55 + \frac{15 - 13}{6} * 5$

= 55 + 10/6 = 56.666 which is approximately 56.67 kg.

问题10.如果平均值为1.46，则找到以下分布的缺失频率和中位数

Age in years:	0 – 10	10 – 20	20 – 30	30 – 40	40 – 50
No of persons:	5	25	?	18	7

解决方案：

No. of accidents (x)	No. of days (f)	fx
0	46	0
1	x	x
2	y	2y
3	25	75
4	10	40
5	5	25
	N = 200	Sum = x + 2y + 140

Since, we know,

 N = 200

Substituting values, we get, 

⇒ 46 + x + y + 25 + 10 + 5 = 200

⇒ x + y = 200 – 46 – 25 – 10 – 5

⇒ x + y = 114…… (i)

Also, Mean = 1.46

⇒ Sum/ N = 1.46

Substituting values, 

⇒ (x + 2y + 140)/ 200 = 1.46

⇒ x + 2y = 292 – 140

⇒ x + 2y = 152 …….(ii)

Solving from (i) and (ii), we get

x + 2y – x – y = 152 – 114

⇒ y = 38

And, x = 114 – 38 = 76 (from equation (i))

Now, putting the values, we get,

N = 200 N/2 = 200/2 = 100

So, the cumulative frequency just greater than N/2 is 122

And, therefore, the median is 1.