Class 11 RD Sharma解决方案–第20章几何级数-练习20.5 |套装2

问题12。如果(a – b)，(b – c)，(c – a)在GP中，则证明(a + b + c) ² = 3(ab + bc + ca)

解决方案：

Given: (a – b), (b – c), (c – a) are in G.P.

(b – c)² = (a – b)(c – a)

b² + c² – 2bc = ac – a² – bc + ab

b² + c² + a² = ac + bc + ab         -(1)

Now,

(a + b + c)² = a² + b² + c² + 2ab + 2bc + 2ca

= ac + bc + ab + 2ab + 2bc + 2ca

So, using eq(1), we get 

= 3ab + 3bc + 3ca

(a + b + c)² = 3(ab + bc + ca)

LHS = RHS

Hence, proved 

问题13：如果a，b，c在GP中，则证明：

$\frac{a^2+ab+b^2}{bc + ca+ab}=\frac{b+a}{c+b}$

解决方案：

Given: a, b, c are in G.P.

So, a, b = ar, c = ar²

$\frac{a^2+ab+b^2}{bc + ca+ab}=\frac{b+a}{c+b}\\ \frac{a^2+a(ar)+a^2r^2}{(ar)(ar^2)+(ar^2)a+a(ar)}=\frac{ar+a}{ar^2+ar}\\ \frac{a^2(1+r+r^2)}{a^2(r^3+r^2+r)}=\frac{a(1+r)}{a(r^2+r)}\\ \frac{1+r+r^2}{r(1+r+r^2)}=\frac{1+r}{r(1+r)}$

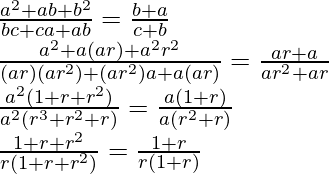
1/r = 1/r 

L.H.S = R.H.S

Hence, proved $\frac{a^2+ab+b^2}{bc + ca+ab}=\frac{b+a}{c+b}$

问题14.如果^{GP的第4，第}10和^第16项分别是x，y和z。证明x，y，z在GP中

解决方案：

Let us considered the 4^th term = ar³

10^th term = ar⁹

16^th term = ar¹⁵

So, ar⁹ = $\sqrt{(ar^3)(ar^{15})}$ = ar⁹

Therefore, 4^th, 10^th, 16^th terms are also in G.P.

Hence, proved

问题15.如果a，b，c在AP中，而a，b，d在GP中，则证明a，a – b，d – c在GP中

解决方案：

Given: a, b, c are in A.P.

2b = a + c          -(1)

also,

a, b, d are in G.P., so

b² = ad         -(2)

Now, 

(a – b)² = a² + b² – 2ab

= a² + ad – a(a + c)

From eq(1) and (2), we get

= a² + ad – a² – ac

= ad – ac

(a – b)² = a(d – c)

(a – b)/a = (d – c)/(a – b)

Hence, proved a, (a – b), (d – c) are in G.P.

问题16：如果^{AP的p th} ，q ^th ，r ^th和s ^th项在GP中，则证明p – q，q – r，r – s在GP中

解决方案：

 Let us considered R be common ratio,

Given: a_p, a_q, a_r, a_s of AP are in GP

R = $\frac{a_q}{a_p}=\frac{a_r}{a_q}\\ =\frac{a_q-a_r}{a_p-a_q}\ \ \ -(Using \ ratio\ property)\\ =\frac{[a+(q-1)d]-[a+(r-1)d]}{[a+(p-1)d]-[a+(q-1)d]}\\ =\frac{(q-r)d}{(p-q)d}\\ R=\frac{q-r}{p-q}\ \ \ \ -(1)$

Now,

$R=\frac{a_r}{a_q}=\frac{a_s}{a_r}\\ =\frac{a_r-a_s}{a_q-a_r}\ \ \ \ -(Using \ ratio\ property)\\ =\frac{[a+(r-1)d]-[a+(s-1)d]}{[a+(q-1)d]-[a+(r-1)d]}\\ =\frac{(r-s)d}{(q-r)d}\\ R=\frac{r-s}{q-r}\ \ \ \ \ -(2)$

Using eq(1) and (2), we get

$\frac{q-r}{p-q}=\frac{r-s}{p-r}\\$ 

Hence, proved (p – q), (q – r), (r – s) are in G.P.

问题17。 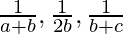$\frac{1}{a+b},\frac{1}{2b},\frac{1}{b+c}$是AP的三个连续项，请证明a，b，c是GP的三个连续项

解决方案：

Given: \frac{1}{a+b},\frac{1}{2b},\frac{1}{b+c}   are in A.P.

$\frac{2}{2b}=\frac{1}{(a+b)}+\frac{1}{(b+c)}\\ \frac{1}{b}=\frac{b+c+a+b}{(a+b)(b+c)}\\ \frac{1}{b}=\frac{2b+c+a}{ab+ac+b^2+bc}$

ab + ac + b² + bc = 2b² + bc + ba

b² + ac = 2b²

b² = ac

Hence, proved a, b , c are in G.P.

问题18.如果x ^a = x ^{b / 2} z ^{b / 2} = z ^c ，则证明1 / a，1 / b，1 / c在AP中

解决方案：

$x^2=x^{\frac{b}{2}}z^{\frac{b}{2}}=z^c=\lambda(say)\\ x=\lambda^{\frac{1}{a}},\ z=\lambda^{\frac{1}{c}}\\ \lambda^{\frac{1}{a}\left(\frac{b}{2}\right)}\times\lambda^{\frac{b}{2}\times\frac{1}{c}}=\lambda\\ \lambda^{\frac{b}{2a}+\frac{b}{2c}}=\lambda^1$

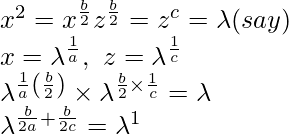
b/2a + b/2c = 1

1/a + 1/c = 2/b 

Hence, 1/a, 1/b, 1/c are in A.P.

问题19.如果a，b，c在AP中，b，c，d在GP中并且1 / c，1 / d，1 / e在AP中，请证明a，c，e在GP中

解决方案：

Given: a, b, c are in A.P.   

2b = a + c         -(1)

Also, b, c, d are in G.P.   

c² = bd         -(2)

1/c, 1/d, 1/e are in A.P.      

2/d = 1/c + 1/e         -(3)

We need to prove that

a, b, c are in G.P.

c² = ae

Now,

$c^2=bd=2b\times\frac{d}{2}\\ ⇒ c^2=(a+c)\times\frac{ce}{c+e}\\ ⇒ c^2=\frac{(a+c)ce}{c+e}\ \ \ \ \ \left[\because\frac{2}{d}=\frac{e+c}{ce}\right]$

c²(c + e) = ace + c²e

c³ + c²e = ace + c²e

c³ = ace

c² = ae

Hence, proved.

问题20.如果a，b，c在AP中，而a，x，b和b，y，c在GP中，则表明x ² ，b ² ，y ²在AP中

解决方案：

Given: a, b, c are in A.P.

2b = a + c         -(1)

Also, a, x, b are in G.P.

x = ab         -(2)

and b, y, c are in G.P.

y² = bc         -(3)

Now

2b² = x² + y²

= (ab) + (bc)              -(By using eq(2) and (3))

2b² = b(a + c)

2b² = b(2b)                  -(By using eq(1))

2b² = 2b²

L.H.S = R.H.S

2b² = x² + y²

Hence,  x², b², y² are in A.P.

问题21.如果a，b，c在AP中，而a，b，d在GP中，则表明a，(a – b)，(d – c)在GP中

解决方案：

Given: a, b, c are in A.P.

2b = a + c         -(1)

Also, a, b, d are in G.P.

b² = ad         -(2)

Now

(a – b)² = a(d – c)        -(By using eq(2))

a² – 2ab = -ac

a² – 2ab = ab – ac

a(a – b) = a(b – c)

a – b = a – c

2b = a + c

a + c = a + c,            -(By using eq(1))

L.H.S = R.H.S

Hence, a, (a – b), (d – c) are in G.P.

问题22。如果a，b，c是GP中的三个实数，并且a + b + c = xb，则证明x <-1或x> 3。

解决方案：

Let us considered r be the common ratio of G.P.

So, a, b = ar, c = ar²

a + b + c = xb

a + ar + ar² = x(ar)

a(1 + r + r²) = x(ar)

r² + (1 – x)r + 1 = 0

Here, r is real, so

D ≥ 0

(1 – x)² – 4(1)(1) ≥ 0

1 + x² -2x – 4 ≥ 0

x² – 2x – 3 ≥ 0

(x – 3)(x + 1) ≥ 0

Hence, x < -1 or x > 3

问题23如果^第p^个，^第q和r一个AP和GP的^第方面均为分别b和c，示出了一个^BC b ^CA c ^ ^AB = 1。

解决方案：

Let us considered the A.P. be A, A + D, A + 2D, …. and G.P. be x, xR, xR², 

Then

a = A + (p – 1)D, B = A + (q – 1)D, c = A + (r – 1)D

a – b = (p – q)D, b – c = (q – r)D, c – a = (r – p)D

Also a = XR^p-1, b = xR^q-1, c = xR^r-1

Hence, a^b-c.b^c-a.c^a-b = (xR^p-1)^(q-r)D.(xR^q-1)^(r-p)D.(xR^r-1)^(p-q)D

= x^{(q-r+r-p+p-q)D}.R^{[(p-1)(q-r)+(q-1)(r-p)+(r-1)(p-q)]D}

= x⁰.R⁰ 

= 1.1 

= 1