问题1.以下哪个陈述适用于菱形?
(i)它有两对平行的边。
(ii)它有两对相等的边。
(iii)它只有两对相等的边。
(iv)它的两个角度成直角。
(v)它的对角线彼此成直角一分为二。
(vi)对角线相等且垂直。
(vii)它的所有边都等长。
(viii)它是一个平行四边形。
(ix)它是一个四边形。
(x)可以是正方形。
(xi)它是一个正方形。
解决方案:
(i) True
(ii) True
(iii) False. A rhombus has all four sides equal.
(iv) False. A rhombus has no right angle.
(v) True
(vi) False. Rhombus diagonals are unequal in length.
(vii) True
(viii) True
(ix) True
(x) True. Rhombus becomes square when any one angle is 90°.
(xi) False. Rhombus is never a square. Since in a square each angle is 90°.
问题2.在下面的每个栏中填写空格,以使陈述正确:
(i)菱形是_______的平行四边形。
(ii)正方形是_________的菱形。
(iii)菱形的各边长度为______。
(iv)菱形的对角线______彼此成______角。
(v)如果平行四边形的对角线彼此成直角一分为二,则它是______。
解决方案:
(i) adjacent sides are equal.
(ii) any one angle is right angle.
(iii) equal
(iv) bisect, right
(v) rhombus
问题3.平行四边形的对角线不垂直。是菱形吗?为什么或者为什么不?
解决方案:
No, Diagonals of a rhombus bisect each other at 90°, where the diagonals must be perpendicular to each other.
问题4.四边形的对角线彼此垂直。这样的四边形总是菱形吗?如果您的答案为“否”,请画一个数字以证明您的答案合理。
解决方案:
No, since the diagonals of a rhombus are perpendicular to each other and bisect. Also, its sides are equal.
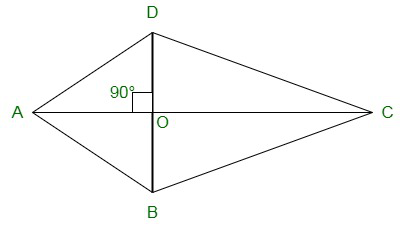
问题5. ABCD是菱形。如果∠ACB= 40°,则找到∠ADB。
解决方案:
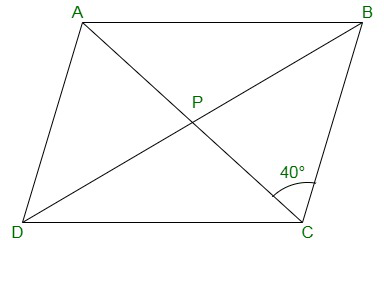
ABCD is a rhombus where the diagonals are perpendicular to each other.
So, ∠BOC = 90o
From ΔBOC, the sum of angles in a triangle is 180o
Therefore,
∠CBO + ∠BOC + ∠OBC = 180o
Solving, we get,
= 180o – 40o – 90o
= 50o
Since, the alternate angles are equal,
∴ ∠ADB = ∠CBO = 50o
问题6.如果菱形的对角线分别为12厘米和16厘米,则求出每边的长度。
解决方案:

In a rhombus, diagonals bisect each other at right angle.
Now, In ΔAOB
AO = 12/2 = 6cm, BO = 16/2 = 8cm
AB2 = AO2 + BO2 (Pythagoras Theorem)
AB2 = 62 + 82
AB2 = 36 + 64
AB2 = 100
AB =√100 = 10cm
Therefore, each side of a rhombus is 10cm.
问题7.构造一个菱形,其对角线长度分别为10 cm和6 cm。
解决方案:
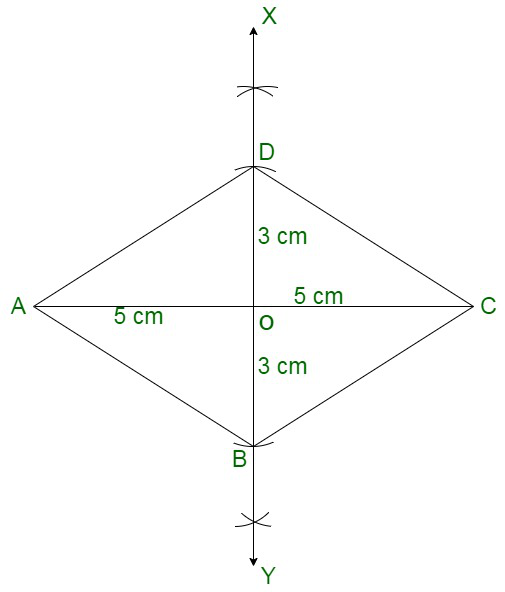
Steps to Construct a rhombus:
(i) Draw a diagonal AC of length 10 cm.
(ii) Taking O as centre, draw a perpendicular bisector of AC to point O.
(iii) From point ‘O’ cut two arcs of length 3cm each to get points OB and OD.
(iv) Join the line segments AD and AB, BC and CD to get rhombus ABCD.
问题8.画一个菱形,每边长3.5厘米,其中一个角度为40°。
解决方案:

Steps to construct a rhombus:
(i) Draw a line segment AB of length 3.5 cm.
(ii) From point A and B construct two angles of 40 and 140 degrees respectively.
(iii) From points A and B cut two arcs of length 3.5 cm each to get the points D and C.
(iv) Join the line segments AD, BC, CD to get rhombus ABCD.