第 9 类 RD Sharma 解决方案 - 第 17 章构造 - 练习 17.2
问题 1. 画一个角并将其标记为∠BAC。构造另一个角,等于∠BAC
解决方案:
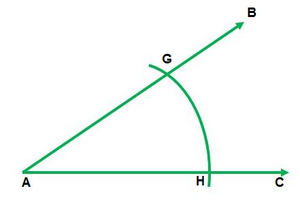

Steps of construction:
1. Draw any ∠BAC and a line segment YZ .
2. Now, from center A draw an arc of any radius which intersects ∠BAC at points G and H.
3. Now, draw an arc of same radius with Y as a centre which intersects YZ at point M.
4. With M as center and radius equal to HG, draw an arc which intersects the previous arc at point L.
5. Draw a line segment joining points Y and L.
Hence ∠XYZ = ∠BAC
问题 2. 画一个钝角。把它一分为二。测量这样形成的每个角度。
解决方案:

Steps of construction:
1. Draw an angle ∠XYZ of 120°.
2. With Y as a centre and any radius, draw an arc which intersects XY at point U and YZ at point V.
3. With U as center and radius more than half of UV draw an arc.
4. With V as a center and same radius draw an arc which cuts the previous arc at point W.
5. Join YW.
Hence ∠XYW = ∠WYZ = 60°
问题 3. 用量角器画一个 108° 的角。使用给定的给定角度,绘制一个 54° 的角度。
解决方案:

Steps of construction:
1. Draw an ∠XYZ of 108°.
2. With Y as the center and any radius draw an arc which intersects XY at point U and YZ at point V.
3. With U as center and radius more than half of UV draw an arc.
4. With V as the centre and same radius draw an arc which intersects the previous arc at point W.
5. Join YW.
Hence, ∠WYZ = 54°
问题 4. 用量角器画一个直角。平分它以获得 45° 的测量角度。
解决方案:

Steps of construction:
1. Draw an ∠XYZ of 90°.
2. With Y as the centre and any radius draw an arc which intersects XY at point U and YZ at point V.
3. With U as center and radius more than half of UV draw an arc.
4. With V as center and same radius draw an arc which intersects the previous arc at point W.
5. Join YW.
Hence, ∠WYZ = 45°
问题 5. 画出一对线性角。平分两个角中的每一个。验证两条平分光线是否相互垂直。
解决方案:

Steps of construction:
1. Draw two ∠OYX and ∠OYZ forming linear pair
2. With center Y and any radius draw an arc which intersects XY at E and YO at point F and YZ at point G
3. With center E and F and any radius draw two arcs which intersect each other at point H
4. Join HY
5. With F and G as center and any radius draw two arcs which intersect each other at point W
6. Join WY
Hence, ∠HYW = 90°.
问题 6. 画一对垂直对角。平分两个角中的每一个。验证二等分光线是否在同一条线上。
解决方案:
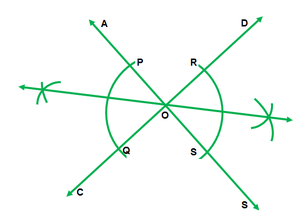
Steps of Construction:
1. Draw a pair of vertically opposite angle ∠AOC and ∠DOB.
2. With O as the center and any radius draw two arcs which intersect OA at point P, OC at point Q, OB at point S and OD at point R.
3. With P and Q as center and radius more than half of PQ draw two arcs which intersect each other at point T.
4. Join TO.
5. With R and S as center and radius more than half of RS draw two arcs which intersect each other at point U.
6. Join OU.
Hence,TOU is a straight line
问题 7. 只用尺子和圆规画一个直角。
解决方案:

Steps of construction:
1. Draw a line segment XY.
2. With X as the center and any radius draw an arc which intersects XY at point M.
3. With M as center and the same radius draw an arc which intersects the previous arc at point N.
4. With N as the center and the same radius draw an arc that intersects arc in (2) at point O.
5. With O and N as center and radius, more than half of ON draw arcs that intersect each other at point Z.
6. Join ZX.
Hence, ∠ZXM = 90°
问题 8. 仅使用尺子和圆规,画出 135° 的测量角。
解决方案:
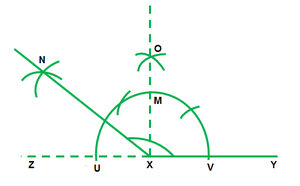
Steps of construction:
1. Draw a line segment XY and produce YX to Z.
2. With X as the center and any radius draw an arc which intersects XZ at point U and XY at point V.
3. With U and V as center and radius more than half of UV draw arcs which intersect each other at point O.
4. Join OX which intersects the arc in step(2) at point M.
5. Keeping M and U as center and radius more than half of MU draw arcs which intersect each other at point N.
6. Join NX.
With ∠NXY = 135°
问题 9. 用量角器画一个 72° 的角度。以这个角度为给定的绘制角度为 36° 和 54°。
解决方案:
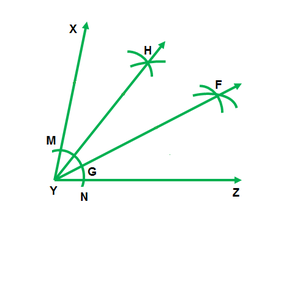
Steps of construction:
1. Draw an ∠XYZ of 720 with the help of a protractor.
2. With Y as center and any radius draw an arc which intersects XY at point M and YZ at point N.
3. With M and N as center and radius more than half of MN draw two arcs which intersect each other at point F.
4. Join FY which intersects the arc in step(2) at point G.
5. With M and G as center and radius more than half of MG draw two arcs which intersect each other at point H.
6. Join HY
Hence, ∠HYX = 54° ∠FYZ = 36°
问题 10. 在给定射线的初始点构造以下角度并证明构造的合理性:
(一) 45°
(ii) 90°
解决方案:

(i) Steps of construction:
1. Draw a line segment XY and produce YX to point Z.
2. With X as the center and any radius draw an arc which intersects XZ at point M and XY at point N.
3. With M and N as center and radius more than half of MN draw arcs which intersect each other at point F.
4. Join FX which intersects the arc in step(2) at point O.
5. With O and N as center and radius more than half of ON draw arcs which intersect each other at point H.
6. Join HX.
Hence, ∠HXY = 45°

(ii) Steps of construction
1. Draw a line segment XY.
2. With X as the center and any radius draw an arc which intersects XY at point Z.
3. With Z as center and the same radius draw an arc which intersects the previous arc at point O.
4. With O as the center and same radius draw an arc which intersects arc in (2) at point G.
5. With G and O as center and radius more than half of GO draw arcs which intersect each other at point H.
6. Join HX.
Hence, ∠HXY = 90°
问题 11. 构造以下测量的角度:
(一) 30°
(ii) 75°
(iii) 105°
(iv) 135°
(五) 15°
(vi) 22(1/2)°
解决方案:

(i) Steps of construction:
1. Draw a line segment XY.
2. With X as the centre and any radius draw an arc which intersects XY at point Z.
3. With Z as center and the same radius draw an arc which intersects the previous arc at point D.
4. With D and Z as center and radius more than half of DZ draw arcs which intersect each other at point E.
5. Join EX.
Hence, ∠EXY = 30°
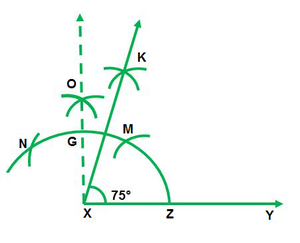
(ii) Steps of construction:
1. Draw a line segment XY.
2. With x as center and any radius draw an arc which intersects XY at point Z.
3. With Z as center and the same radius draw an arc which intersects the previous arc at point M.
4. With M as center and same radius draw an arc which intersects arc in step (2) at point N.
5. With N and M as center and radius more than half of NM, draw arcs intersecting each other at point O.
6. Join OX which intersects arc in step(2) at point G.
7. With G and M as center and radius more than half of GM draw arcs intersecting each other at point K.
8. Join KX.
Hence, ∠KXY = 75° .
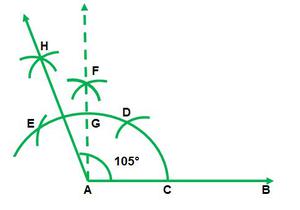
(iii) Steps of construction:
1. Draw a line segment AB.
2. With A as the center and any radius draw an arc which intersects AB at point C.
3. With C as center and the same radius draw an arc which intersects the previous arc at point D.
4. With D as the centre and same radius draw an arc which intersects arc in step (2) at point E.
5. With E and D as center and radius more than half of ED draw arcs which intersect each other at point F.
6. Join FA which intersects arc in step (2) at point G.
7. With E and G as center and radius more than half of EG draw arcs which intersect each other at point H.
8. Join HA.
Hence, ∠HAB = 105°

(iv) Steps of construction:
1. Draw a line segment AB and produce BA to point C.
2. With A as the center and any radius draw an arc that intersects AC at D and AB at point E.
3. With D and E as center and radius more than half of DE draw arcs that intersect each other at point F.
4. Join FA which intersects the arc in step(2) at point G.
5. With G and D as center and radius more than half of GD draw arcs that intersect each other at point H
6. Join HA.
Hence, ∠HAB = 135°

(v) Steps of construction:
1. Draw a line segment AB.
2. With A as the centre and any radius draw an arc that intersects AB at point C.
3. With C as center and the same radius draw an arc that intersects the previous arc at point D.
4. With D and C as center and radius more than half of DC draw arcs that intersect each other at point E.
5. Join EA which intersects arc in step(2) at point F.
6. With F and C as center and radius more than half of FC draw arcs that intersect each other at point G.
7. Join GA.
Hence, ∠GAB = 15°

(vi) Steps of construction:
1. Draw a line segment AB.
2. With A as the center and any radius draw an arc that intersects AB at point C.
3. With C as center and the same radius draw an arc that intersects the previous arc at point D.
4. With D as the centre and same radius draw an arc that intersects arc in step (2) at point E.
5. With E and D as center and radius more than half of ED draw arcs which intersect each at point F.
6. Join FA which intersects arc in step(2) at G.
7. With G and C as center and radius more than half of GC draw arcs intersecting each other at point H.
8. Join HA which intersects the arc in step(2) at a point I.
9. With I and C as center and radius more than half of IC draw arcs intersecting each other at point point J.
10. Join JA.
Hence, ∠JAB = 22(1/2)°